The variation of induced EMF $\varepsilon$ with time $t$ in a coil if a short bar magnet is moved along its axis with a constant velocity
tack.imgur.com/hWpO5.png
can someone kindly tell the mathematical proof? Not the theoretical one stating Lenz law
"As the magnet comes close, there will be emf induced, Later on, the magnet moves away, hence induced emf is present in opposite direction on compared to before."
This is the waveform for a free falling, relative short length magnet through a long solenoid (Actual experiment):
The negative semi-period is larger in amplitude than the positive depicted above due to the g acceleration.
For an almost constant speed, falling magnet the waveform looks like this bellow (Actual experiment):
Note: Due to constant speed the waveform is in this case symmetrical in amplitudes of positive and negative pulse.
In both cases the induced current is almost zeroed when total length of short magnet is inside the long solenoid. The flux change of the leading pole inside the solenoid is negated form the flux change by the trailing end pole of the magnet.
(see also this explanation https://physics.stackexchange.com/a/89296/183646).
In general for the pole of the magnet approaching one end of the solenoid at $vt$ distance from the solenoid opening (along the central axis of the long solenoid) and then leaving with its opposite pole the other end of the solenoid this formula holds:
$$I(t)=\frac{\mu_{0} q_{m}}{2 L}\left[1+\frac{v t}{\sqrt{(v t)^{2}+b^{2}}}\right]$$
with $b$ the inner radius of the solenoid, $t=0$ the time at which the dipole short magnet is totally inside the solenoid and the induced current is zeroed, $t<0$ the time in which the magnet is approaching the solenoid and $t>0$ the time in which it is leaving the other end of the solenoid. $L$ is the inductance of the solenoid, $q_{m}$ (in SI units of magnetic flux $Φ$, Wb) is the magnetic charge of the pole (using the magnetic pole model) and $\mu_{0}$ the magnetic permeability of vacuum space. Use $q_{m}$ with an opposite sign for the magnet leaving the solenoid.
Then use,
$$\varepsilon=-L \frac{d I}{d t}$$
to find the self-induced emf voltage value.
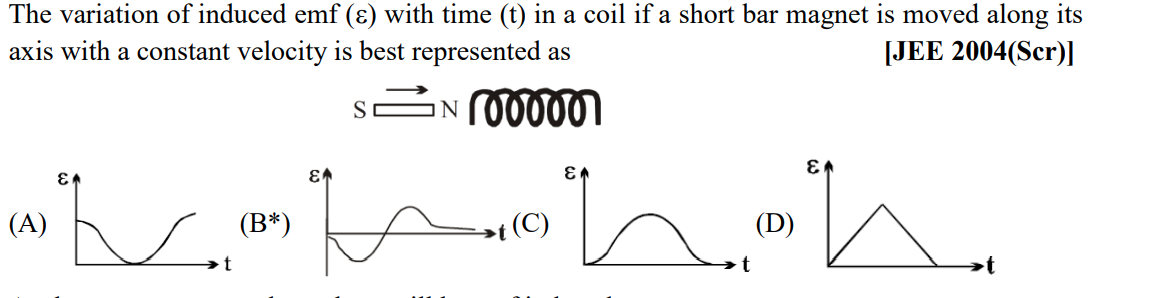
The coil in your diagram is a solenoid, that is a tube-shaped coil. I shall assume that it is longer than the magnet.
A full mathematical treatment would take proper account of the magnet's magnetic field pattern. This can be done, but the mathematics is a little tedious. I offer instead a simple, but I hope, instructive, treatment based on a huge over-simplification of the field pattern... Imagine that at the North Pole end of the magnet the field lines (total flux $\Phi$) sprout out at right angles to the magnet's geometrical axis, and then, well away from the magnet, the lines bend round and return to the South Pole end in the same way that they left the North end, and continue through the magnet to its North end. Each line is a closed loop – a rectangle in our model.
If the magnet is advanced at a steady speed, $v$, along the solenoid's axis, so that its North end enters the solenoid at time zero, then at time $t$ ($vt$ < length, $l_\text{mag}$, of magnet) the number of turns linked by the magnet's flux will be $\nu vt$, in which $\nu$ is the number of turns per unit length of solenoid. So we have $$\text{flux linkage}=\Phi\nu vt.$$ So $$\text{emf}=\frac d{dt}\Phi\nu vt=\Phi\nu v=\text{constant}.$$ When $vt=l_\text{mag}$ the magnet is fully inside the solenoid so, as the magnet advances, no further turns of the solenoid are linked with the magnet's flux, the flux linkage remaining constant at $l_\text{mag}\nu \Phi$, so the emf is zero. When the North Pole of the magnet emerges from the other end of the magnet, the flux linkage starts to fall at the same rate that it rose when the magnet entered, so there will be an equal and opposite emf until the whole magnet is outside the solenoid.
If we had used a more realistic version of the magnet's field, rather than modelling the field lines as rectangular loops, we would find a gradual rise in the emf as the magnet approached the solenoid, an increase to a peak and a gradual fall to almost zero when the magnet was well inside the solenoid. There would be a negative hump as the magnet emerges. So smooth humps replace the rectangular pulses of our simple model.
