Where do Cooper pairs fit in the superconducting density of states?

Question

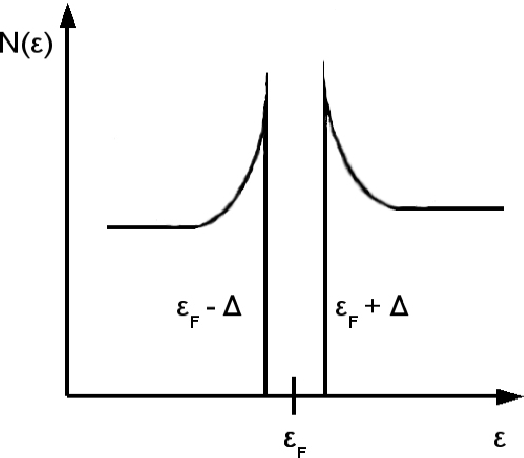
This is a very common diagram of the superconducting density of states. In a normal metal, the Fermi energy lies somewhere in the conduction band and there are unoccupied states above it in the same band.

As we transition to the superconducting phase, the conduction electrons form Cooper pairs. I have read in various sources that the pairs "condense" into the same ground state. At absolute zero there are no conduction electrons left as they have all become Cooper pairs. At the same time an energy gap appears in the density of states, with $\Delta$ being several orders of magnitude below the energy range of the conduction band (I think).

My question is as follows: Where do the Cooper pairs fit in this diagram? What state to they occupy?

Do they all "condense" into the lowest energy state of the conduction band, and as pairs are broken do the electrons just populate the states in between the bottom of the band and the energy gap? Some sources state (unless I have misunderstood them) that when a Cooper pair breaks the electrons are excited above the energy gap. But what about all those states in the conduction band below the gap? Is this density of states even applicable to Cooper pairs, or will their ground state not even appear on this diagram? I would appreciate some help understanding this.

Answer 1

The density of states shown is the single electron density of states. In other words, it shows the density of single electron states. Once the electrons condense into Cooper pairs, they cannot be described with single electron states, and thus "vanish" out of your single electron state description. Assuming zero temperature, all states to the left of $\epsilon_F$ are filled (valence band), all states to the right are empty (conduction band). Current is now carried by Cooper pairs, not single electrons.

To break a Cooper pair, you need to place each electron in the conduction band, which requires about $2 \Delta$ energy. These electrons were not in any other single electron states of the DoS before.

On the bands: At zero temperature, all states below $\epsilon_F$ are filled, and all states above are empty. In a metal, $\epsilon_F$ is in the conduction band, and moving $\epsilon_F$ slightly up or down changes how many electron states are filled. In an insulator, $\epsilon_F$ is in the gap between the bands, and moving $\epsilon_F$ slightly changes nothing. In terms of bands, it makes sense to consider the superconductor an insulator, since $\epsilon_F$ is in a gap, and moving it does not change anything for the electron states.

In a sense, the superconductor is an electron insulator, since no electrons can carry current, only Cooper pairs. By carving a gap through the conduction band, we have turned the metal into a kind of insulator, and the bottom part of the previous conductance band is now a filled valence band.

This is all in terms of electron states, not the electrons themselves. Trying to develop an intuition for which electrons go into the condensate is a futile task. All of them went into the condensate, and the DoS diagram no longer shows where the electrons are, but where they can go if we excite the superconductor out of its ground state.