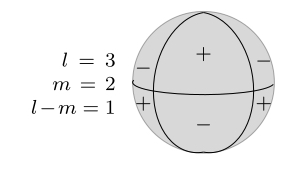
In our 3+1 dimensional world covalent bonds occur because electron pair are shared between atoms. How many electrons are needed to form a covalent bond in a n+1 dimensional world?
The reason this is not entirely obvious is that there are more spin quantum numbers in a high-dimensional world. In our world there is just one spin, and hence two electrons with opposite spins are allowed to have otherwise equal quantum numbers, spreading out between the two atoms. Hence we end up with two electrons involved in the bond.
In a $n$ dimensional space there are $\lfloor n/2 \rfloor$ separate angular momenta, and it seems reasonable (but more complex to analyze properly) that there would be more spin quantum numbers. So does that mean that we could have not just 2-electron covalent bonds, but k-electron pair bonds (up to $k=n/2$)? Or do we need $n$ electrons to make a covalent bond? Or even $2^{n/2}$ so each combination of spin quantum numbers is present?
(Ionic bonds appear much easier to model, although the rapid decay of electromagnetic attraction would make them weaker.)