0
$\begingroup$

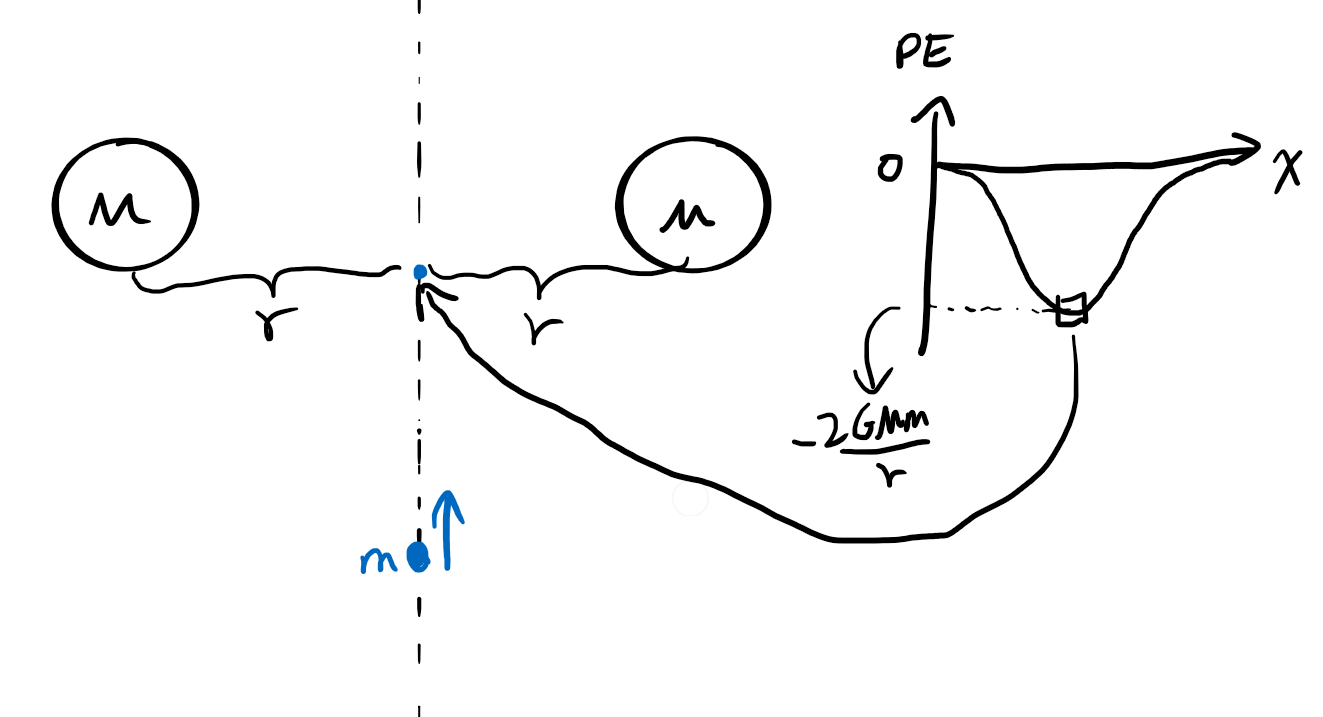
The situation is illustrated below: enter image description here

The blue dot is an object with mass $m$, moving at constant speed on the equidistant line between the two masses $M$. The graph on the right shows how the potential energy of object $m$ changes as it moves along the line — gradually decreasing until it reaches a minimum at the centre of mass of the masses $M$.

Now so far so good, the problem arises when you examine the energy of mass $m$, in that its potential energy decreases without changing its kinetic energy, as the work done by the masses $M$ cancel each other out, and this seems to be a violation of the law of conservation of energy.

What I've tried

At first I thought the fallacy must lie within the assumption that the masses $M$ are not moving, which they most definitely are. However, after some more thought I realised this does not matter, because as long as the movement of the two masses are symmetrical, they still do zero work on mass $m$ overall. Indeed all it does is complicating the potential energy vs distance graph, yet the potential energy still changes without changing the kinetic energy.

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ If he object is moving at constant speed along that line, then there must be a third force acting on it. Under the influence of just the gravitational force exerted by the other two masses, the object will speed up as it moves to the center-point and slow down as it moves away from the center point, consistent with the conservation of energy. The work done by the two masses do not cancel! They are both positive while the object is moving towards the center and both negative while the object is moving away from the center. $\endgroup$
    – march
    Commented Jan 27, 2022 at 5:20
  • $\begingroup$ By the way, if you find that the gravitational potential energy changes when the work done by the gravitational force is zero, then you're doing something wrong, because $\Delta P_{\textrm{grav}} = -W_{\textrm{by grav}}$, where you have to be careful about how you choose your system in order to interpret this statement. $\endgroup$
    – march
    Commented Jan 27, 2022 at 5:23
  • $\begingroup$ @march haha thank you so much I don't know how I missed that $\endgroup$
    – joshua mason
    Commented Jan 27, 2022 at 6:37

1 Answer 1

Reset to default
2
$\begingroup$

As stated in march's comments, your belief that the work done by the masses cancels is false. The change in potential energy is just the negative of the work it does $\Delta PE_{gravity} = -W_{gravity} $. At every point along the line (except the intersection with a line joining the two masses M) mass m is subject to a net force along its direction of motion. You can see this by vector addition of the forces from the two masses. This force does work $W = \int \mathbf{F}_{gravity} \cdot d \mathbf{s} = \Delta KE$ on m. The net force changes sign where potential energy has its minimum.

Energy will be conserved even if the two M masses are held static. In this case the forces acting on them will not act over any distance so $W = \int \mathbf{F} \cdot d \mathbf{s} = 0$. The situation where the bodies are free but $M \gg m$ will approximate this.

Improve this answer
$\endgroup$
1
  • $\begingroup$ I do NOT know how didn't notice this hahaha tysm. $\endgroup$
    – joshua mason
    Commented Jan 27, 2022 at 6:36

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.