2
$\begingroup$

Current and "amount of substance" are base units

The SI system treats both electric current and "amount of substance" as a quantity that is measured in "fundamental" or "base" units. These are amperes and moles, respectively.

Charge has been quantized for more than a century

However, since Millikan's oil drop experiment in 1909, physicists have known that charge is quantized. Perhaps this is still debatable in some forms of exotic string theory or something, but charge quantization is indisputably a reasonable approximation (at least!) for all situations for everyday mundane planetary conditions.

A missed simplification

The SI system already has the unit "mol" to measure "amount of substance". Why doesn't SI treat "fundmental charges" as a substance that is to be measured in moles? That is, why hasn't anyone tried to advocate that the SI base unit for current should be e.g. moles of charge per second?

Moving in this direction would seem to simplify things considerably. We wouldn't need volts; it's just Joules per mole of charge. And so on...

Conversely, if you don't like the unit "mol", you could argue that since charge is quantized, we don't need moles anymore and and can measure the "amount of substance" in Coulombs. A similar simplification would ensue.

So why haven't relevant standards organizations or committees for the SI system considered these types of simplification?

Improve this question
$\endgroup$
2

1 Answer 1

Reset to default
2
$\begingroup$

What you suggest is not really a simplification. Perhaps you confuse the SI base units and the SI derived units. The SI base units are a set of seven units from which all SI derived units can be derived (hence their name). The base units are defined by relating them to measurements of fundamental constants. The unit of Volt that you mention, is a derived unit which can be expressed in base units as kg m$^2$ A$^{-1}$ s$^{-3}$.

In addition, if you look at the SI base units and how they relate to the fundamental constants, you see that linking the mole to the Ampère, does not really simplify things. One still need a link to the electric charge of the electron and the hyperfine splitting of Cs.

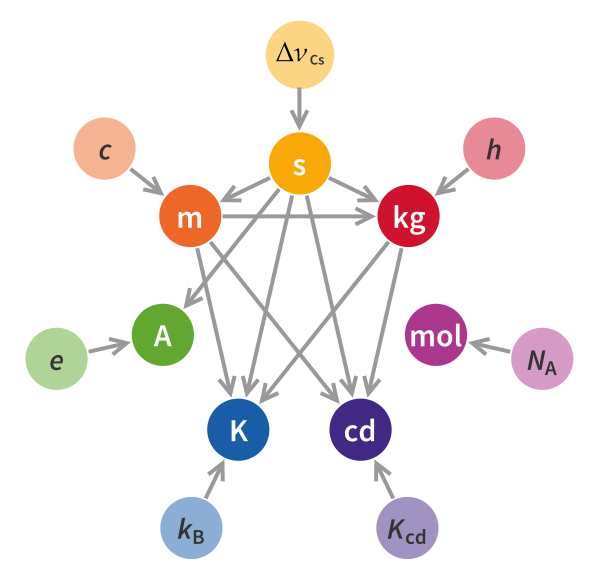enter image description here Image from Wikipedia.

Improve this answer
$\endgroup$
5
  • $\begingroup$ The charge on the electron would be "1" in my system. That's the simplification. Amperes could become a derived unit that refers to mols / second of charge. $\endgroup$
    – Curt F.
    Commented Jan 18, 2022 at 19:03
  • 1
    $\begingroup$ This would be similar to for example atomic units. Defining the electron charge as unity would loose connection with the physical world and you would need another constant to convert numbers into something you can compare with experiment (just as you have to do when working with atomic units). $\endgroup$
    – Paul
    Commented Jan 18, 2022 at 19:12
  • $\begingroup$ An analogy is maybe the distinction between inertial mass and gravitational mass. In SI, they are both measured in kilograms. However, charge and "amount of substance", are measured in different units, despite both effectively describing quantities of fundamental particles. If it makes sense to treat inertial mass and gravitational mass with the same "base unit", why doesn't it make sense to treat "amount of substance" and "charge" with the same unit? I don't understand how you would lose connection with the physical world... $\endgroup$
    – Curt F.
    Commented Jan 18, 2022 at 21:52
  • 1
    $\begingroup$ I do not really get your analogy, inertial mass and gravitational mass represent the same quantity, so why would their units be different? It is true that the mol is a somewhat superfluous unit that mostly serves the purpose of making quantities workable. You can see that directly from the figure, $N_\text{A}$ is not a fundamental constant of nature of course. There are several questions on this site asking about the purpose of the mol. $\endgroup$
    – Paul
    Commented Jan 19, 2022 at 5:08
  • $\begingroup$ Paul -- I appreciate your answer and have accepted it. The analogy I was making in more detail: imagine a world in which different units were used for inertial mass and gravitational mass, say "kilograms" and "florgles". Then, 100 years ago, someone did an experiment and determined a precise constant that interrelates kilograms and florgles, and theory supports that this relationship is exact. Wouldn't it make sense to discontinue the use of florgles and to use kilograms for everything? So it is, I think, with e and moles. $\endgroup$
    – Curt F.
    Commented Jan 21, 2022 at 21:06

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.