I am confused about this contradiction in special relativity?

Question

Consider a stationary object and an object moving at half the speed of light to the right and light moving to the right past them. To observe light moving at the same speed that the stationary object sees the light moving at, it has to experience half the time that the stationary object experiences and that is because when the stationary object sees the light moving a certain distance the moving object will see the light have moved half the distance, so it must see the light moving half the distance in half the time for the speed of light to be the same. But if you consider another light moving in the opposite direction, for the moving object to see that light moving at the same speed that the stationary object sees that light moving at, it must experience double the time that the stationary object experiences because the moving object will see the light moving double the distance this time. The problem here is that the moving object can not experience both half the time, and double the time that the stationary object experiences, which means that the moving object cannot see both lights moving at the same speed (the speed of light). But special relativity states that light speed is constant for all frames of reference. So how can a moving object see all lights moving in different directions all moving at the same speed of light which the stationary object sees these lights moving at?

Answer 1

You are missing the relativity of simultaneity. The Lorentz transform is more than just time dilation and length contraction. The relativity of simultaneity is also essential.

Not only is the time dilated and length contracted, but also the moving clocks are de synchronized so that leading clocks lag. This allows for the speed of light to be c in all directions in both frames.

The easiest way to demonstrate this is as follows:

The equation of a sphere expanding from the origin in all directions at the speed of light is $$ x'^2 + y'^2 + z'^2 = c^2 t'^2$$ This is a pulse of light at the origin travelling in all directions outward at c.

We use the Lorentz transform, which includes time dilation, length contraction, and the relativity of simultaneity, to transform this into the unprimed frame and obtain $$(x-tv)^2\gamma^2+y^2+z^2=\left( c t \gamma-\frac{v x \gamma}{c}\right)^2$$ which simplifies to $$x^2+y^2+z^2=c^2 t^2$$ thus showing that the speed of light is the same in both frames in all directions, not just in one specific direction.

Answer 2

Understanding simultaneity is the most important aspect of learning SR, as you will find that both length contraction and time dilation are the consequence of the lack of simultaneity.

The relativity of simultaneity means that a plane of fixed time in one frame of reference is a sloping slice through time in a frame that is moving relative to the first frame.

To take your specific example, if light leaves the stationary object at time t=0, and moves off to the left and right, after a second in the frame of the stationary object the light will be about 300,000km away in each direction. So let's suppose there happen to be clocks 300,000km away in both directions, they will each read t=1s as the light passes them.

Now from the perspective of the person moving to the right at 0.5c, when the light is passing the right hand clock, it has only travelled about 150,000km ahead of that person. Conversely, however, the light that is passing the left hand clock is about 450,000km away. How can that be resolved if the speed of light is the same in each direction from the perspective of the moving person?

Firstly, you will see straight away that the concept of time dilation cannot explain it, as around half a second appears to have passed in the forward direction (ie to the right) and about one and a half seconds seem to have passed in the opposite direction to the left.

The answer is that the moving and stationary observers disagree about what time it is at the left and right hand clocks when the light passes them. The stationary observer thinks t=1s at each of the clocks. The travelling observer thinks t=0.5 by the right hand clock, so the clock is running 0.5 seconds ahead, while the time by the left hand clock is t=1.5, so the left hand clock is lagging by 0.5s.

So to the travelling observer the stationary clocks are out of synch. It is not a matter of time dilation, but of the fact that the two events (of the light passing the left and right hand clocks) are simultaneous in the frame of the stationary observer but happen at different times in the frame of the travelling observer.

Answer 3

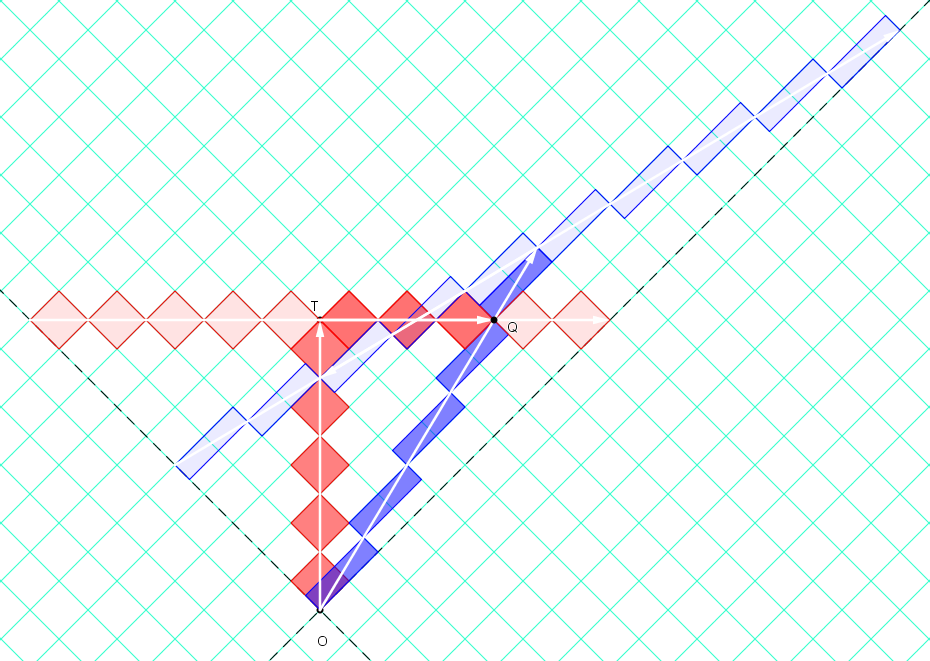
This spacetime diagram may help.
The diamonds represent the light signals in a light-clock.
The resulting diamonds give us a graphically accurate visualization of the ticks of time and space according an observer. The rotated graph paper helps us draw these diamonds for different observers.

I use $V=(3/5)$ to make the arithmetic easier.

A light-flash at event O travels in all directions.

The observer at rest in this diagram measures the velocity of the forward light-signal as $$\frac{\Delta x}{\Delta t}=\frac{(+5)}{(5)}=1,$$ because after 5 of her ticks, that light signal is 5 of her space-ticks ("sticks") in the forward direction.

The moving observer in this diagram has velocity $$V=\frac{(+3)}{(5)}.$$

The moving observer in this diagram measures the velocity of the forward light-signal as $$\frac{\Delta x'}{\Delta t'}=\frac{(+5)}{(5)}=1,$$ because after 5 of his ticks, that light signal is 5 of his space-ticks ("sticks") in the forward direction.

Similarly for the backward light-signal, both measure a velocity of $-1$.

Both of these observers measure the same value of the speed (magnitude of the velocity) of a light-signal.