The following is an image from my school textbook
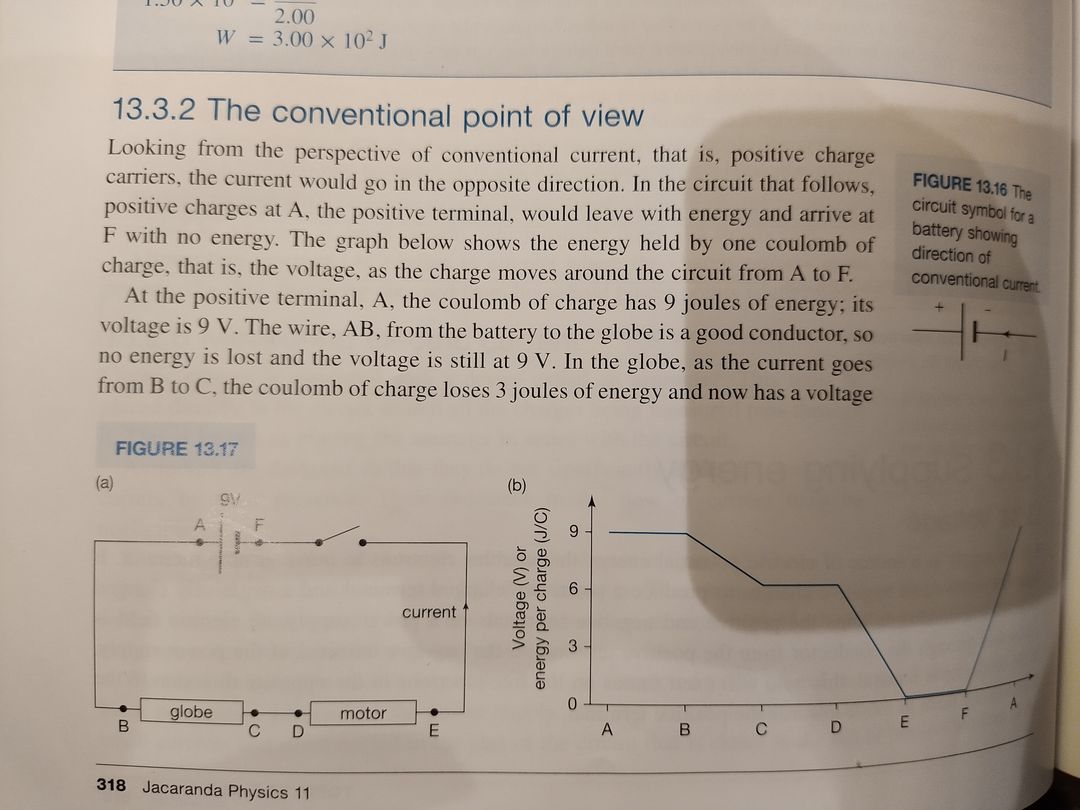
How do electrons travel from E to F is voltage (or the J per C of charge) is zero?
I think my issue is a deeper misunderstanding of the nature of voltage. I have learnt that voltage is the potential difference between two points in an electric field. This means it depends entirely on an electrons position in the electric field. So how come 'voltage' (as seen on the graph) decreases when electrons move through a resistor (e.g. light globe and motor), and not when they move between any two points on the wire (say, between A and B)? Both are simply positional changes, yet one results in a loss of electrical potential energy and the other does not.
The only solution I can work out is that the 'voltage' as shown in the graph, i.e. energy per charge, is a combination of electron's kinetic energy and potential energy. As electrons experience a force from the electric field they keep accelerating as the convert PE to KE, but the total amount of energy remains constant (assuming ideal wires). When they encounter a resistor, they lose KE and so total energy decreases. However, with this theory at point E electrons would have neither KE nor PE, and so should not be able to move to point F.
What's wrong in my understanding?
