3
$\begingroup$

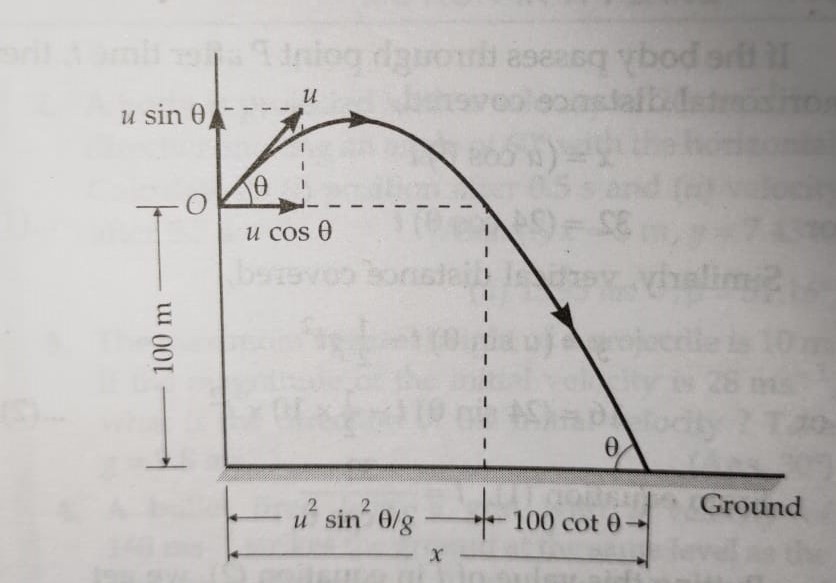
enter image description here

I came across a problem in my book with this diagram,

A machine gun is mounted on the top of a tower 100 m high. At what angle should the gun be inclined to cover a maximum range of firing on the ground below? The muzzle speed of bullet is 150 ms^-1 .

I read that when a body is projected with a certain angle from the ground it returns with the same angle and speed with which it was projected. But in this case the projectile goes below the level of projection. How does it still hit the ground at an angle $\theta$?

Improve this question
$\endgroup$
1
  • $\begingroup$ It does not hit the ground at a theta angle. In order to be sure about it, we can note that the angle is given by the tangent to the curve, which is parabolic by definition of the problem. The derivative of a parabolic function is a linear function crossing the abscissa axis at the extremum (here, maximum) level of the parabolic function. $\endgroup$
    – Balfar
    Commented Nov 18, 2021 at 10:51

1 Answer 1

Reset to default
6
$\begingroup$

It does not. The diagram is incorrect, as it assumes that the path becomes a straight line after it reaches $y = 100 \text{m}$ from the ground. The path still remains a parabola, so the final angle($\theta_f$) is different from the initial angle at which the bullet was shot ($\theta_i$)

To solve the question, find the range $R$ for any arbitrary $\theta_i$ and set $\frac{dR}{d\theta_i}$ to zero to maximise $R$ in terms of $\theta_i$.

Hope this helps.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.