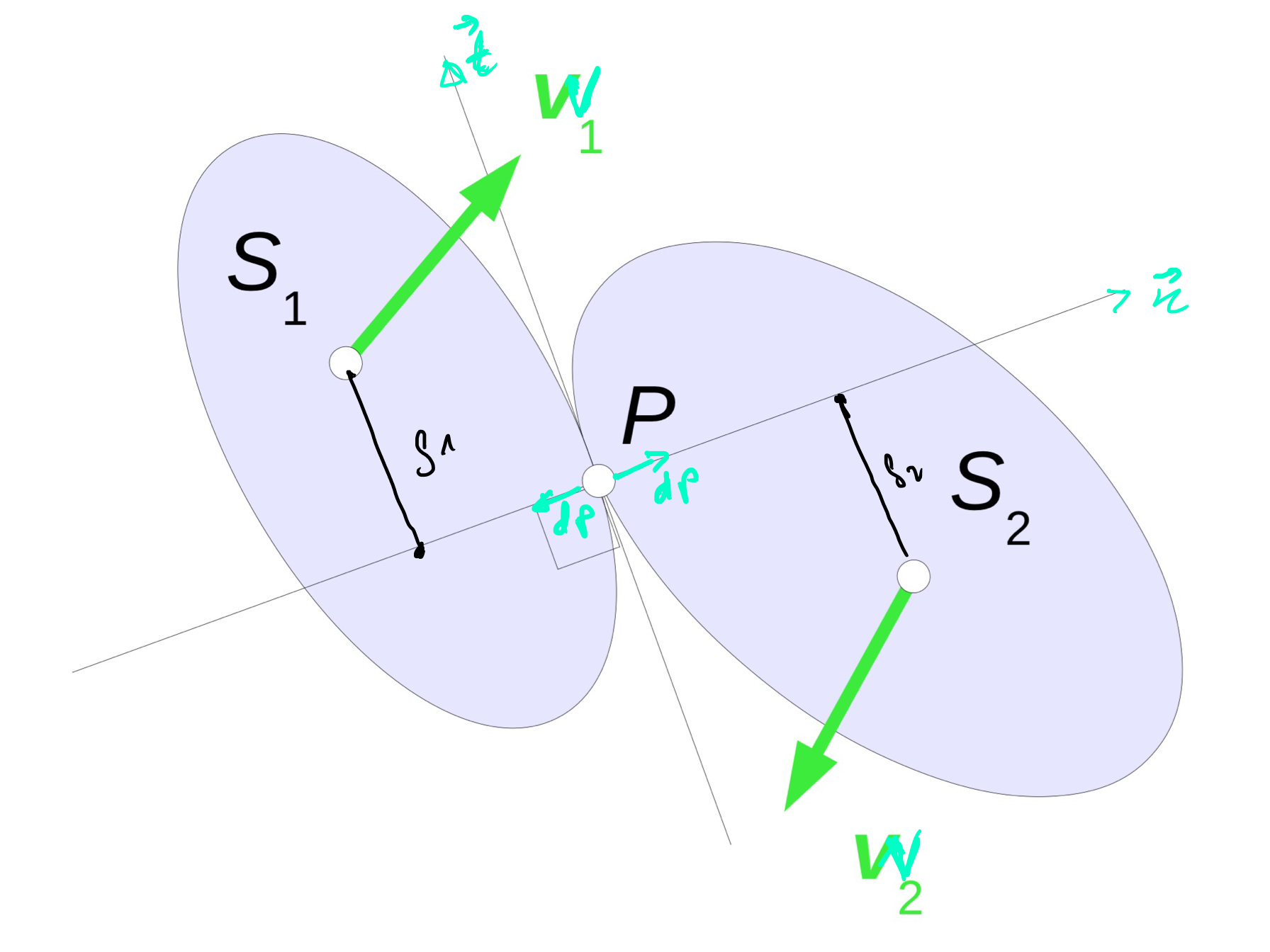
I can set up some equations for colliding spheres in D dimensions as follows. Let there be two spheres of mass $m$ and $M$ respectively. Let the first sphere have ingoing velocity vector $u$ and outgoing velocity vector $u'$. Similarly the second sphere has ingoing momenum $v$ and outgoing momentum $v'$. The balls collide tangent to an imagined hyperplane with normal $n$.
The equations are:
(1) Conservation of energy (1 equation): $$mu^2+Mv^2 = mu'^2+Mv'^2$$ (2) Conservation of momentum in direction of normal (1 equation): $$n.(mu+Mv) = n.(mu'+Mv')$$ (3) Momementum is conserved perpendicular to collision normal independently for each sphere (2D-2 equations): $$|n|^2(u-u') = n.(u-u') n$$ $$|n|^2(v-v') = n.(v-v') n$$
This gives 2D equations in which to find the pair of vectors $u'$ and $v'$.
I am trying to set up similar equations for a pair of colliding ellipsoids in D dimensions. This will involve having an antisymmetric angular momentum tensor $\omega^{ij}$ for each ellipsoid. I know the angular momentum must be conserved but not sure how to express this as equations. Additional information needed would be a vector $r$ which equals $p-x$ where $x$ is the center of the ellipsoid at collision and $p$ is the point of impact.
I'm finding it hard to find such a generalised equation in the literature. It is probably not too hard to set up though. (The objects need not be mathematical ellipsoids, just some extended object of some kind).