What is the difference between thermal conductivity and thermal diffusivity?

Question

Please excuse me, for any inability in my way to frame the question. I myself had a hard time making myself understand what was the doubt I really had :P. Also, I'm studying heat transfer at Undergraduate Level.

I first really need to make sure I understand the concept of thermal conductivity correctly or not. This is what I understand about thermal conductivity:

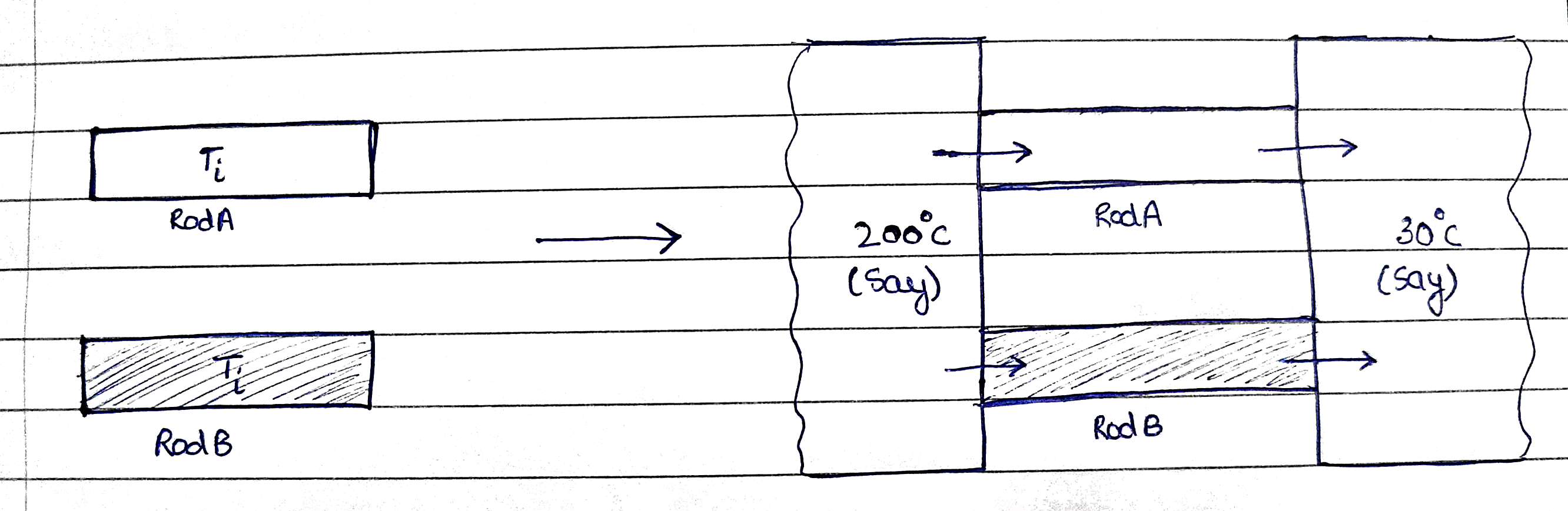
Thermal conductivity as defined in many books is a measure of a material's ability to conduct heat. A material with a high thermal conductivity will propagate heat faster within it. It tells about the speed at which thermal energy travels in a medium. So, suppose I have two identical rods- A and B made up of different materials such that thermal conductivity of A > thermal conductivity of B. Both the rods are initially at the same temperature and are brought in contact at the same time with identical heat source and heat sink. The heat transfer is one dimensional.

With the knowledge that thermal conductivity of A is greater than thermal conductivity B, I can only conclude that heat travels faster in A than in B, from one end to another. No conclusions can be made about the heat taken from the source by rod A and rod B (like I cannot tell by just a knowledge of thermal conductivity that which one of rod A and rod B will take more energy from source in any given time). Earlier I used to think that the one with a higher thermal conductivity will take more energy from the source in any given time.

Are there any other corollaries that I can make, if I know a material has a higher thermal conductivity? Corollary like if it conducts heat well, it will store less (I don't think so but..).

---

This is an excerpt from the book I'm referring to on the topic thermal diffusivity:

The sentence in the green - isn't that what thermal conductivity tells, about how well thermal energy propagates or diffuses into the medium?

The sentence in blue - just can't make sense.

Edit: Even though my question mentions 'rods', a more general explanation for difference between k and $\alpha$ will be appreciated, which is applicable to solids, liquids and gasses. As pointed out in one of the answers, solids have high thermal conductivities and high thermal diffusivities implying a solid conducts heat well and stores less. But this is not the case always a material can conduct less energy and store less also. Consider water and air for example, water has a higher $k$ than air, which means it conducts well but it does not store less (contrary to what solids do, they conduct well and store less), because water has a higher specific heat than air.

Answer 1

The rate of heat flow per unit area through a material depends on the temperature gradient in the material.The higher the temperature gradient, the higher the rate of heat flow per unit area. The constant of proportionality is the thermal conductivity k: $$q=-k\frac{dT}{dx}$$

The thermal diffusivity of a material determines how fast a temperature change at the boundary propagates into the material. If the material has a very low thermal diffusivity, the speed of the thermal "wave" propagating into the material is lower than if the diffusivity is high. This is because more energy can be stored near the boundary so the wave travels slower.

Answer 2

In essense,

• thermal conductivity, $\kappa$, is how well the material passes on heat (thermal energy), while

• thermal diffusivity, $\alpha$, is how well the material passes on a temperature change.

The diffusivity namely takes into account the heat capacity and density as well which are proporties that influence the temperature change caused by the transferred thermal energy.

Answer 3

Are there any other corollaries that I can make, if I know a material has a higher thermal conductivity?

For one thing, if you know a solid material has higher thermal conductivity chances are it will also have higher thermal diffusivity. I base this on having made a table of various solids (metals, plastics, glass, etc.) sorted by thermal conductivity (highest to lowest) and found that they were almost all sorted by thermal diffusivity as well (highest to lowest).

Corollary like if it conducts heat well, it will store less (I don't think so but..).

Actually, a solid material that conducts heat well (high $k$) does store less per unit mass because the specific heats of high conductivity materials, like metal, are low. So metals will store much less than non metals (e.g. plastics) per unit mass because the specific heat of non metals is higher then metals.

But parts have volume. So if you are comparing two identical parts of different material, you need to look at their volumetric heat capacities, $\rho c$ in the denominator of the thermal diffusivity, not their specific heats. Since the density $\rho$ of metals is much greater than plastics, the volumetric heat capacity is often not very much different between metals and non metals. For example, the volumetric heat capacity of steel and Teflon is about the same.

The sentence in the green - isn't that what thermal conductivity tells, about how well thermal energy propagates or diffuses into the medium?

Since, as I stated above, solid materials with high thermal conductivity generally also have higher thermal diffusivity, it stands to reason that the diffusion of heat in solid materials should be higher with higher thermal conductivity. But you can't determine what it actually is based only on thermal conductivity because $k\ne k/\rho c$. In order to determine how temperatures in a solid material varies as a function of time $t$ and depth $x$ under transient conditions, you need to obtain the solution to the following heat equation

$$\frac{\partial T(x,t)}{\partial t}=\alpha\frac{\partial^2 T(x,t)}{\partial x^2}$$

For the given initial and boundary conditions, where $\alpha=k/\rho c$ = thermal diffusivity.

hope this helps.