In string theory low-energy $n$-dimensional gravity is described by an action of the following form: $$S^{(n)}=\frac{1}{2\kappa^{(n)}}\int d^nx\sqrt{-G}e^{-2\Phi}\Big(\mathcal{R}+4\partial_\mu\Phi\partial^\mu\Phi-\frac{1}{12}H_{\mu\nu\rho}H^{\mu\nu\rho}\Big)\tag{1}$$ where $H_{\mu\nu\rho}=\partial_\mu B_{\nu\rho}+\partial_\nu B_{\rho\mu}+\partial_\rho B_{\mu\nu}$ (see David Tong's Lectures on String Theory ch.$7$ p.$168$ eq.$7.16$, using natural units $\hbar=c=1$ and metric signature $-$,$+$,$+$,$+$).
There are three massless bosonic fields: the space-time metric $G_{\mu\nu}$, the anti-symmetric tensor field $B_{\mu\nu}$ and the scalar dilaton field $\Phi$.
The string coupling parameter is given dynamically by the dilaton field: $$g_s=e^\Phi.\tag{2}$$ To a first approximation at tree level (see David Tong's Lectures on String Theory ch.6 p.128) the strength of the gravitational coupling between strings is given by $$\phi=\frac{1}{g_s}.\tag{3}$$ Substituting Eqns.$(2,3)$ into Eqn.$(1)$, performing a dimensional reduction to $4$-d, ignoring the $B_{\mu\nu}$ field and adding a cosmological constant $\Lambda$, we obtain the following $4$-d action $$S^{(4)}=M^2_{Pl}\int d^4x\sqrt{-g}\Big(\frac{\phi^2\mathcal{R}}{2}+2\partial_\mu\phi\partial^\mu\phi-\Lambda\Big),\tag{4}$$ where the reduced Planck mass $M_{Pl}=1/\sqrt{8\pi G}$. By setting $\phi=1$ one recovers the Einstein-Hilbert action with a cosmological constant $\Lambda$. It is interesting to note that the $\Lambda$ term breaks the scale-invariance symmetry of the action in Eqn.$(4)$ allowing for time evolution of the universe.
In order to find a cosmological solution we use the FLRW metric in a generalized form whose line element is given by $$ds^2=-dt^2+a^2(t)\gamma_{ij}(u)du^i du^j\tag{4.5}$$ where $t$ is the timelike coordinate, $a(t)$ is the scale factor and $\gamma_{ij}$ is the metric on a maximally symmetric $3$-space with coordinates $u^1,u^2,u^3$.
We wish to find the cosmological equations of motion of the action in Eqn.$(4)$. In order to do this we first transform to a time coordinate $\eta$ with an arbitrary lapse function using $dt=N(\eta)d\eta$. The equations of motion can be derived from generalized Euler-Lagrange equations, using time $\eta$, for the dilaton field $\phi,\phi'$ $(5)$, lapse function $N,N'$ $(6)$ and scale factor $a,a',a''$ $(7)$. After transforming back to time $t$ the equations of motion are: \begin{eqnarray} \ddot{\phi}+3\frac{\dot{a}}{a}\dot{\phi}+\frac{3}{2}\phi\Big(\frac{\ddot{a}}{a}+\frac{\dot{a}^2}{a^2}+\frac{k}{a^2}\Big) &=& 0,\tag{5}\\ \phi^2\Big(\frac{\dot{a}^2}{a^2}+\frac{k}{a^2}\Big)+\frac{2}{3}\dot{\phi}^2+2\frac{\dot{a}}{a}\dot{\phi}\phi-\frac{\Lambda}{3} &=& 0,\tag{6}\\ \phi^2\Big(2\frac{\ddot{a}}{a}+\frac{\dot{a}^2}{a^2}+\frac{k}{a^2}\Big)+4\frac{\dot{a}}{a}\dot{\phi}\phi+2\ddot{\phi}\phi-\Lambda &=& 0.\tag{7} \end{eqnarray}
The above equations of motion are also described in Eqns.$(7,8,9)$ of this paper with $\omega=-1$, $\phi \rightarrow 2 i \phi$, $p_M=-\rho_M$ and $\Lambda=\rho_M$.
Scale change leads to effective universe expansion
Now I make the assumption that the apparent expansion of the universe is entirely due to the increase in the dilaton field $\phi(t)$ so that we have $$\phi(t)=a(t).\tag{8}$$
As $\phi(t)$ increases the masses of all particles increase and therefore their sizes decrease according to the uncertainty principle of quantum mechanics. This decrease of the length of rulers leads to an effective increase in the scale factor $a(t)$.
In fact this assumption realizes the global rescaling symmetry of the FLRW metric (in polar coordinates: $a \rightarrow \lambda a$, $r \rightarrow r/\lambda$, $k \rightarrow \lambda^2 k$).
By substituting Eqn.$(8)$ into Eqns.$(5,6,7)$ we find a unique vacuum solution given by: \begin{eqnarray} a(t) &=& H_0\ t,\tag{9}\\ k &=& -3H_0^2,\tag{10}\\ \Lambda &=& 2H_0^2\tag{11}\\ \end{eqnarray} where $H_0$ is the Hubble constant.
The vacuum energy density is given by $$\rho_\Lambda=\Lambda\ M_{Pl}^2 = 2 H_0^2 M_{Pl}^2.\tag{12}$$
Thus we obtain a spatially open universe that only seems to be expanding because particle sizes are decreasing. The cosmological redshift is then explained by the increase of energies of present-day absorbing atoms relative to past emitting atoms.
In fact the effective linear expansion of space given by $a(t)=H_0\ t$ for an empty universe with a cosmological constant $\Lambda$ is a very good fit to the cosmological expansion data for the actual universe according to researchers like Fulvio Melia (see observational support for his $R_h=ct$ model). It is certainly true that the linearly expanding vacuum solution of the present model is much better "behaved" than the exponentially expanding vacuum solution of standard general relativity.
The energy scale $E_\Lambda$ for the energy density $\rho_\Lambda$, given in Eqn.$(12)$, is approximately
$$E_\Lambda = \rho^{1/4}_\Lambda = H^{1/2}_0 M^{1/2}_{Pl},\tag{12.1}$$
$$\log{E_\Lambda} = \frac{1}{2}(\log{H_0}+\log{M_{Pl}}).\tag{12.2}$$
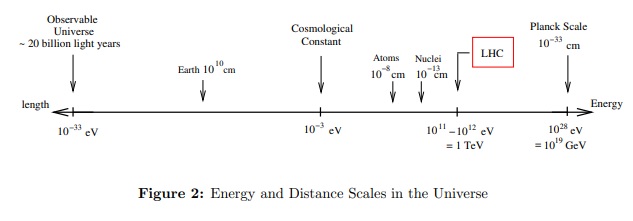
Therefore the energy $E_\Lambda$ of a model universe with a cosmological constant is halfway between the Hubble constant and the Planck mass on a logarithmic scale in accordance with the following diagram by David Tong in his Quantum Field Theory Lectures, ch.$0$, p.$5$.
Possible experimental test
Consider two charged massive spheres both with mass $m$ and charge $q$ such that their gravitational attraction is balanced by their electrostatic repulsion.

Thus, using $8\pi G=M^{-2}_{Pl}$, we have $$\frac{q^2}{4\pi\epsilon_0 d^2}=\frac{G m^2}{d^2}=\frac{m^2/M^2_{Pl}}{8\pi d^2},\tag{12}$$ $$\frac{2q^2}{\epsilon_0}=\frac{m^2}{M^2_{Pl}}.\tag{13}$$ The balance of forces only depends on a ratio of masses which does not change as the dilaton field $\phi(t)$ increases. Therefore the separation $d$ between the centers of the spheres does not change. But as $\phi(t)$ increases the size of the atoms in the spheres decrease so that the radii $r$ decrease. Let us assume that we measure the distance $x=d-2r$ between the spheres by comparing it with $r$ so that we have $$\frac{x}{r}=\frac{d}{r}-2.\tag{14}$$ If the increasing dilaton field $\phi(t)$ causes masses to increase and length scales to decrease then our measurement of the separation distance ratio $x/r$ should increase. This effect should occur for a table-top experiment with a fixed large mass and a small mass on a frictionless track. The fractional change in the effective separation distance would be roughly $10^{-10}$ per year so that it would have to be measured using laser interferometry.