Conflict between a system lowering its potential energy and kinetic energy

Question

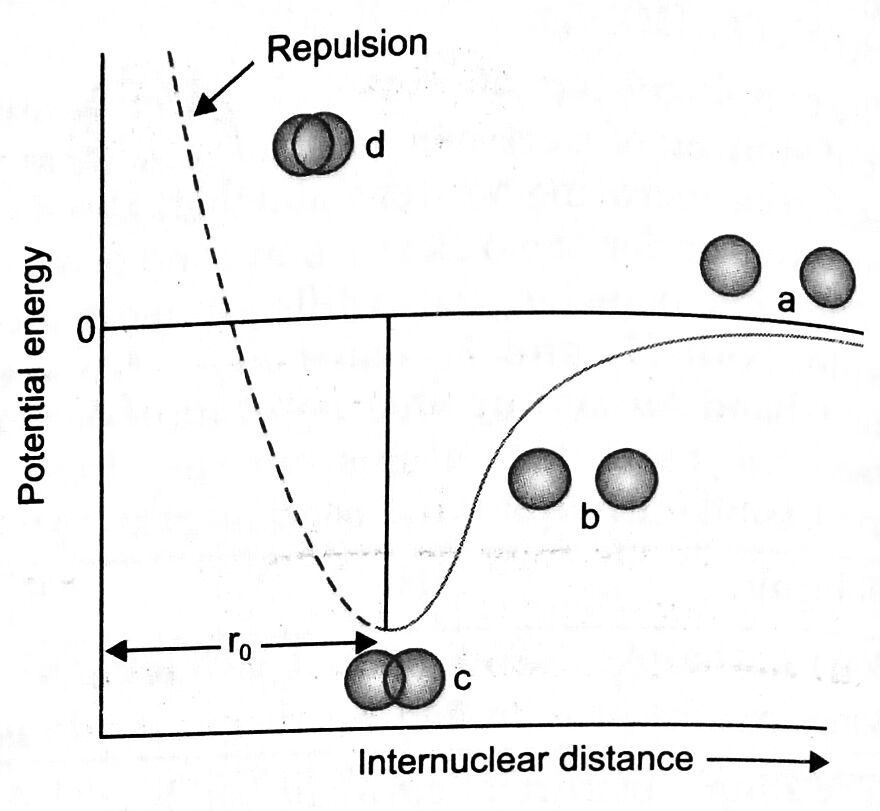
Every system in the universe has a natural tendency to attain a state of minimum energy. The state of minimum energy corresponds to a state of maximum stability.

These are from a renowned book. I am facing some problems in accepting this statement.
From the law of conservation of energy I can conclude that a decrease in potential energy must be accompanied with an increase in kinetic energy. So why is it that every system wants to decrease potential energy and not kinetic energy? Matter we see around us could have split into constituent particles with infinite separation but why does that not happen?

Addendum

(Replying to an answer)

Infinite separation is not the minimum potential energy that can be achieved but why does the system wants to minimize its potential energy and not kinetic energy? For better understanding considering this: a ball is dropped from a tall building. It lowers its potential energy with an subsequent increase in kinetic energy and hits the surface. Now revert the clock back. The ball moves from the surface to the top of the building with its velocity gradually decreasing(N.B. The direction of velocity is downwards). This process of course violates fundamental definitions like $$\vec{a} = \frac{d\vec{v}}{dt}$$ but is energetically favourable. I favour energy conservation before anything else because it reduces calculations and simplifies problems. But why does it fail in this case?

Answer 1

Good question. TL;DR: saying that systems want to minimize energy is some kind of heuristic, but it is not a true law.

The statement that systems have a tendency to lower their energy is a sloppy rephrasing of the second law of thermodynamics which favours disorder i.e. homogeneous distribution of energy across all degrees of freedom (DOF). And in the approximation here, the system is interacting with an environment which has an infinite amount of such DOF.

More technically, when a system can exchange energy with such an infinite environment (also 'bath' or 'reservoir'), given any absence of driving that prevents it from reaching equilibrium, it will strive towards a configuration that minimizes the free energy

$F=E-TS$

where $E$ is the system's energy, $T$ the temperature of the bath and $S$ the entropy. For macroscopic systems at room temperature, the second term is often negligible.

Note that the situation is different in isolated systems that cannot exchange energy with their environment (dissipation), then there is no relaxation and energy is solely exchanged between potential and kinetic. The typical example is a pendulum, which would go on oscillating forever in absence of such dissipation. Also for example for the solar system, we are lucky that there is no efficient channel for the earth to dissipate its gravitational energy, otherwise we would collapse into the sun.