The direction of the velocity of a body can change when its acceleration is constant. How is it possible since acceleration is a vector quantity?

Question

As we already know that acceleration is a vector quantity which means that it has both direction as well as magnitude. It can also change given any one of the two or both (magnitude and direction) changes.

Yet in the high school physics textbook of mine, it explicitly states that “the direction of the velocity of a body can change when its acceleration is constant” as a true statement which seems to contradict the premise which we have established.

How can this be possible since acceleration should also change (not constant but become variable) if the direction of the velocity of a body changes given that in uniform circular motion the body is under variable acceleration as the direction of the body is changing and so not constant acceleration?

Is this case not similar to uniform circular motion? If not, please explain.

Answer 1

Projectile motion is motion under constant acceleration. Projectiles move on a parabolic path where both the magnitude and the direction of the velocity are continuously changing but the magnitude and the direction of the acceleration are not changing. The textbook is correct.

Answer 2

This is possible. Let us suppose a body moves in the positive $x$ direction with a constant velocity $v_x$. We provide a constant acceleration in the $y$ direction equal to $a$. After a time $t$, the velocity of the body will be $v = v_x \hat{x} +at\hat{y}$. The direction changed by $\theta = \tan^{-1}\frac{at}{v_x}$. This happens in many cases, and one example you must be familiar with is projectile motion, where a constant acceleration of $g$ changes the direction of motion of the body projected.

Answer 3

You do need variable acceleration (with constant magnitude) to maintain uniform circular motion, as centripetal $\vec a$ must remain perpendicular to tangential $\vec v$ at all instants. But for a body moving with an arbitrary velocity $\vec v = v_0 \hat i + v_1\hat j + v_2\hat k$, any constant, non-zero acceleration that is not along the direction of $\vec v$ must change the component of $\vec v$ along $\hat i$, $\hat j$ or $\hat k$. That is what it means to accelerate, to change the velocity. We're just doing it in a different direction to that of $\vec v$.

Also, if you are confused about this, $\vec v$ has a dependence on the direction of $\vec a$, but $\vec a$ can always exist on a body without any influence from its velocity. Uniform circular motion, or rather any kind of motion in a closed loop, like the elliptical motion of planets, requires $\vec a$ to have a dependence on $\vec v$, as what is consistent in any motion in a loop, we must first travel in one direction until we reach a point of maxima, and then accelerate the $\vec v$ to move in the other direction, until a point of minima, upon which we again need to accelerate in the other direction.

Since each point in the trajectory corresponds to a particular direction of $\vec v$, we can clearly see how the $\vec a$ is dependent on which way $\vec v$ is pointing.

Answer 4

An object can have a changing direction of velocity, and this is still called an acceleration, but the acceleration itself need not change (constant acceleration, meaning its magnitude and direction stay the same).

Consider an object moving as a projectile on earth's surface $$\vec x(t) = \vec v_0 t+\frac{1}{2}\vec gt^2$$ $$\vec v=v_x\hat i+gt\hat j$$ At any given time the direction of velocity changes even though there is a constant acceleration $\vec g$ pointing to earth (center of earth). At launch, it has components of velocity both in the vertical and horizontal direction $$\vec v_0= v_{0x}\hat i + v_{0y}\hat j$$ and when it has reached its maximum height it has only a horizontal component, then both directions change again till it hits the ground. The whole time $\vec g$ keeps its magnitude and direction constant.

Answer 5

Let's try to understand it using an example:

Initially if a body had a certain velocity in the positive x direction and the acceleration in the negative x direction then as the time progresses the velocity of the body would keep decreasing. Eventually it would become zero followed by a velocity in the negative x direction. So, the direction of its velocity changed while its acceleration was constant all along.

Answer 6

Perhaps the easiest way to see this is to imagine a body with a velocity initially in the y direction, V_y. If the body is then subject to a constant force in the x direction, it will gain an ever increasing component of velocity V_x in that direction. As a result of V_y remaining fixed and V_x continually changing, the velocity V of the body will continually change direction, coming to point more and more in the x direction.

You cannot achieve circular motion with a constant acceleration, as the direction of the acceleration must continually change to remain pointed at the centre as the body moves around the circumference.

Answer 7

Is this case not similar to uniform circular motion? If not, please explain.

Not fully, no. The situation with uniform circular motion is actually slightly more complicated; this is a simpler case.

How can this be possible since acceleration should also change (not constant but become variable) if the direction of the velocity of a body changes given that in uniform circular motion the body is under variable acceleration as the direction of the body is changing and so not constant acceleration?

I think what's confusing you is that you're thinking of the acceleration as of an intrinsic property, a vector attached to the body. But acceleration is supplied by external forces. In uniform circular motion, the direction of the acceleration is not changing because the direction/velocity of the body is changing, but because the position of the body is changing in relation to the source of the force.

But that's just an extra complication. The changing velocity direction is not caused^* by the change in acceleration, but is the consequence of the fact that the acceleration has a component perpendicular to the velocity (it's not pointing in the same direction).

---

^* Note that I'm talking about what causes what; there is, of course, a correlation between velocity and acceleration in both directions, but I think your confusion is partly a consequence of a misinterpretation of the causal relationship.

---

Any non-zero acceleration will by its nature affect the velocity of an object by "just existing" (the acceleration doesn't need to "do" anything extra). An acceleration encodes a change in velocity; the unit for it is $m/s^2$, which is just another way of writing:

$$\frac{m/s}{s}$$

As you know, $m/s$ is the unit of velocity, so an acceleration vector encodes by how much a velocity vector would change after one second, and in which direction, assuming constant acceleration. It reproduces the $\Delta\vec{v}$ vector, that you can vectorially add to the current velocity vector $\vec{v}$ to get the new velocity vector $\vec{v}_1 = \vec{v} + \Delta\vec{v}$. Or, for an arbitrary time period, $\Delta\vec{v} = \Delta t \vec{a}$ and $\vec{v}_1 = \vec{v} + \Delta t \vec{a}$.
(You'd often just write that as $\vec{v}_1 = \vec{v} + t \vec{a}$, taking $t_0$ to be zero.)

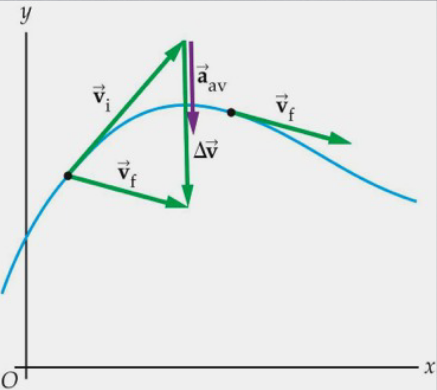
For example, in this image, after some chosen time $t$, the velocity changes from an initial value of $\vec{v}_i$ to the final $\vec{v}_f$ (to the right, on the trajectory). Doing the subtraction $\vec{v}_f - \vec{v}_i$ gives you the change in velocity $\Delta\vec{v}$. Assuming again constant acceleration^**, dividing $\Delta\vec{v}/t$ (where $t$ is the time span) gives you a sort of "standardized" change in velocity — the one that happens per single second — that we call acceleration. Then you can scale (multiply) that by time to get the change in velocity for any $\Delta t$ (even going backwards). So for some time span $t$, the change is $\Delta\vec{v} = t\vec{a}$ and, $\vec{v}_f = \vec{v}_i + \Delta \vec{v}$.

---

^** This is just some image I found on the Internet; the trajectory for a constant acceleration would not look like this (it wouldn't curve back at the other end as depicted, it would instead be a parabola). But let's ignore that for now.

---

So you see, the direction is changing because by applying an acceleration you're actually vectorially adding to the initial velocity a $\Delta \vec{v}$ that doesn't have the same direction as it, which makes it turn.

The math is more complicated with variable acceleration and involves integration, but the basic idea and the underlying logic is the same. For a changing acceleration, you're interested in the instantaneous value of the acceleration, which you can get closer and closer to by considering smaller and smaller $\Delta t$; for tiny $\Delta t$, the change in velocity is practically very simple (the technical term is linear), as if the acceleration was constant for the duration of that small timeframe - so you can apply the same trick of "standardizing" the change in velocity (which gives you a value of for the acceleration that is the limit of the average acceleration as $\Delta t \to 0$, and otherwise makes the math work out).