What is "field size" when referring to perspective?

Question

I'm trying to get my head around perspective. If you look at the animation in Wikipedia :

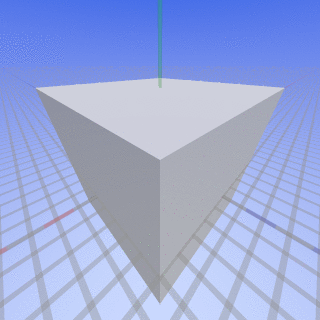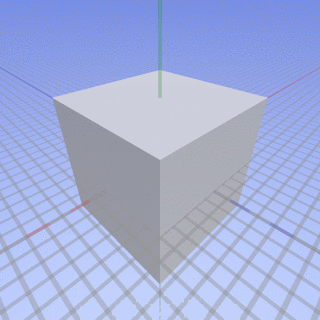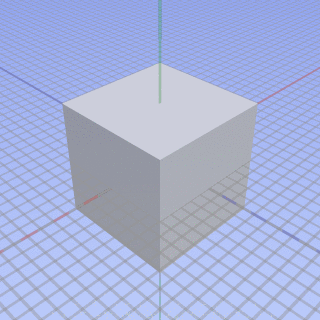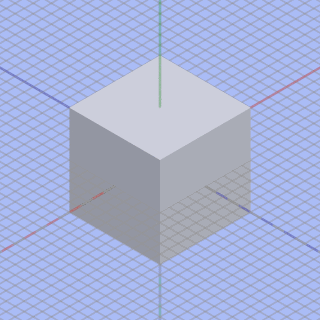
You can see that the cube looks very distorted, then the edges go more parallel.

From my understanding, the distorted version is when the camera is very close to the cube, and the less distorted version is when the camera is further away.

But if the camera is further away, why does the cube not appear smaller?

Is this something to do with keeping the "field size" constant? Is the field size a flat area? Or part of the area of a sphere? It looks as though you can see more stuff in the background and foreground when you're zoomed out compared to zoomed in, so how can you know that you're keeping the "field size" constant?

Answer 1

The animation does not represent what our eyes would see.

If you go far away from the cube you will see its faces more parallel, but the cube will be smaller, just as you explained. You can then use binoculars to make it bigger, and the faces will remain parallel.

The computed image scales the image up to make it appear bigger, just like what binoculars do.

In optics terms, the eye sees an object with a given magnification (ratio of sizes) and the computer adds an additional magnifying power (ratio of angles) such as the one provided by binoculars.

Answer 2

What they did in the picture is to move away from the object along with "changing the lens". So you are right, the cube is not "photographed" from the same distance all the time, but they keep the same relative size of it through applying longer/shorter lenses. Effectively, the field size of the photographs (distance from the closest points included in them to the furthest) is approximately the same.

This shows two different aspects of perspective distortion as introduced by camera.

There is this normal distortion perspective that everybody can see with their own eyes, without the aid of a camera according to which parallel lines meet together in infinity and closer objects are larger than those further away.

But there is also the distortion introduced by the fact that the camera takes pictures with one "eye" only and when a relatively large object is very close to it, the outer-most (to the left and to the right) points are much further away from the centre of optics than the middle ones. So their relative size is different then it is in reality - the closer ones are bigger and those further away are smaller. When we look with two eyes this effect is minimized, as each of the eyes is closer to one of the edges of the object we see from close distance.