Resolving forces on a banked track

Question

This is basically the same question as Why resolve some forces into components and not others?

However, I don't understand how the preferance of coordinate systems affect the resolution of forces.

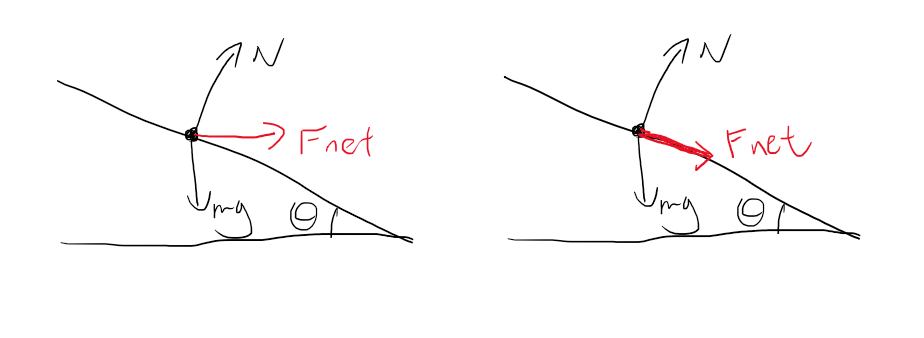
In the diagram above, if we resolve the forces with respect to the horizontal axes, that is the one of the left, we get \begin{equation} F_N=\dfrac{mg}{\cos \theta} \end{equation} whereas if we resolve the forces diagonally with respect to the incline, such as on the right, we get \begin{equation} F_N=mg\cos\theta \end{equation} These two quantities are obviously different, and I fail to see how a choosing one coordinate system over another changes the value of $F_N$.

$\underline{\textbf{My attempt at understanding}}$

Apart from the axes argument, there is also this post Looking for an intuitive understanding of normal force that explains it (or so as I interpreted it) as a result of the direction of motion, because when an object slides down an incline, the motion of along the slope, thus $F_N$ cancels off forces in any other direction, whereas on a banked road the direction of motion is horizontal, namely towards the centre, so $F_N$ is such that the net force is in that direction.

This seems to make a bit more sense to me. But then again the choice of the direction of motion seems to be rather arbitrary, what if the direction is $10^\circ$ north of east, does $F_N$ change according to that?

Thank you in advance.

Answer 1

You can only equate opposite forces in a given direction if you know that the object is not accelerating in that direction, and so the net force acting on it in that direction must be zero.

If the object is sliding down the slope then it is not accelerating in a direction perpendicular to the slope, so it makes sense to resolve forces along axes parallel to and perpendicular to the slope.

If the object is travelling around a banked curve at a constant height and speed then it is not accelerating vertically, so it makes sense to resolve forces along horizontal and vertical axes.

In other words, to determine the net force on an object if one of the forces acting on it (in this case the normal force) has a known direction but an unknown magnitude, then you need to know not just the position of the object, but also the direction in which it is accelerating.