Why don't we use rocket engines in cars?

Question

If rocket engines can reach 70% efficiency, why don't we use them in cars?

Internal combustion engines get less and less efficient with higher speed just due to the kinetic energy equation (a unit of fuel produces a unit of velocity^2), while rocket engines have a constant efficiency (a unit of fuel produces a unit of velocity), ignoring fuel consumption.

This is a fundamental constaint, since an internal combustion engine necessarily pushes against the moving earth to propel the car, and a rocket engine effectively pushes against a gas that is stationary wrt the rocket.

So why not use a rocket engine in a car? Wouldn't it be more fuel-efficent?

reference for 70% figure: https://en.wikipedia.org/wiki/Propulsive_efficiency

Answer 1

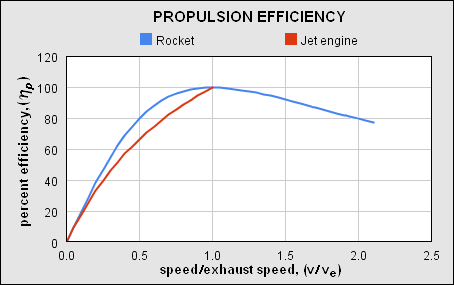
I think you've misread the article. It says rocket engines can attain up to 70% $\eta_c$, which is only the cycle efficiency (how well it turns the energy of the fuel into mechanical energy). This is not the propulsive efficiency.

Unfortunately, for a rocket much of this mechanical energy is used to (wasted..) increase the KE of the exhaust rather than the rocket. As the article mentions, optimum efficiency is when the exhaust speed and rocket speed are matched. But this ends up being horrible for fuel consumption.

Being able to throw the mass of the earth or the atmosphere around makes regular propulsion much more efficient.

In one of your comments you linked to the question Velocity and kinetic energy, violating galilean relativity and said that the efficiency of a car drops with speed. I wouldn't agree with that statement. The question was specifically about interpreting energy in different frames.

If we stick to to just the frame where the ground is at rest (a very valid frame for travel on the earth), then the theoretical efficiency of your battery car approaches 1 as you eliminate drag. The energy of the battery can be given into KE of the vehicle almost entirely since the earth is so massive.

---

Do cars legitimately use 100X as much fuel to go 100km/h => 101km/h as 0km/h => 1km/h?

In the frame of the ground, yes (although it's more like 200x).

It's because cars are pushing on the ground. When the ground is going backward at 100km/h, it's much, much harder to push forward along it. You probably need additional gearing to make the wheels go faster compared to your power source. But when you do that, the force on the power plant goes up.

And do rockets not have this problem?

(Useful) rockets don't start off moving at high speed. They start off moving at slow speed. When at slow speed the fuel burn "pays" for accelerating both the rocket and the remaining fuel.

Later, the rocket can use the accelerated fuel (which now has much more energy than it did on the ground) to accelerate even faster.

The rocket is using not only the chemical energy content of the fuel, it is also using the KE of the fuel. We ignore that in the car. It's like the first part of the burn is also charging the fuel. We get to burn that charged fuel at the end, so it works better.

So is it true that, at high speeds, fundamentally a car is staggeringly less fuel-efficient than a rocket?

Yes. If the only way you can accelerate is by pushing backward on the ground, and the ground is receding from you at 1km/s, you're going to have a very tough time accelerating. There are no cars that can do it, even if there were zero rolling/air resistance.

Answer 2

Why don't we use rocket engines in cars?

The claim is not quite true: many of the land speed records were established using cars with jet engines, as, e.g., ThrustSSC:

(image source)

There is however some dispute as to whether such a vehicle could be still called a "car/automobile", or whether the term should be reserved to the vehicles with internal combustion engines and the electric ones.

Wikipedia Rocket engine article provides the answer to the Q.:

For a vehicle employing a rocket engine the energetic efficiency is very good if the vehicle speed approaches or somewhat exceeds the exhaust velocity (relative to launch); but at low speeds the energy efficiency goes to 0% at zero speed (as with all jet propulsion). See Rocket energy efficiency for more details.

Taking the graph from the Propulsive efficiency article quoted in the Q.:

Typical exhaust velocities of the existing jet and rocket engines are given in the table below (see Specific impulse):

A typical car speed is $70$mph$\approx 110$km/h $\approx 30$m/s - this speed is simply too low to take advantage of the rocket technology.

Note further that the speed of sound is $340$m/s$\approx 1200$km/h$\approx 800$mph - the exhaust speeds, and the vehicle speeds, need to approach this value to have reasonable efficiency. This is true for the commercial airplanes, which move at about half of the speed of sound and higher, but not really an option for commercial road vehicles.

Answer 3

Because on the ground where friction commands,- you can't speed-up much unless you are riding on the straight highway and still if you will speed there up to $500~mph$ or more,- you will forget to make the right exit, or deceleration will be highly unpleasant and/or risk of car accidents on the highway will increase exponentially by each $100~mph$ added.

That's why,- car roads are not suitable for great speeds, so you can use rocket engine on the car only for breaking some speed Guinness World Record.

Not to mention where all citizens will buy required tons of rocket fuel ? And the effect of that on the global warming and Earth ecological issues.

Greediness always makes more trouble.

Answer 4

These answerers are all really dumb.

A unit of fuel produces a unit of v^2 in BOTH examples. The question is wrong.

Answer 5

From one of your comments:

So is it true that a car uses 100X as much fuel to go 100km/h => 101km/h as 0km/h => 1km/h? And rockets do not have this constraint?

No, rockets have the same constraint.

Fuel usage is usually expressed in distance/unit volume of fuel ie. miles per gallon (mpg) so the distance covered is the metric and from kinematic equations.

$$ v^2 = v_0^2 + 2ad$$ or $$ d = \frac{v^2-v_0^2}{2a}$$

From the equation above, the distance is proportional to $v^2$ but if the acceleration of the rocket and car are the same and both have fuel usage expressed in mpg, then a car and a rocket will travel the same distance given the same initial and final velocities.