I need to check units in this equation, I know, that there's Pa in the numerator, but what about the denominator?
$p_1$ - pressure in [Pa]
$T_1$, $T_2$ - temperature in [K]
$\rho_w$ - density
$g$ - acceleration
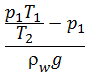
I need to check units in this equation, I know, that there's Pa in the numerator, but what about the denominator?
$p_1$ - pressure in [Pa]
$T_1$, $T_2$ - temperature in [K]
$\rho_w$ - density
$g$ - acceleration
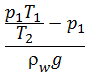
You know that $\rho gh$ is defined as pressure inside a liquid at a depth $h$ . Now you can get dimensions of denominator. $$\rho gh\equiv[Pa]$$
Density is mass per volume, so that we get: $\dfrac{kg}{m^3}$
Acceleration is $\dfrac{m}{s^2}$
The two multiplied together gives you: $\dfrac{kg}{s^2m^2}$
Now, if you want to turn this into the SI units of pressure, you can, since the units of pressure can be derived from depth, density and acceleration due to gravity ($P = h\rho g$) and you get: $$\text{Units of pressure}: \text{Pa} = m\times \dfrac{kg}{m^3} \times \dfrac{m}{s^2}$$
You can recognize the second part, so it becomes $\dfrac{\text{Pa}}{m} = \dfrac{kg}{s^2m^2}$
Above: $\text{Pa}$;
Below: $\dfrac{\text{Pa}}{m}$