In cartesian coordinates, a particle under an arbitrary potential $U(x,y,z)$ will have a Lagrangian $$L=\frac{m}{2}\left(\dot{x}^{2}+\dot{y}^{2}+\dot{z}^{2}\right)-U(x,y,z)$$ Consequently, the canonical conjugate momenta are $p_{x} =\frac{\partial L}{\partial \dot{x}}=m \dot{x}$, $p_{y} =\frac{\partial L}{\partial \dot{y}}=m \dot{y}$, and $p_{z} =\frac{\partial L}{\partial \dot{z}}=m \dot{z}$. They represent the linear momentum of the particle over each coordinate $x$, $y$ and $z$.
Similarly, in cylindrical coordinates the lagrangian will be $$L=\frac{m}{2}\left(\dot{\rho}^{2}+\rho^{2} \dot{\phi}^{2}+\dot{z}^{2}\right)-U(\rho, \phi,z)$$
With the associated conjugated canonical momenta $p_{\rho} =\frac{\partial L}{\partial \dot{\rho}}=m \dot{\rho}$, $p_{\phi} =\frac{\partial L}{\partial \dot{\phi}}=m \rho^{2} \dot{\phi}$, and $p_{z} =\frac{\partial L}{\partial \dot{z}}=m \dot{z}$. As I understand it, in this case, $p_\rho$ and $p_z$ would represent the linear momentums over the radial and vertical directions given by $\hat{u}_\rho$ and $\hat{u}_z$, whereas $p_\phi$ would correspond to the angular momentum of the particle rotating around the $Z$ axis.
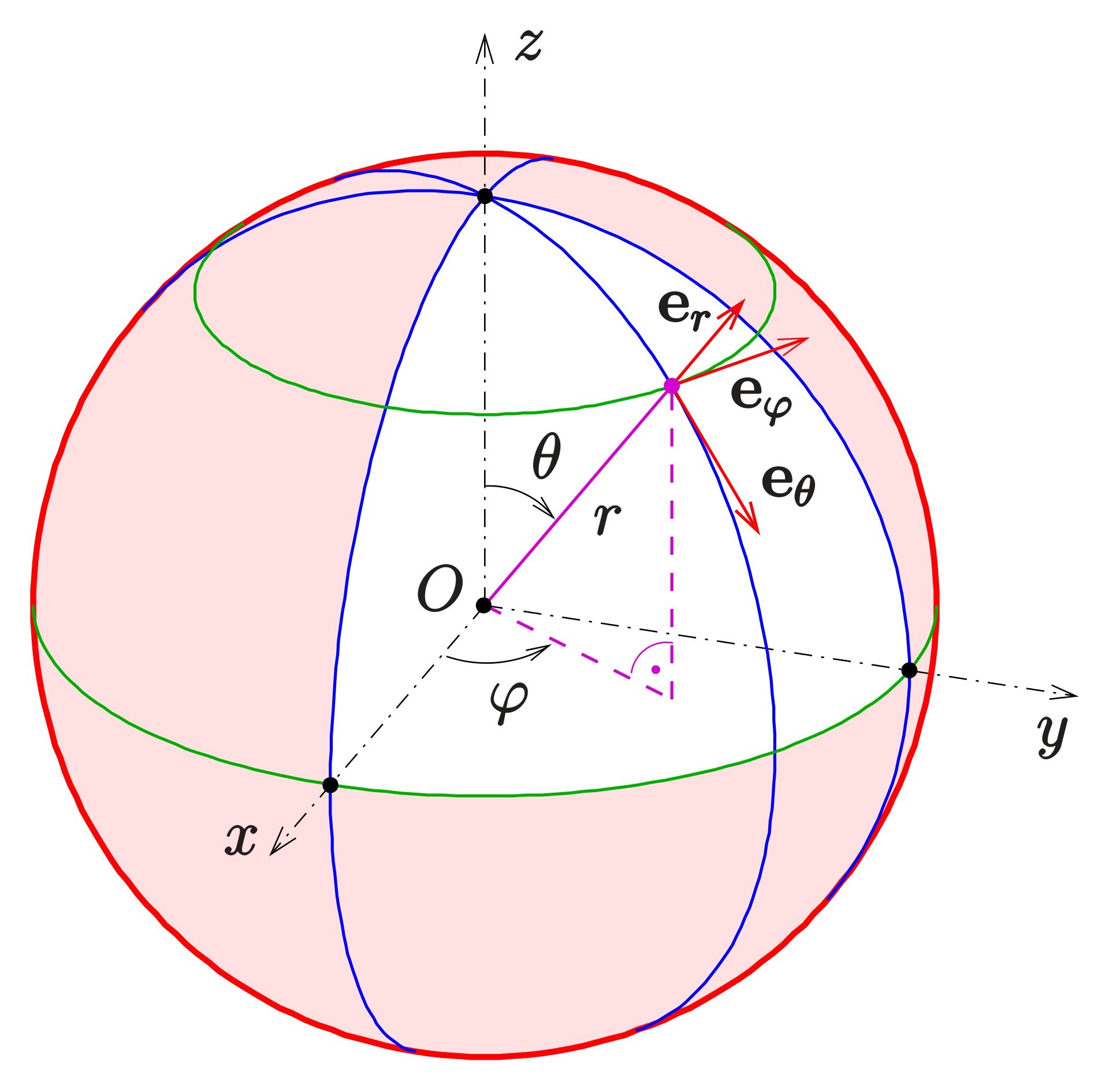
Finally, in spherical coordinates, $L=T-U=\frac{m}{2}\left(\dot{r}^{2}+r^{2} \dot{\theta}^{2}+r^{2} \sin ^{2} \theta \dot{\phi}^{2}\right)-U(r, \theta, \phi) $, and
$$ \begin{aligned} p_{r} &=\frac{\partial L}{\partial \dot{r}}=m \dot{r} \\ p_{\theta} &=\frac{\partial L}{\partial \dot{\theta}}=m r^{2} \dot{\theta} \\ p_{\phi} &=\frac{\partial L}{\partial \dot{\phi}}=m r^{2} \sin ^{2} \theta \dot{\phi} \end{aligned} $$
While I see that $p_r$ would have a similar meaning to $p_\rho$ in cylindrical coordinates, what would be the meaning of $p_\theta$ and $p_\phi$ in this case?