Lagrange's equation - thin disk that rolls without slipping on a horizontal plane

Question

The Goldstein book has the following question (1.11):

Consider a uniform thin disk that rolls without slipping on a horizontal plane. A horizontal force is applied to the center of the disk and in a direction parallel to the plane of the disk.

(a) Derive Lagrange’s equations and find the generalized force.

(b) Discuss the motion if the force is not applied parallel to the plane of the disk.

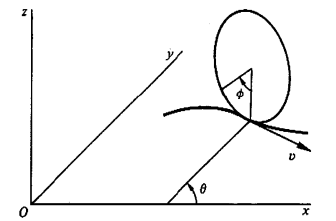
In the previous pages, they showed a similar scenario with the following illustration:

In this case, the coordinates $x$ and $y$ are the coordinates of the center of the disk, an angle of rotation $\phi$ about the axis of the disk, and an angle $\theta$ between the axis of the disk and $x$ axis. If I understood right, the disk just rolls in one direction, so I can resume the question to a movement in one direction, just about one axis, like what was done here.

But I saw here an explanation using all the coordinates from the book. But this does unnecessary to me, and one special result it's specifically strange. Solving the Lagrange equation for $\theta$ they found these results:

$$a^2\ddot{\theta}+\frac{3\cos\theta}{\sin^3\theta}\dot{x}^2=0$$

I think that this has something wrong, because if the center of mass stays the movement in the same direction by all the time, and this is the situation that I understood that is the question, so $\theta$ is constant, but then $\ddot{\theta}=0$, so $\dot{x}=0$. And this isn't a result that sounds physically correct to me.

So, this last equation is really wrong, or it's I that understood something wrong?

Answer 1

The method from the quizlet link appears incorrect to me as well. If you apply a force to the center of the disk which is not parallel to the plane of the disk, then we can generally decompose it into a component parallel to the plane of the disk and one that is perpendicular to it, so parallel to the disk's symmetry axis (I'm assuming for simplicity that the force does not have a vertical component, which would go along it's vertical diameter). In that case, two coordinates are needed to describe the motion of the disk, namely the angle of rotation around the disk's symmetry axis (due to the component of the force parallel to the disk's plane and the coupling of the frictious force along the same direction) and the angle of rotation about the disk's vertical diameter (due to the component of the force parallel with the disk's symmetry axis). In principle, you could also have a torque pointing along its horizontal diameter which would effectively cause the disk to fall over if it's greater than the torque arising from the frictious force keeping it on the plane.

In any case, due to the constraint of having to roll without slipping, those two angles do not form a set of independent degrees of freedom. They are connected by a non-holonomic equation of constraint that involves their associated angular velocities and therefore you cannot set up Lagrangian equations of motion involving them. Trading one angle for the coordinates of the center of mass for example does not change the situation in any way, as these coordinates are still connected through the same kind of constraint.