Particles connected with spring, sliding over lines. Question about the potential energy

Question

I'm currently studying small oscillations with the Lagrangian formalism. I stumbled upon an exercise that I can't seem to understand the method of solving it.

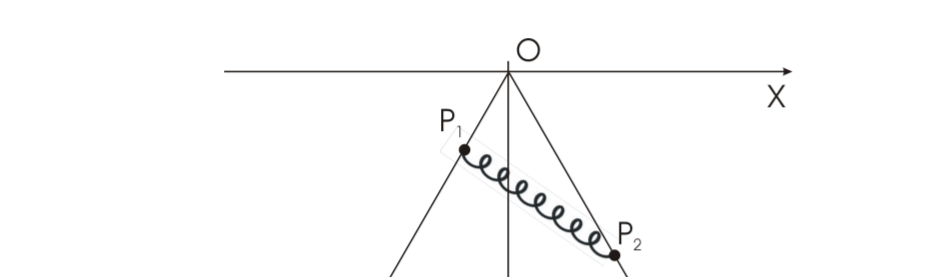
Two particles $P_1$ and $P_2$, with the same mass $m$, slide over two smooth, frictionless lines (see the figure). The two lines intersect each other at an angle of $60$°. The particles experience gravity and are connected by an ideal spring with natural length $l$ and a spring constant $k = \frac{\sqrt{3} mg}{l} $.

a) Prove that the equillibrium position of the system is given by $ |OP_1 | = |OP_2| = 2l $

b) Show that this equillibrium is stable and show that the frequencies of the small oscillations along the equillibrium position are given by $\omega_1 = \sqrt{\frac{k}{2m}}$ and $\omega_2 = \sqrt{\frac{3k}{4m}}$

To find the equillibrium position I calculated the potential energy $V$, both gravity and the elastic forces acting on the particle due to the spring contribute to the potential. I started by choosing the movement along both lines as generalised coordinates. $p_1$ and $p_2$ as the movement of particle $P_1$ and $P_2$ respectively.

The potential energy due to gravity is given by

$$ V_g(y_1, y_2) = -mg y_1 - mgy_2 $$ $$ V_g(p_1, p_2) = -mg p_1 \cos(30°) - mg (p_2 \cos(30°)) $$ $$ V_g(p_1, p_2) = -\frac{\sqrt{3}}{2} mg ( p_1 + p_2 ) $$

The potential elastic energy is given by

$$ V_e(p_1, p_2) = \frac{1}{2}k (L-l)^2 $$

Where I denoted the total length with $L$ and the natural (rest) length as $l$. With the cosine law, the abive can be written as

$$ L = \sqrt{p_1^2 + p_2^2 - 2p_1p_2 \cos(60°)} $$

$$ L = \sqrt{p_1^2 + p_2^2 - p_1p_2 } $$

Such that the elastic potential energy can be written as:

$$ V (p_1, p_2) = V_g (p_1, p_2) + V_e (p_1, p_2) $$ $$ V(p_1, p_2) = -\frac{\sqrt{3}}{2} mg ( p_1 + p_2 ) + \frac{\sqrt{2}}{2l}mg \left( \sqrt{p_1^2 + p_2^2 - p_1p_2 } - l \right)^2 $$

I then differentiated this potential, but I didn't find the correct answer. Is the potential correct like this? How can you define it in systems like this, where you have to take the natural length in account

Answer 1

It looks like you wrote $\sqrt{2}$ instead of $\sqrt{3}$ (look back at the expression for the spring constant) in the coefficient of the second term of the potential. With this correction, we get $$ \begin{split} \frac{\partial V}{\partial q_1} &= \frac{\partial}{\partial q_1}\left[\frac{\sqrt{3}}{2}mg\left(\frac{1}{l}\left(\sqrt{q_1^2 + q_2^2 - q_1q_2} - l\right)^2-q_1-q_2\right)\right] \\ &= \frac{\sqrt{3}}{2}mg\left[\frac{1}{l}\frac{\left(\sqrt{q _1^2 + q_2^2 - q_1q_2} - l\right)\left(2q_1-q_2\right)}{\sqrt{q_1^2 + q_2^2 - q_1q_2}} - 1\right]. \end{split} $$ (I've changed $p_1$ and $p_2$ to $q_1$ and $q_2$, respectively, because the $p$'s look like generalized momenta to me.) By symmetry, the equilibrium positions must be the same for both masses. So we can set $q_1 = q_2 = r_\text{eq}$. Setting the derivative of the potential to zero and simplifying, we find $$ \frac{(r_\text{eq}-l)r_\text{eq}}{lr_\text{eq}} - 1 = 0 \Rightarrow \boxed{r_\text{eq} = 2l}, $$ as desired.