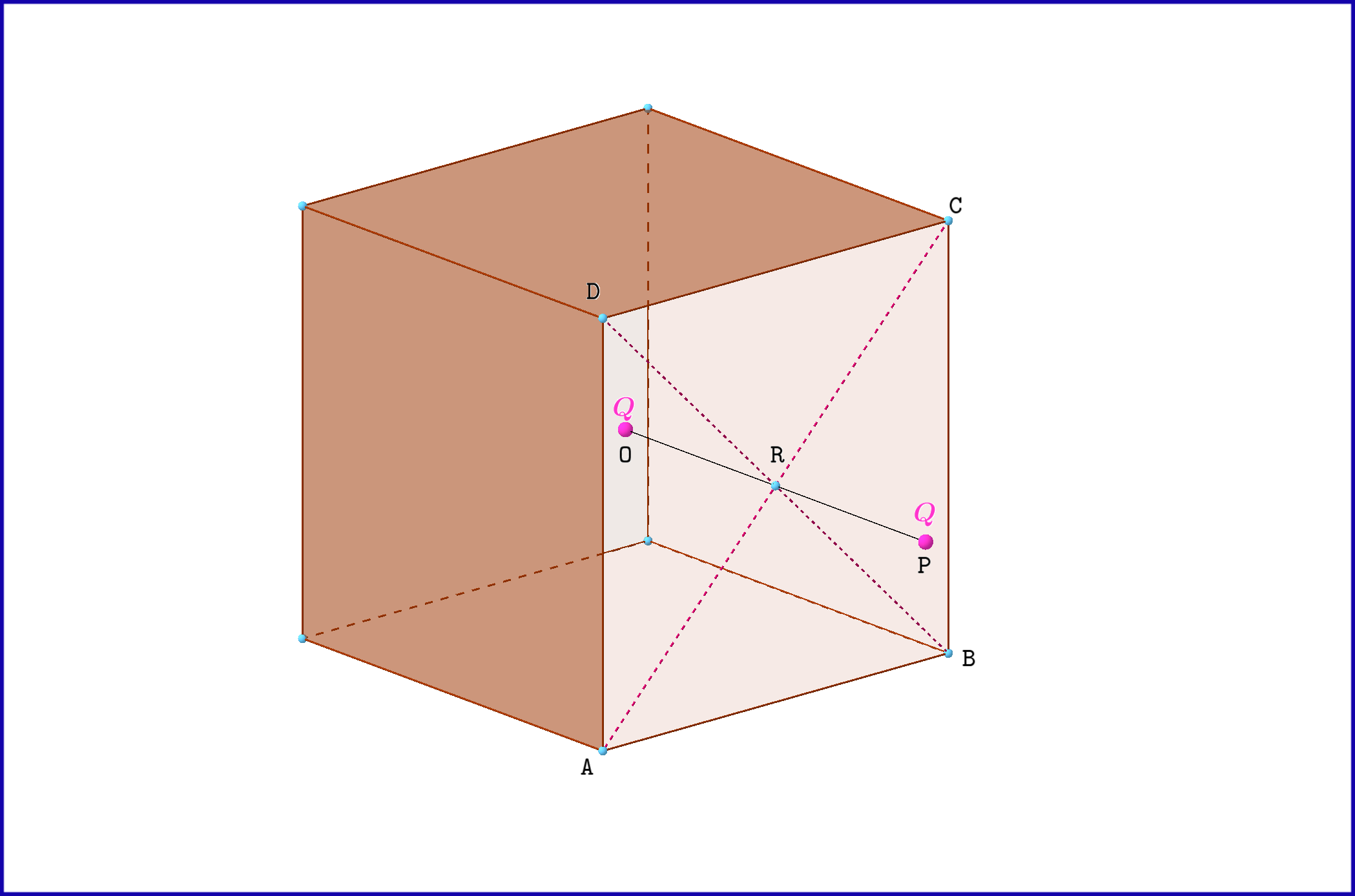
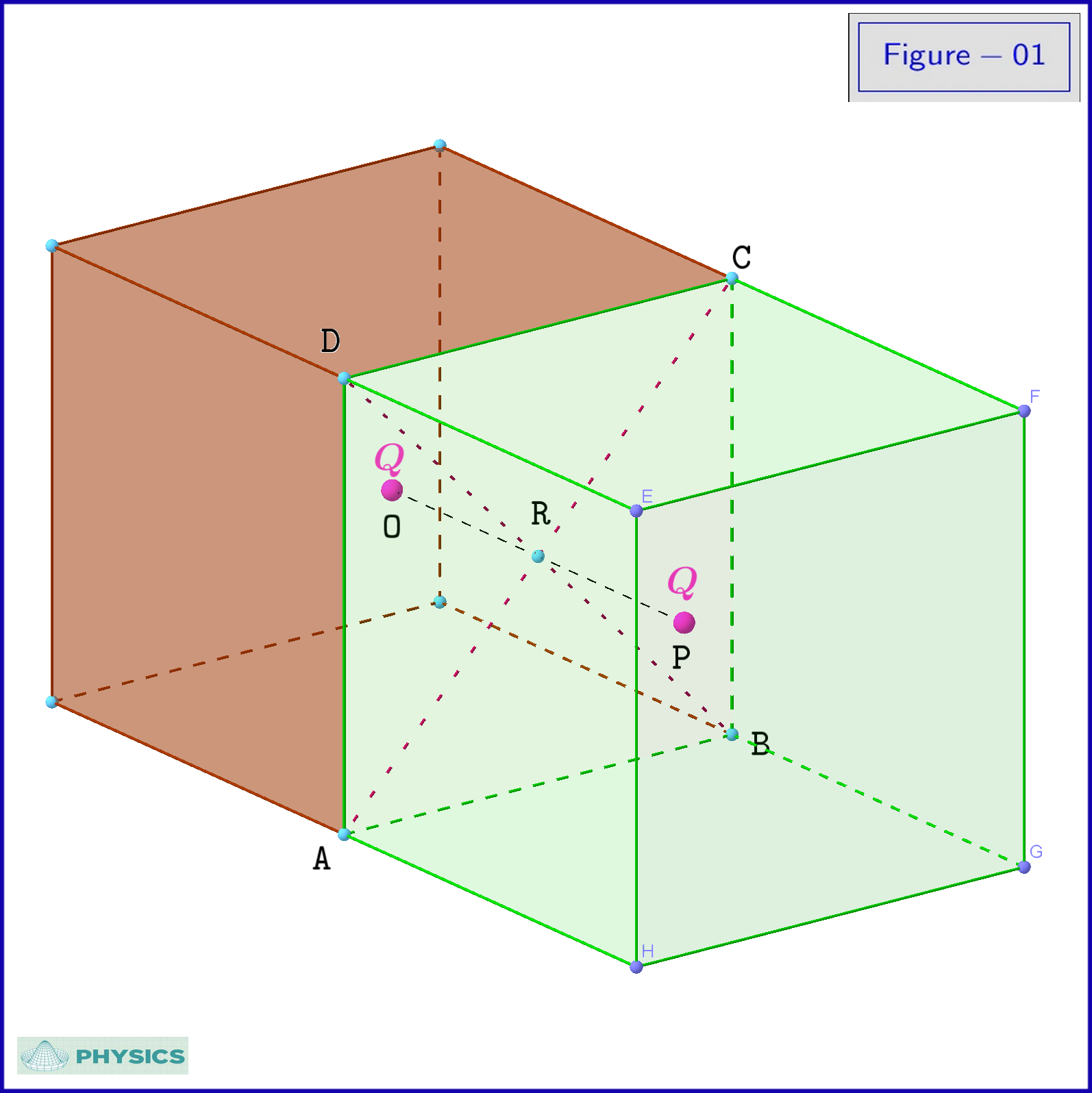
Consider a region in free space bounded by the surfaces of an imaginary cube having sides of length $a$ as shown in the Figure. A charge $+Q$ is placed at the centre $\mathrm O$ of the cube. $\mathrm P$ is such a point outside the cube that the line $\mathrm{OP}$ perpendicularly intersects the surface $\mathrm{ABCD}$ at $\mathrm R$ and also $\mathrm{OR}=\mathrm{RP}=a/2$. A charge $+Q$ is placed at point $\mathrm P$ also. What is the total electric flux through the five faces of the cube other than $\mathrm{ABCD}$?
My answer comes out to be $Q/\epsilon$. The solution is:
Flux due to inner charge only through fives faces $= 5q/6\epsilon$
Net flux due to outer charge $=0$ (Gauss's Law)
Flux due to outer charge only through one face $= q/6\epsilon$
Flux due to inner charge only through fives faces $=q/6\epsilon$ (what goes in must come out)$$\boxed{\text{Total flux} = \frac{5q}{6\epsilon} +\frac q{6\epsilon} =\frac q{\epsilon}}$$
My doubt is: Can we add the flux individually assuming no charge was present apart from charge under consideration?
Is my solution right or any improvements are required? Is there another way using calculus to prove that flux through each surface of cube due to point charge at center is $q/6\epsilon$?