Will the center of mass of this system move in the vertical direction?

Question

Information provided:

The mass $M_1$ slides down the mass $M_2$; ignore friction for the problem. Also initially the block $M_1$ was at rest.

Now as told by my friend the center of mass will change because in the two block system, one is coming down and other has no vertical movement, but my question is as all the $F_{\text{ext}}$ are balanced along the vertical direction thus $a(\text{c.o.m})$ must be zero along the vertical and thus the momentum is conserved along the vertical direction, because if the momentum is not conserved along the vertical then it means that $F_{\text{ext}}$ is unbalanced, but if you draw all the external forces on the system of 2 blocks then you can say that they are balanced. Now my friend says that the in the vertical direction the center of mass is not stationary and thus the momentum is not conserved for the system in the vertical direction, I am confused please help.

Answer 1

The COM of the two body system would move down, because this statement

"as all the $F_{\text{ext}}$ are balanced along the vertical direction..."

is false.

There is the external force of gravity acting on the small mass - that external force isn't balanced unless you include the earth.

The earth pulls the small mass down and the small mass pulls the earth up, with equal magnitude. So for the three body system the COM would stay in the same position.

For the two body system alone, momentum is not conserved, we can clearly see an increase in downward momentum with time. However momentum would be conserved for the full three body system.

Answer 2

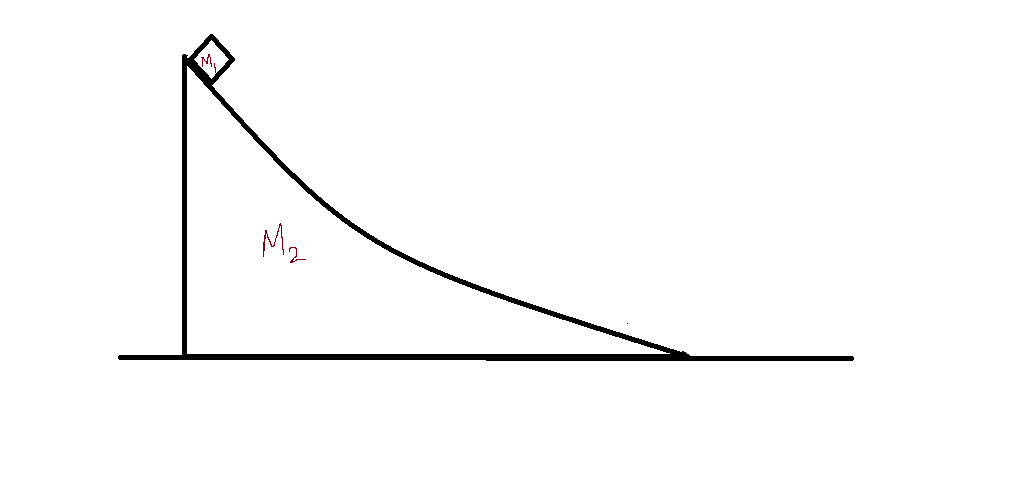
Clearly, at the end, the small block will be at the bottom whereas now is on the top so yes, the center of mass has shifted to the bottom as there now is more mass on the ground than on the top of the slide.

I hope this is intuitively clear: when the block gets to the bottom, the CoM has moved.

Why? Because mechanics impose that the acceleration $\vec{a}$ of the CoM times the total mass of the system $M=M_1+M_2$ (in this case) is equal to the sum of all external forces.

The only external forces acting on this system are gravity ($-M_1g$ for the big mass and $-M_2g$ for the small mass, towards the bottom, hence the minus sign) and the normal force acting from the ground $N$. There is also a normal force between the two masses, but that is an internal force.

So the total external force acting is $$F_{ext}=N-(M_1+M_2)g$$

So there are external forces acting hence the CoM can in principle move with the general equation

$$(M_1+M_2)a_v = F_{ext}=N-(M_1+M_2)g$$

where $a_v$ is the vertical component of the acceleration.

A more precise computation to find $N$ can be made of course, and shows that the sum is such that $F_{ext}\ne 0$. The key is in the fact that not all forces are balanced: the normal is not balancing gravity because the block on top is on a titled incline so it is not "pushing the ground" with its full weight. To be more precise

$$N=(M_2+M_1 \cos(\theta))g$$ where $\theta$ is the angle of the incline so that

$$(M_1+M_2)a_v = F_{ext}=N-(M_1+M_2)g =(M_2+M_1 \cos(\theta))g -(M_1+M_2)g = M_1g(cos(\theta)-1)$$

which indicates that the CoM is moving in the vertical direction with constant acceleration (until it reaches the bottom).

Notice that

1. on the other hand, in the horizontal acceleration there are no external forces, so the CoM does not move in that direction. As the small block goes down, the big one moves to the left.

2. if there was no ground (e.g. the two blocks are in the void) or if you include the ground in the system as a very big ($M\rightarrow \infty$) body, the result would change of course. In the latter case, the CoM would not move.

Answer 3

I take it that M2 is stationary and there is a uniform gravitational field.

In this case, the mass M1 will move downwards and hence the center of mass of the system will move downwards. The logic is:

• There is a force of gravity downwards.

• M1 cannot pass through M2, so there is a force normal to the surface of M2 to prevent this.

• Since the normal direction is not vertical, the net force is nonzero in a direction parallel to the surface of M2.

• Hence, there is nonzero acceleration along the surface of M2, and the horizontal and vertical components of the center of mass will move.