Question about the direction of Centrifugal and coriolis force

Question

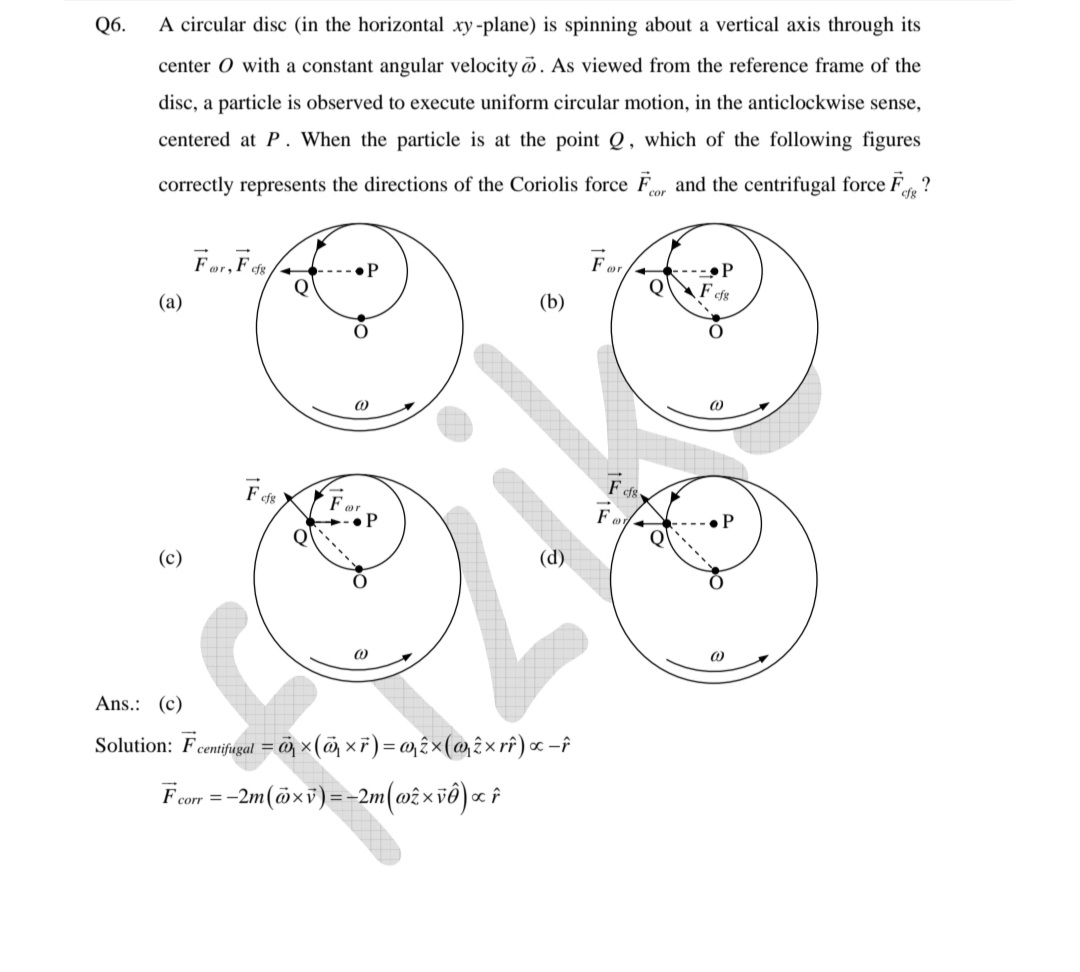
I can't understand how the cross product of $\hat{\mathbf{z}}$ and $\hat{\mathbf{r}}$ works in the equation of centrifugal force and the same cross product between $\hat{\mathbf{z}}$ and $\hat{\boldsymbol{\theta}}$ in the equation for Coriolis force. Please help me understand this.

According to my understanding, the cross product between $\hat{\mathbf{z}}$ and $\hat{\boldsymbol{\theta}}$ is along the direction that is completely opposite to the direction that is given here, i.e. rotate the Coriolis vector ($\mathbf{F}$) given in the answer by 180°. I can't even begin to comprehend how the direction of centrifugal force is found here.

Answer 1

This question is completely screwed up, and the answer is very wrong.

1. Centrifugal force is given by $ {\displaystyle -m{\boldsymbol {\omega }}\times ({\boldsymbol {\omega }}\times {\boldsymbol {r}})}$. There is a minus sign missing in the solution.
2. There are two simultaneous rotations in the problem, meaning the total centrifugal force is a vector sum of both. This can be directly visualized by noting the fact that for each circular motion the centrifugal force is radially outward and taking a vector sum of those two.
3. The Coriolis force is given by ${\displaystyle -2m{\boldsymbol {\omega }}\times \left[\operatorname {d} {\boldsymbol {r}}/\operatorname {d} t\right]}$, directed along $-\hat{z}\times\hat{v}$, where v is the velocity vector of the particle. $-\hat{z}\times\hat{v}=\hat{r}_{small}$, where $r_{small}$ is the coordinates centered at the smaller circle.