Feynman diagrams for neutron-neutron scattering

Question

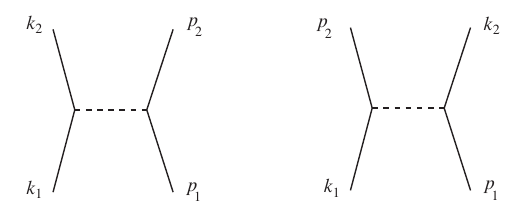
Neutron-neutron scattering can be described using the phenomenological Lagrangian $$\mathcal L_I=g \pi^0(x)\bar\psi_n(x)\gamma^5\psi_n(x). $$ Since the Lagrangian contains three fields and the process $n+n\to n+n$ involves four particles (let $p_1,k_1$ and $p_2,k_2$ be the momenta of the 'in' and 'out' pairs respectively), the terms in $g^2$ are the lowest that contribute to the scattering amplitude: the corresponding Feynman diagrams are

However, I have a small doubt. Why is there no diagram with $p_1$ and $k_1$ entering the same internal vertex?

Answer 1

Your two diagrams are the t-channel and u-channel interactions. (Note that, in your spacetime diagrams, the time axis points up, but in the examples at the links, the time axis points to the right.)

There is no s-channel scattering between neutrons because it would require the intermediate steps $\rm nn\to\pi^0$ and $\pi^0 \to \rm nn$, which violate baryon number conservation.