Why don't things rotate when they fall?

Question

One of the answers to this question: How does the center of gravity work? said the follows:

Since we can see that things do not start to rotate when they fall, we must assume gravity when averaged out to be pulling in the point where all torque cancel each other out, which is the center of gravity.

Also in some answers to that question, I learned that gravity isn't applied only at the center of gravity in real life, but rather every single particle of an object. Thus, I am confused why things do not start to rotate when they fall in real life as the torque caused by gravity doesn't cancel out?

Answer 1

The statement, "Objects don't rotate when they fall" is false.

I know this as an experimental fact b/c I worked on SRTM (https://www2.jpl.nasa.gov/srtm/). Consider the object in the figure:

We can approximate this as an asymmetric dumbbell of length $L=60\,$m and two masses, $M=2\,$Gg (the space shuttle), and $m=20\,$Mg (the outboard radar antenna). The system is a side-looking interferometric synthetic aperture radar (InSAR) with 2 antenna forming a baseline that is perpendicular to the radar wave vector...which means it needs to sit at 45 degrees off-horizontal.

That configuration is unstable. It wants to rotate as the space shuttle orbits [read: falls]. A nitrogen cylinder was rigged to a nozzle on the outboard antenna to apply a smooth torque to stabilize the position; however, it froze in space, and the mission used the shuttle's attitude thrusters (not smooth). (The ensuing oscillations in the antenna arm added 1 year to the data processing, as the relative positions of the two antenna phase centers needs to be know to a small fraction of the radar wavelength, which was 5.6 cm).

The Earth's gravity field is not uniform. It's given by the potential:

$$ V(r)=-\frac{GM_E} r $$

which can be Taylor expanded at LEO ($R=6611137.0\,$m):

$$ V(R+\delta r) = V(R)\Big[1-\frac{\delta r} R +\big(\frac{\delta r} R\big)^2 \Big]$$

The lever arms of the two masses relative to the C-O-M are:

$$ L_M = L\frac m {M+m} $$ $$ L_m = L\frac M {M+m} $$

If you plug that into the Taylor expansion and look at the change in potential energy relative to $\theta=0$ (horizontal), the 0th doesn't contribute, and 1st order terms cancel:

$$\Delta U(\theta) = \frac{V(R)}{R^2}\frac{m^2M+M^2m}{M+m}$$

The result is shown in the figure:

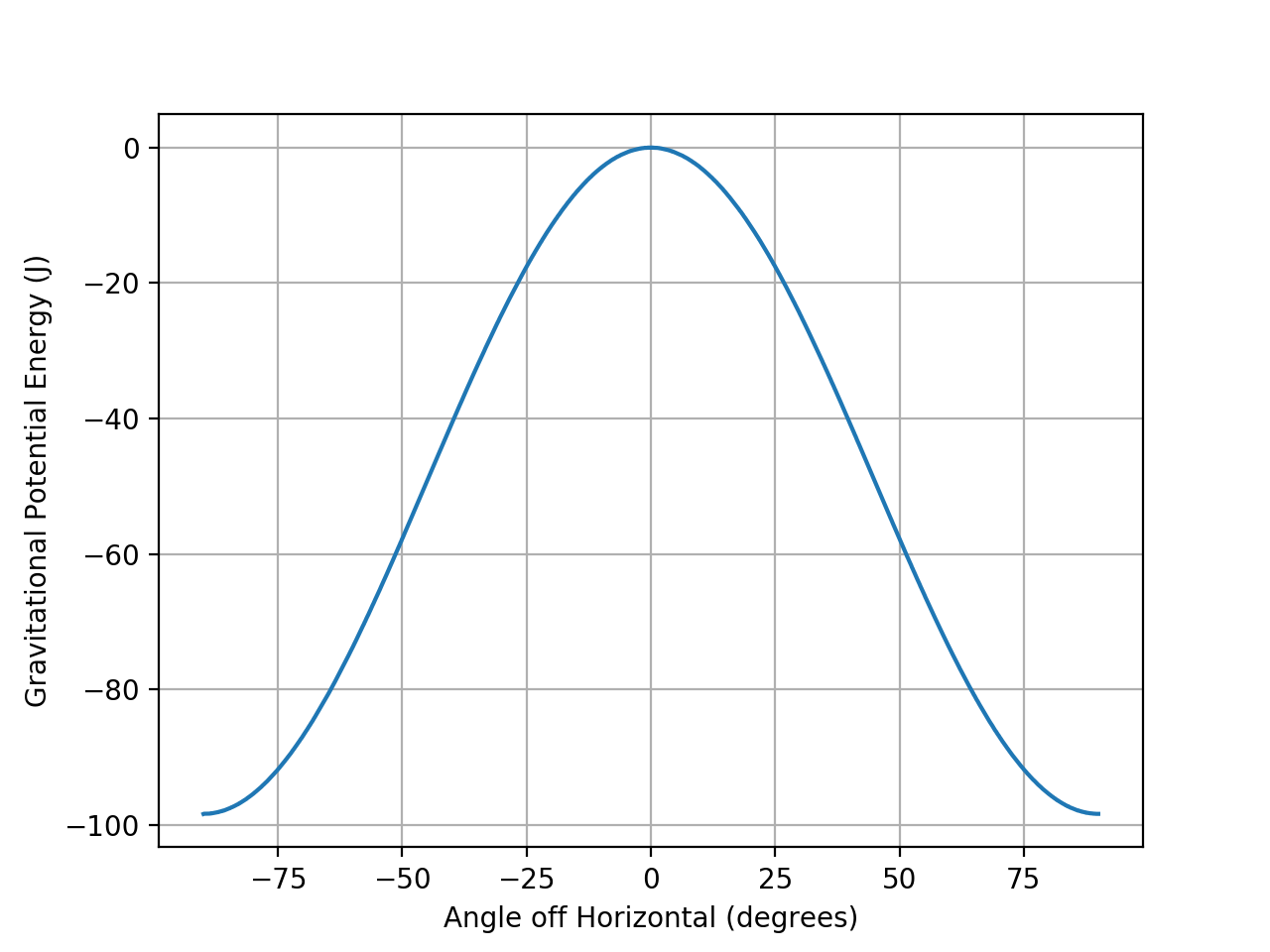
So horizontal is an unstable equilibrium. The system wants to be aligned vertically, and it doesn't matter which mass is higher or lower. The 180 degree symmetry is characteristic of quadrupole moments. [This is a quadrupole moment coupling (hence the mass-squared terms) to a tidal tensor (2nd order potential derivative)].

Note that the magnitude, a hundred Joules, is tiny compared with the many TJ of total potential energy; nevertheless, the torque was enough to require thruster corrections every 20 minutes.

In general, forces and torques are caused by energy gradients. For an arbitrary distribution in an arbitrary conservative field, the various terms that contribute to energy are approximated by a multipole expansions. The zeroth order term in gravity is mass times potential:

$$ m\times -\big(\frac{GM} r)\big) \rightarrow m\times(gh) $$

In EM, a dipole couples to the field:

$$ \vec q \cdot \vec E = \vec q \cdot -\nabla \Phi $$

Note that a dipole moment is a 1st moment of charge, which couples to the gradient of the field. Since gravity is strictly attractive, this term must cancel by symmetry. (There are no gravitational dipoles).

The SRTM example is the quadrupole moment of a mass distribution (a 2nd moment), coupling to the tidal tensor:

$$ Q^{ij}\Phi_{ij} $$

The tidal tensor is a 2nd derivate with isotropic terms removed:

$$ \Phi_{ij} = J_{ij}-\frac 1 3 {\rm Tr}(J) $$

there $J$ is the 2nd derivative tensor of the potential:

$$ J_{ij} = \frac{\partial^2 \Phi(r)}{\partial x_i\partial x_j} $$

On can continue this to higher moments, with a rank-3 octupole tensor coupling to a suitable gradient of the tide (a la $\nabla_i\Phi_{jk}$), and so on, but that is not common. By the time fields and their gradients are strong enough that 3rd derivatives matter, you're into General Relativity and different techniques are used.

Answer 2

A gravitational field affects every bit of matter equally - for any object on earth, the acceleration due to gravity is 9.8 $m/s^2$. When an object is at rest, there is clearly no relative movement between any parts of the object, which would be observed as a bending, stretching, or rotation of the object. When you drop that object, every bit of it is accelerated due to gravity in the exact same way. The object as a whole is now moving, but since every atom was accelerated the exact same amount, there is still no relative movement between any parts of the object. The net torque is zero even when accounting for the force of gravity acting on every part of the object.

This answer assumes a uniform gravitational field, which is never exactly true but is a very good approximation when on the surface of the earth. A non-uniform gravitational field, (such as one experienced by a very long object near a black hole, or by the moon in earth's gravitational gradient for example) can induce a rotation as different parts of an object get accelerated differently, resulting in stretching, bending, or spinning. Tidal locking is an example of this phenomenon, in which a celestial body induces a rotation in another through the force of gravity alone.

Answer 3

Look into tidal locking.

Ask yourself why the same side of the moon always faces earth. In your search for an answer, you will come to understand how gravity is applied to every material point of a body. In some cases, this can in fact cause a gravitational torque.