1
$\begingroup$

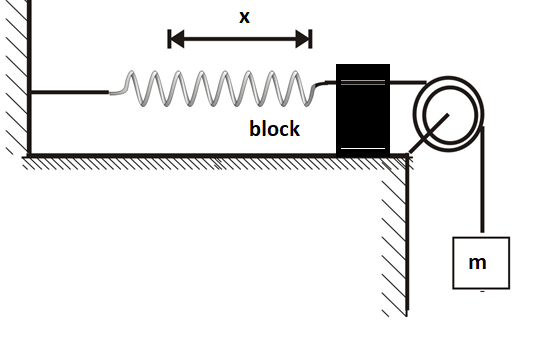
enter image description here

I am doing to a practical and having a hard time understanding the physics behind the actual response. A block is attached to the spring and mass is attached at the end of the spring. There is friction in the table and block. During the loading cycle, mass is increased gradually (say 100 grams) till the spring reaches the required extension. During the unloading cycle, it is observed that the block does not move until a specific amount of weight is removed (say 300 grams). Afterward, the spring moves with every 100 grams. Due to this reason, if I note the displacement x, half the way, there is a difference due to the initial response of the spring. I am trying to understand the physics behind the problem but failed to understand why there is no initial movement after I remove the 1st 100 gram. If its the static friction causing the initial delay, then why it does not happen afterward too. I would really appreciate some insight as I am stuck.

Improve this question
$\endgroup$
3
  • $\begingroup$ Static friction (non-moving) is higher than dynamic friction (while-slipping). When it stops slipping, static friction again is in control. $\endgroup$
    – Jim Clark
    Commented Jul 14, 2021 at 13:53
  • $\begingroup$ Thank you for the response. you are absolutely right. the thing that confused me though was why the system did not respond immediately to the weight removal and why it did not happen consistently. $\endgroup$
    – rayan
    Commented Jul 14, 2021 at 13:57
  • $\begingroup$ This is an example of hysteresis - see en.wikipedia.org/wiki/Hysteresis. $\endgroup$
    – gandalf61
    Commented Jul 14, 2021 at 14:26

1 Answer 1

Reset to default
1
$\begingroup$

Static frictional forces are equal and opposite to the net force on the block, up to a certain limit. The block only starts moving when the net force on the block is above the friction limit.

At the start, the forces on the block are in equilibrium: the force exerted by the mass is equal and opposite to the force of the spring, and so there is no net force on the block.

After removing the first 100 gram from the weight, the spring force is now larger than the force of the weight, and so there is a net force on the block to the left. But that net force is still below the limiting frictional force, and so the block does not move.

After removing enough weight, the net force will rise above the frictional limit and the block moves. But the block moving will shorten the spring, and therefore reduce the force of the spring. The block will stop moving as soon as the spring force is equal to the frictional force plus the weight. The net force on the block in that situation is equal to the limiting frictional force (or actually a little less, because a moving block has less friction than a stationary one).

So this situation is not equal to the initial situation, where there was zero net force on the block. The block will immediately start moving again when you remove more weight, because the net force will immediately rise above the limit again.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Thank you very much for the detailed response. So if I understand correctly, the distance traveled by the block during the unloading cycle (when weights are removed) would always be less than the distance traveled during the loading cycle (when weights are added). On a side note, should I try to tackle the problem by having force equilibrium, a free body diagram of the system at the initial and final position or by doing conservation of energy to determine the travel 'x' of the block? $\endgroup$
    – rayan
    Commented Jul 14, 2021 at 13:51
  • $\begingroup$ @rayan Before the loading you start with a block with no horizontal forces on it (I assume), and after the unloading, there is a spring force balanced by the frictional force. So yes, it will not return to the starting point after unloading. $\endgroup$
    – fishinear
    Commented Jul 14, 2021 at 13:58
  • $\begingroup$ @rayan Free body diagrams of the system just before a move, and just after a move seems most applicable. Conservation of energy is not going to help you, because the friction is converting kinetic energy into thermal energy. $\endgroup$
    – fishinear
    Commented Jul 14, 2021 at 14:01
  • $\begingroup$ Thank you very much. Could you also give a tip on solving with force equilibrium vs energy balance for such a system as mentioned in my previous comment? $\endgroup$
    – rayan
    Commented Jul 14, 2021 at 14:01
  • $\begingroup$ bundle of thanks $\endgroup$
    – rayan
    Commented Jul 14, 2021 at 14:14

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.