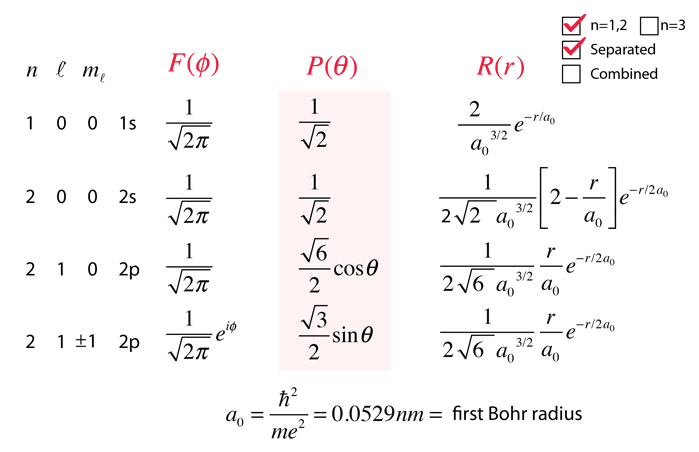In the quantum model of atom, an electron has a probability of being found anywhere in the space (except nodes). Now suppose a first shell electron ($n=1$) is present at a distance $r1$ from the nucleus and has energy $E$. Now if the next instant it is present at a new distance $r2$ from nucleus, will the energy change or remain the same? If no, then why? If yes, then how can we say that principal quantum number determines the energy of the electron? What would be the physical meaning of shell then?
$\begingroup$
$\endgroup$
Add a comment
|
4 Answers
$\begingroup$
$\endgroup$
2
"a first shell electron (n=1) is present at a distance $r_1$ from the nucleus and has energy $E$."
Quantum-mechanically this is impossible. The Hamilton-operator does not commute with the position operator. So having an electron at energy $E$ and at a known position $r_1$ is impossible.
So of course you could measure the position. But if you do so, the electron will be in a pure position state. However, then the information on the energy state is completely lost. The position state can be decomposed in a series of energy states, and nobody would know in which of these energy states the electron would be. So you can measure the energy state again, but in the very same moment you loose the information of the electron's position ...
This said, it is impossible to know position and energy of an electron at the same time and all speculations about this are make no sense in Quantum mechanics.
answered Jun 28, 2021 at 10:22
-
$\begingroup$ okay....but first tell me what constitutes the total energy of an electron in a QM model $\endgroup$ Commented Jun 28, 2021 at 12:06
-
$\begingroup$ @user95732 Well, this depends on the circumstances, for instance the potential (harmonic oscillator potential, free electron (no potential), H-atom, He-atom etc). In a H-atom it is $E_n = - \frac{m e^4}{2 (4\pi \epsilon_0)^2 \hbar^2 n^2}$ in SI-units. $n$ is the principal quantum number $n\in \mathbf{N}$ $\endgroup$ Commented Jun 28, 2021 at 12:48
$\begingroup$
$\endgroup$
11
Here are the hydrogen wave function solutions in space, for (r,θ,φ)
and further in the link.
The energy of the electron is basically given by the level of the n quantum number (with some corrections for the spin state), In the simple Bohr model the values are given here, which do not differ from the correct quantum mechanical solution. So the electron in its orbital ( not orbit) has a probability given by $Ψ^*Ψ$ to be at a particular (r,θ,φ) if measured.
Now suppose a first shell electron (n=1) is present at a distance 'r1' from the nucleus and has energy E. Now if the next instant it is present at a new distance 'r2' from nucleus, will the energy change or remain the same?
The wavefunction gives only the possibility of calculating probabilities, it is not tied like a quantum number on the electron, that is the misunderstanding. It does not describe where the electron is in space, but just the probability of finding the electron at (r,θ,φ) if measured at an arbitrary time. The energy is always the one characterized by the n quamtum number. It does not change.
To get an idea, look how the orbitals available for electrons in the hydrogen solution look.
-
$\begingroup$ But how could the energy remain same if the electron is closer to nucleus? Shouldn't the electrical and gravitational potential energy decrease.....and thus the overall energy? $\endgroup$ Commented Jun 28, 2021 at 12:03
-
2$\begingroup$ @user95732 that is why quantum mechanics had to be invented, to explain the spectra ( transition energies )of atoms which cannot be explained with the classical model you have in mind. $\endgroup$– anna vCommented Jun 28, 2021 at 12:08
-
$\begingroup$ @user95732 It's time to abandon the notion that the electron exists at some location before measurement. It does not. It's not like the electron is moving around and we just have no idea where it is. Quantum mechanical particles do not have trajectories $\endgroup$ Commented Jun 28, 2021 at 12:16
-
$\begingroup$ but why does the electron have energy in the first place? isn't it potential energy anc kinetic energy?? $\endgroup$ Commented Jun 28, 2021 at 12:24
-
2$\begingroup$ @annav Part of studying is asking questions. I think it is appropriate to say that the OP needs build a better foundation of QM understanding; I don't think it helps to discourage them from asking questions though. $\endgroup$ Commented Jun 28, 2021 at 13:53
$\begingroup$
$\endgroup$
In a classical framework, the total energy of the electron is its potential energy plus its kinetic energy (although to be precise, the electric potential energy is a property of the entire system, not just the electron). So in the classical model, an electron that's close to the nucleus would have higher velocity than one farther from the nucleus. This only partly translates to the quantum picture, but there is some correspondence for the correctly formulated quantities.
$\begingroup$
$\endgroup$
The way I see it you have the other way around, which is the source of your confusion.
From a quantum mechanical standpoint the possible energies are the eigenvalues of the Hamiltonian. There really is no other way to look at it. The Hamiltonian is dependent on space is the potential and possibly via interactions.
Thus energies are a functional of space dependent functions, but not coordinate dependent explicitly.
That being said, the converse is true. I.e. the probability of measuring a particle at a certain position depends on is energy. From a probability point of view the energy and position of a particle are two dependent random variables, drawn from separate distributions.
