Is this current direction represented correctly?

Question

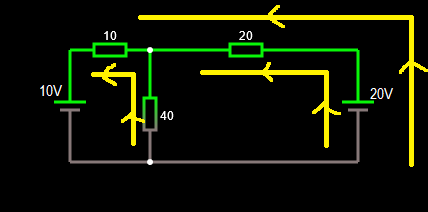
I was reading about Kirchhoff's Circuit Law and came across the following diagram:

Direction of the loop 1 and loop 2 makes sense to me as current (convention) is flowing from positive to negative. But it seems to me that the 3rd loop's direction is opposite to that of the 2nd one. Once $I_1$ splits at node A it will be at the opposite direction of $I_2$, what happens then?

I went to Falstad and drew the same diagram to make a simulation, but the result confused me even more, here is the current flow I got:

This even contradicts the flow in the first image all current is going from positive to negative of the bigger voltage source and "ignoring" the $10\rm\ V$ one.

Falstad link for the animation

Any explanation is really appreciated!

Answer 1

As already pointed out in @JunSeo-He and @Dale answers, the direction of the current loops can be assigned arbitrarily, as long as you are consistent in applying the following:

1. In moving around the loop in the direction you assigned the current, the polarities of the components (resistors, inductors, capacitors) in the loop should be + to - entering and exiting each component in the direction of the loop current assigned.

2. In applying KVL to each loop, - to + is a voltage rise (given a positive value) and + to - a voltage drop (given a negative value) and the algebraic sum of the rises and drops has to equal zero.

3. Here's one that is sometimes overlooked or messed up. When treating each loop don't forget to include any voltage rise or drop contribution from another loop in a component that is common to both loops. For example, in loop 1, there is a there is a voltage drop in R3 due to loop current 1 but a voltage rise in loop 1 in R3 due to the current in loop 2.

If you are consistent in the above, you may get positive or negative values for the loop currents. A positive value for a loop current simply means you selected the direction correctly. A negative value means the direction of the current is opposite to what you assigned.

Hope this helps.

Answer 2

With some conventions the direction of the arrow label that you use for the current direction on a circuit diagram is defined but when using other conventions you have a free choice.
In your top diagram drawing the arrows for loops $1$ and $2$ is possibly making the assumption that the batteries will be discharging (chemical energy converted to electrical energy) and so current will be leaving from the positive terminals of the battery.

Adding a third loop adds redundancy to the problem in that you only need two current loops to solve this problem although those could have been loops $2$ and $3$ or loops $1$ and $3$.
There is nothing special about loop $3$ as you will note that each loop misses out part of the circuit and that is why you need a second loop.

Having set up the Kirchhoff's laws equations and solved for the two currents an important thing to note is the sign of the value of the currents.
If positive then your original choice of arrow label direction was correct in that the actual current flows in that direction.
If however the numerical value of the current is negative that means that the actual current direction is in the opposite direction to the arrow label direction.

Answer 3

When doing nodal analysis you pick the direction of the current randomly,you then apply KVL in each loop using Ohm's law and then you find the currents.

Answer 4

what happens then?

You get a negative number for the current.

The direction of the current is completely arbitrary. There is no wrong or right direction. When I solve such systems I never even pause for a moment to consider which direction the current might actually flow, I simply make all currents clockwise.

If you make a correct guess then you get a positive value for current. If you make an incorrect guess then you get a negative value for current. Negative numbers are perfectly valid so there is no penalty for guessing wrong and no bonus for guessing right. I adopted the always clockwise convention so that I could go faster and so that I reduced the chance of being inconsistent.

The only important thing is to make some guess and then be absolutely consistent with that guess in all of your math. In my opinion, the always clockwise rule helps with being consistent.