I have never understood what's the meaning of the sentence "rolling without slipping". Let me explain.
I'll give an example. Yesterday my mechanics professor introduced some concepts of rotational dynamics. When he came to talk about spinning wheels he said something like:
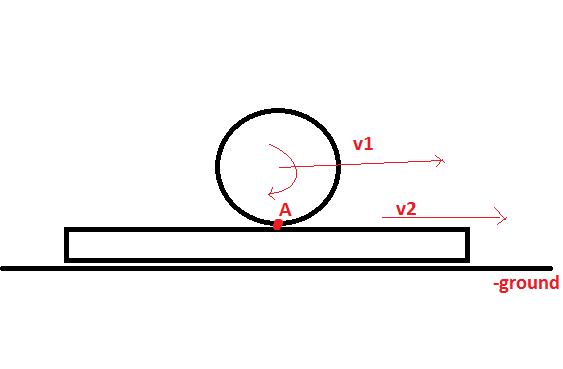
"If the wheel is rolling without slipping, what's the velocity of the point at the base of the wheel?? It is... zero! Convince yourself that the velocity must be zero. Since if it wasn't zero, the wheel wouldn't be rolling without slipping. So the wheel is rolling without slipping if and only if the point at the base has velocity zero, i.e. if and only if the tangential speed equals the speed of the center of mass."
Well, what I really don't understand is this: is the "rolling without slipping" condition defined as "Point at the base has zero speed"? If not, what's the proper definition for that kind of motion?
Looking across the internet, I have found more or less the same ideas expressed in the quotation. Furthermore, if it was a definition, then it would be totally unnecessary to say "convince yourself" and improper to talk about necessary and sufficient conditions.
I'd like to point out that I'm not really confused about the mathematics behind this or with the meaning of the condition above. What puzzles me is why are those explanations always phrased as if the condition $v'=0$ (where $v'$ is the relative velocity beetween the point at base and the surface) is some necessary and sufficient condition to be "rolling without slipping". Seems to me that this is exactly the definition of "rolling without slipping" and not an "iff".
Any help is appreciated, thanks.