You can calculate the torque with respect to any given point in any given coordinate system. In particular, you can calculate the torque relative to the center of mass with all vectors represented in the inertial coordinate system. Only because you choose some point to calculate the torque with respect to, doesn't mean you have to change the coordinate system and move it to that point. You just have to take cross products of vectors pointing from the chosen point to the points at which various forces are applied and add them together. The differential equation, you mentioned however, requires an inertial coordinate system. In an non-inertial one, you just have to correct it with the corresponding fictitious torques so something like this:
$$\text{Inertial: } \frac{d \vec{L}}{dt} \, = \, \vec{\tau}$$
$$\text{Non-inertial: } \frac{d \vec{L}}{dt} \, = \, \vec{\tau} \, + \, \vec{\tau}_{\text{fict}}$$
For example, in rigid body dynamics, in an inertial frame, you have
$$\frac{d \vec{L} }{dt} \, = \, \vec{\tau}$$
But in the frame attached firmly to the body and centered at the center of mass
$$\frac{d \vec{L}}{ dt} \, = \, \vec{\tau} \, + \, \vec{L} \times \vec{\omega}$$ where $\vec{\omega}$ is the angular velocity expressed in the body-fixed frame. $\vec{L}$ and $\vec{\tau}$ are also expressed in the body-fixed frame.
Your case: you have a cart with a stationary man on top of it, moving uniformly along a circle of radius $R$ with constant velocity of magnitude $v$.
Let us fix an inertial coordinate system, centered at the center of the circular arc formed by the train-tracks, $x$ and $y$ coordinate axes aligned with the ground and the $z$ axis perpendicular to it. Let $\vec{r} \, = \, \vec{r}(t)$ be the vector pointing from the origin of the system to the projection of the man's center of mass onto the cart (the cart follows the circular train-tracks in the $x, y$ horizontal plane). Then, because the cart movies uniformly along a circle,
$$\frac{d^2\vec{r}}{dt^2} \, = \, - \frac{v^2}{R^2} \vec{r}$$
The angular velocity of the cart in the inertial system, is $$\vec{\omega} \, = \, \frac{v}{R} \, \vec{e}_z$$ Since the man is motionless relative to the cart, i.e. he moves the same way the cart does, his angular velocity, relative to the inertial system, is equal to the cart's angular velocity $\omega$.
Consequently, the angular momentum of the man in the inertial coordinate system looks like $$\vec{L} \, = \, I \,\vec{\omega}\,= \, I_{z} \, \frac{v}{R} \, \vec{e}_z$$
where $I_z$ is the inertia moment of the man calculated along the vertical axis passing through his center of mass, which is always parallel to the $z$ axis of the inertial coordinate system, so $I_z$ is a constant. This particular configuration is special. Had the man been tilted somehow, so that his inertia axes were not as in the picture, then the equation would have needed an extra term for a time-changing angular momentum. But because of how the man stands on the cart, the angular momentum and the angular velocity are aligned (point in the same direction). This is because one axis of inertia of the man is aligned with the $z-$coordinate axis of the inertial coordinate system, while the other two inertia axes always rotate parallel to the $x,y-$coordinate plane of the inertial coordinate system.
The forces, in the inertial coordinate system, acting on the man on the cart are
$$N_1 \vec{e}_z, \,\,\, N_2 \vec{e}_z, \,\,\, - \, \frac{f_1}{R}\vec{r}, \,\,\, - \, \frac{f_2}{R}\vec{r}, \,\,\, -\,\vec{mg}\,\vec{e}_z$$
The vector $\vec{r}_G$ from the origin of the coordinate system to the man's mass center is
$$\vec{r}_G \, = \, \vec{r} \, + \, L \, \vec{e}_z$$
The vectors from the origin of the coordinate system to the feet of the man are
$$\vec{r}_1 \, =\, \left(1 + \frac{d}{2R}\right)\vec{r}$$
$$\vec{r}_2 \, =\, \left(1 - \frac{d}{2R}\right)\vec{r}$$
because $|\vec{r}| = R$ and so $\frac{\vec{r}}{R}$ is a unit vector, aligned with $\vec{r}$ at all times $t$. Hence
$$\vec{r}_i \, = \, \vec{r} \,\pm\, \frac{d}{2} \, \frac{\vec{r}}{R}$$
So the vectors from the center of mass of the man to his feet are
$$\vec{r}_1 - \vec{r}_G \, = \,\,\, \left(1 + \frac{d}{2R}\right)\vec{r} \, - \, \vec{r} \, - \, L \, \vec{e}_z \, = \, \frac{d}{2R}\,\vec{r} \, - \, L \, \vec{e}_z$$
$$\vec{r}_2 - \vec{r}_G \, = \, \left(1 - \frac{d}{2R}\right)\vec{r} \, - \, \vec{r} \, - \, L \, \vec{e}_z \, = \, -\, \frac{d}{2R}\,\vec{r} \, - \, L \, \vec{e}_z$$
Now, let's calculate the torques:
$$(\vec{r}_1 - \vec{r}_G) \times ( \,N_1\,\vec{e}_z \,) \, = \, \frac{N_1d}{2R} \,\vec{r} \times \vec{e}_z$$
$$(\vec{r}_2 - \vec{r}_G) \times ( \,N_2\,\vec{e}_z \,) \, = \, - \, \frac{N_2d}{2R} \,\vec{r} \times \vec{e}_z$$
$$(\vec{r}_1 - \vec{r}_G) \times \left( \, - \, \frac{f_1}{R}\vec{r} \,\right) \, = \, \frac{f_1L}{R} \,\vec{e}_z \times \vec{r}$$
$$(\vec{r}_2 - \vec{r}_G) \times \left( \, - \, \frac{f_2}{R}\vec{r} \,\right) \, = \, \frac{f_2L}{R} \,\vec{e}_z \times \vec{r}$$
The torque of gravity with respect to center of mass is zero.
So the vector equation of motion for angular momentum/angular velocity is
$$\frac{d}{dt} \vec{L} \, = \,\frac{d}{dt}\left( I_{z} \, \frac{v}{R} \, \vec{e}_z\right) \,= \, \frac{N_1d}{2R} \,\vec{r} \times \vec{e}_z \, - \, \frac{N_2d}{2R} \,\vec{r} \times \vec{e}_z \,+\,\frac{f_1L}{R} \,\vec{e}_z \times \vec{r} \, +\, \frac{f_2L}{R} \,\vec{e}_z \times \vec{r}$$
However, since $\frac{v}{R}$ is a constant,
$$\frac{d}{dt}\left( I_{z} \, \frac{v^2}{R} \, \vec{e}_z\right) \,= \, 0 \, = \, \left(\,- \, \frac{N_1d}{2R} \, + \, \frac{N_2d}{2R} \,+\,\frac{f_1L}{R} \, +\, \frac{f_2L}{R} \,\right) \, \vec{e}_z \times \vec{r}$$
which yields
$$- \, \frac{N_1d}{2R} \, + \, \frac{N_2d}{2R} \,+\,\frac{f_1L}{R} \, +\, \frac{f_2L}{R} \, = \, 0$$ and if you multiply both sides of the latter identity by $R$, you get exactly
$$- \, \frac{N_1d}{2} \, + \, \frac{N_2d}{2} \,+\, ({f_1} \, +\, {f_2})\,L \, = \, 0$$
So I guess you have some reason to be unsatisfied with the textbook's explanation, because they were sloppy. The total torque would not have been zero, had the motion been accelerating along the circle or some more general motion of the cart. The only reason the latter equation holds is because the angular velocity and the angular momentum are constant vectors for this specific uniform motion along a circle!
Maybe If you would like, you can read this answer of mine, where I try to derive rigorously the mathematical modelling behind the motion of a rigid body and how that motion can be decomposed into translational motion of the center of mass plus rotation around the center of mass.
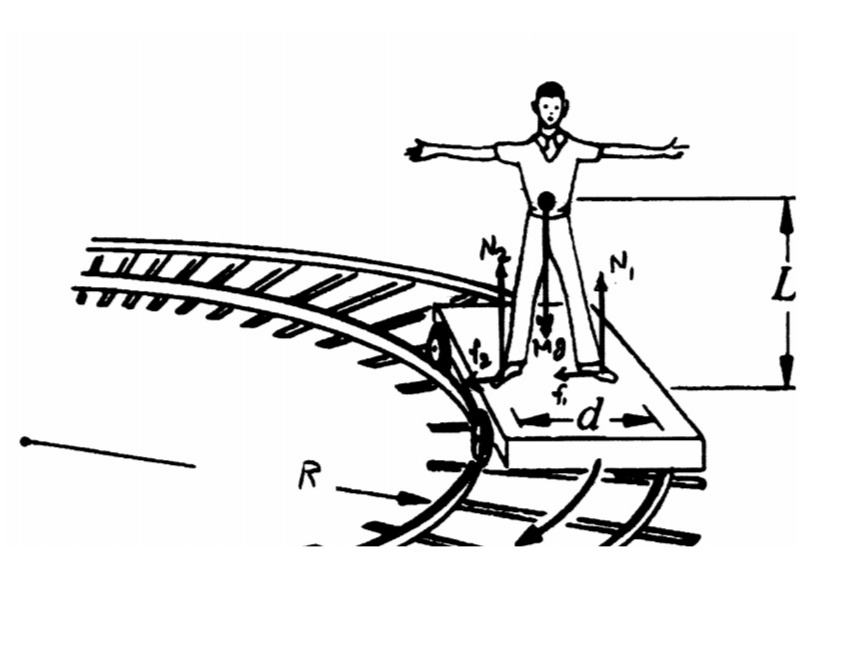 Statement:
A Man of mass M stands on a railroad car that is rounding an unbanked turn of radius R at speed v. His center of mass is height L above the car, and his feet are distance d apart. The man is facing the direction of motion.
Statement:
A Man of mass M stands on a railroad car that is rounding an unbanked turn of radius R at speed v. His center of mass is height L above the car, and his feet are distance d apart. The man is facing the direction of motion.