Conductivity of an Ideal Gas

Question

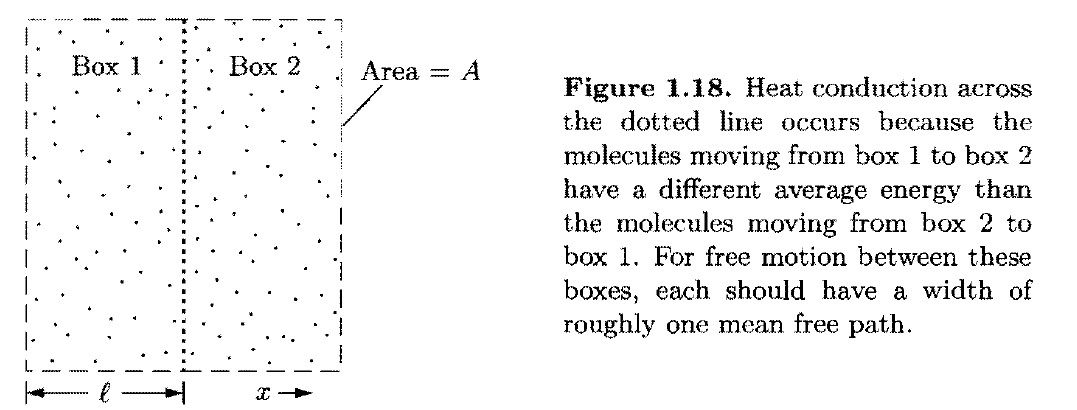
I'm currently going through the derivation of heat flow equation of an ideal gas in the An Introduction to Thermal Physics book by Schroeder and had some trouble understanding it. It uses the setup below:

The diagram above represents a small region within a container of gas. The temperature increases in the positive $x$ direction but does not vary in the vertical direction. $l$ is the mean free path of the molecules in the two boxes. $\Delta t=l/v_{rms}$ is the average time between collisions where $v_{rms}$ is the RMS velocity of all the molecules in the two boxes. The two boxes each have a length of $l$. $U_{1}$ is the total internal energy of particles in Box 1 and $U_2$ is the total internal energy in Box 2. The boxes are intended to be imaginary separations and not actual physical separations.

During the time interval $\Delta t$, the book states that the energy crossing the dotted line from Box 1 is equal to $U_{1}/2$ since half of the particles in Box 1 will have a positive velocity in the $x$ direction. In other words, half of the molecules in Box 1 will cross the dotted line during $\Delta t$. By the same logic, the energy crossing the dotted line from Box 2 to the left is equal to $U_{2}/2$. Then, the net heat energy $Q$ that crosses the dotted line is: $$Q = \frac{1}{2} (U_1-U_2) = -\frac{1}{2} (U_2-U_1) = -\frac{1}{2} C_V (T_2-T_1) = -\frac{1}{2}C_V l \frac{\text{d}T}{\text{d}x}$$

But my problem with this derivation is that $\Delta t$ is the time taken to travel one mean free path $l$ the mean free path averaged over all of the molecules in both boxes. That is, since the temperature in Box 1 is smaller than the average temperature of the two boxes (and hence the average velocity of molecules in Box 1 are smaller than $v_{rms}$), the molecules in Box 1 will not travel the entire distance $l$ during $\Delta t$. This implies that less than half of the molecules in Box 1 will cross the dotted line and hence the energy crossing the dotted line from Box 1 should be less than $U_{1}/2$. Is there something incorrect with my reasoning or is the book skipping over the details?

Answer 1

Since $l$ is very small, the difference of temperatures of Box 1 and Box 2 are also small hence we can ignore the difference of the average speeds of molecules in the two boxes.

Answer 2

There are all sorts of differences between those two boxes (in addition to different temperature): different number density, different mean free path, different mean speed etc. All these differences are small compared to the mean value in either box. So in a rough calculation (and the calculation you are describing is very rough, giving no better than an order-of-magnitude estimate) we ignore those details. You are just trying to get a 'leading order' result, as we sometimes say.

The things left out in this simple calculation are any attempt to do the averaging more fully, allowing for different mean free path for different speeds, and the fact that it takes several collisions for any given molecule to thermalize, and the angles etc.