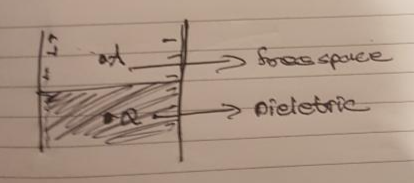
In the above set up I have two oppositely charged capacitor plates seperated by a distance 'd' with half of the region between filled with a dielectric material of constant $K$ and the other half in vacuum. My question is how would we prove that in both regions, the electric field is normal to the surface of plate at all points?
The context for the above is that I am trying to understand this answer which proves that the electric field is same in the dielectric media and the free space regions. It assumed that electric field only exists in direction normal to surface in region between plates and I'm trying to justify that.