Why is this result no contradiction to length contraction?

Question

Let's consider 2 events in an inertial system $S$: $$x_1=(0,0,0,0) \quad \quad \quad x_2=( \Delta t, \Delta x,0,0)$$

If we assume the two events occur in the same place we have: $\Delta \bar{t}= \gamma \Delta t$ for every other inertial system, also known as time dilation.

If we assume instead the 2 events occur at the same time in $S$ we get $\Delta \bar{x} =\gamma \Delta x$, hence in every other reference system (with non zero relative motion with respect to $S$?) we have that this length appears to be bigger than in the system $S$, which appears to be a contradiction to the effect of length contraction.

I don't see how to solve this issue: is it related that we give definition of "length" to some objects, which we assume to be at rest in the reference frame where we define the proper length? I'm not sure if in this case $ \Delta x$ can be associated to a length. I'm just very confused and (as you already know by now) new to relativity.

Thank you for any help!

Answer 1

The answer by Vincent Thacker is correct, but I hope I can clarify further.

The term "length contraction" or "Lorentz contraction" does not refer to a distance between any given pair of events. Rather it refers to a separation between two worldlines. The worldlines are typically the ones at the two ends of a given solid object. But how do you measure the distance between lines? If they were lines in space then we might for example employ the perpendicular distance, and there is just one answer. But for lines in spacetime there can be more than one suitable way to state the distance.

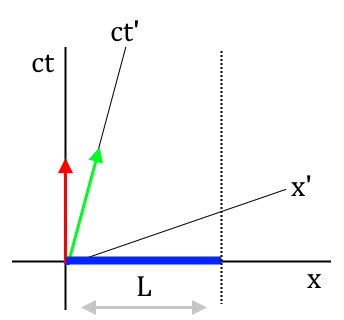
In particular, a suitable way to state the distance between the ends of a solid object is to pick events on the two worldlines (at the ends of the object) which are simultaneous. The length contraction idea refers to this distance. The specification of which pairs of events are simultaneous depends on the reference frame.

Here is my preferred way to present the calculation.

Consider a rod whose length is $L_0$ in frame S, where it is at rest. The two ends of the rod are therefore at $$ x_1 = 0, \;\;\;\; x_2 = L_0 $$ and this is where they are for all times $t$, where $t$ is the time coordinate in frame S.

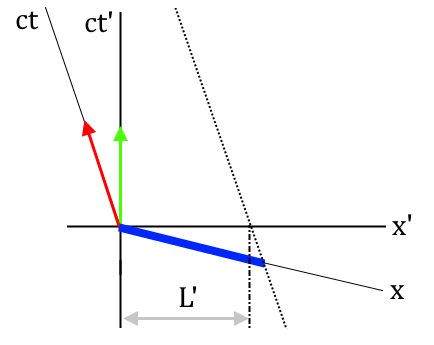
Now let's see where the ends are in frame S$'$. We employ the Lorentz transformation: $$ t' = \gamma( t - v x / c^2 ), \\ x' = \gamma( x - v t ) $$ so events on the worldline of the first end are at $$ t_1' = \gamma t, \\ x'_1 = -\gamma v t $$ and events on the worldline of the second end are at $$ t_2' = \gamma (t - v L/c^2), \\ x'_2 = \gamma(L- v t). $$ Now we want to find where the ends of the rod are, as measured in frame S$'$, at some moment in that frame. We pick the moment $t' = 0$. We find that at $t_1' = 0$, the above equations yield $x_1' = 0$. And at $t_2' = 0$ we obtain $t = vL /c^2$, which, when substituted into the equation for $x_2'$, gives $$ x_2' = \gamma( L - v^2L/c^2) = \gamma L (1-v^2/c^2) = \frac{\gamma L}{\gamma^2} = \frac {L}{\gamma}. $$ Hence the length of the rod is $x_2' - x_1' = L/\gamma$. Where, to repeat, by "length" we mean distance between the ends at events found to be simultaneous in the frame under consideration.

Answer 2

This may seem like a weird thing to require but to measure the length of a line, every point along that line has to exist at the same time. Let's say I want to measure the length of (a 1-dimensional) stick. I can give each of the points along that stick a pair of coordinates $(t,x)$. When I want to measure the length I will require by definition that each of the time coordinates is the same i.e. $t_1=t_2=t_3=\dots$ This is so obvious in your mind which is accustomed to Galilean relativity that you might forget it.

So let's draw a diagram. We have two frames: the primed and the unprimed frame. In the unprimed frame the length of the stick is $L$. Note that the stick traces out a rectangle in spacetime and to measure the length we took a slice at $t=0$ (the blue line).

Now we can transform to the primed frame using a Lorentz transformation. The blue line got transformed from the coordinates $(0,0), (0,L)$ to the coordinates $(0,0),(-\gamma\beta L,\gamma L)$. So you would think the new length of the stick is $L'=\gamma L$. In reality we have to consider a new time slice $t'=0$ and from the diagram we see that at $t'=0$ the length is smaller than $\gamma L$. By doing some calculations you will find that $L'=L/\gamma$.

Hint in case you want to do the calculations. You have to construct the dotted line in the primed coordinates. The line passes through the point $(-\gamma\beta L,\gamma L)$ and has slope $\Delta x'/\Delta t'=-v$ because the right end of the stick is travelling with $-v$ to the left. You can then read of the $x'$ coordinate of that line at $t'=0$.

Answer 3

To add to the answer by @AndrewSteane , the key idea is that
"the [proper] length of an object is the distance between the parallel lines [of its endpoints]"
and this distance is taken along a line perpendicular to those lines.
In relativity, "perpendicular to timelike lines" corresponds to "simultaneity", as suggested below.

• This should be familiar from Euclidean Geometry.
  
  Given two parallel lines (in red).
  Note that "perpendicular" to the radial red line is defined as "tangent to the [unit] circle".
  (The red frame would label that "line" by the radius.. call the radius "$t'=1$"... so all points on that tangent have are assigned "$t'=1$".)
  Through the origin, draw the parallel to that tangent. Those points are assigned "$t'=0$".
  The "proper length" is the green segment along this parallel.
  We mark the "right angle" at the red point on the other red line.
  
  In the resulting right-triangle,
  the "observed length" ($\Delta x=L_{obs}$) is the (blue) "hypotenuse",
  the "proper length" ($\Delta x'=L_{proper}$ and $\Delta t'=0$ in the object frame) is the (green) "adjacent side".
  So, $$L_{observed}={HYP}=\displaystyle\frac{ADJ}{\mbox{"cosine"}}=\frac{L_{proper} }{\cos\theta} \geq L_{proper}$$ ...since $\cos\theta\leq 1$.
  This is why [observed] shadows on the ground [of an upright meterstick] can look very long: $L_{observed} \geq L_{proper}$.
  (Implicitly, I am taking the angle of the lines (with respect to the vertical) to be less than 45 degrees… since I will use these same lines as worldlines of the endpoints of an object in a position vs time graph.)
  

• In Galilean relativity, on a "position-vs-time graph" with time running upward (a spacetime diagram), the "circle" is a horizontal line.
  All tangent lines will coincide... this is absolute simultaneity. No time dilation.
  Given the same two parallel lines (in red).
  By analogy with the above, we have a "degenerate right-triangle" so that $$L_{observed}={HYP}=\displaystyle\frac{ADJ}{\mbox{"cosine"}}=\frac{L_{proper} }{ 1} = L_{proper}.$$
  
  Hence, no length contraction: $L_{observed} = L_{proper}$, as well as no time dilation, etc....
  
  

• In Special relativity, on a "position-vs-time graph" with time running upward (a spacetime diagram), the "circle" is a hyperbola.
  Given the same two parallel lines (in red).
  (... following the same ideas in the Euclidean case above ...)
  In the resulting right-triangle,
  the "observed length" ($\Delta x=L_{obs}$) is the (blue) "hypotenuse",
  the "proper length" ($\Delta x'=L_{proper}$ and $\Delta t'=0$ in the object frame) is the (green) "adjacent side".
  By analogy, $$L_{observed}={HYP}=\displaystyle\frac{ADJ}{\mbox{"cosine"}}= \frac{L_{proper} }{ \cosh\theta_M } = \frac{L_{proper} }{ \gamma} \leq L_{proper}$$ ...since $\gamma=\cosh\theta\geq 1$.
  
  
  Hence, "length contraction": $L_{observed} \leq L_{proper}$.
  (Yes, the hypotenuse (in blue) is shorter than the adjacent side (in green).)
  To see this, I'll draw in a "hyperbola of constant spacelike separation" (dotted blue)
  

---

(For a discussion of the symmetry of Length Contraction,
visit my answers at Graphical explanation for length contraction and What exactly is the meaning of length contraction? .
This may also be helpful: Why is the condition stated this way for length contraction, in the derivation for the Lorentz transformations? )

Answer 4

The biggest problem with understanding special relativity is to understand that events that are simultaneous in one frame are not simultaneous in any other frame. Length in $S$ is measured by taking two measurements at the same time in $S$. The phrase "same time" should immediately alert you that it will not be the same time in another frame $S'$.

If $S'$ is moving with velocity $v$ relative to $S$, the Lorentz transformations are $$\Delta x' = \gamma(\Delta x - v\Delta t) \\ \Delta t' = \gamma(\Delta t - \frac{v}{c^2}\Delta x )$$ In the case of length contraction, we have $\Delta t = 0$ and it becomes $$\Delta x' = \gamma\Delta x \\ \Delta t' = -\gamma \frac{v}{c^2}\Delta x $$ As expected, there is a difference in time between these two measurements in $S'$ and therefore these do not measure the length in $S'$. In order to correct for the time difference, we need to subtract off the time difference multiplied by $-v$ (since we have taken the object to be stationary in $S$, from the view of $S'$, it moves with $-v$) to get $$\gamma \Delta x - (-v)\left(-\gamma \frac{v}{c^2} \Delta x \right) \\ = \frac{\gamma}{\gamma^2} \Delta x = \frac{1}{\gamma} \Delta x$$ which is the length in $S'$.

Answer 5

Let me start by saying that $x_2=( \Delta t, \Delta x,0,0)$ is not an event.

It's somewhat confusing. If you state that $\Delta x=\gamma x'$ you must put $\Delta t'=\frac{1}{\gamma}\Delta t$. You didn't do this though. Let me explain.

The Lorenz transformations are:

$$x'=\gamma(x-vt)$$

$$t'=\gamma(t-\frac{vx}{c^2})$$

For $t=0$ this gives for $x'$

$$x'=\gamma x,$$

so a distance $\gamma\Delta x$ is squeezed in a distance $\Delta x'$, which means space in the moving frame seems contracted (say a bus is squeezed).

When $x=vt$ we get

$$t'=\gamma(t-\frac{v^2}{c^2}t)\rightarrow$$

$$t'=\gamma t(1-\frac{v^2}{c^2}),$$

which means that

$$t'=\frac{1}{\gamma}t$$

So a duration $\Delta \frac{1}{\gamma}t$ is squeezed in a duration $\Delta t'$. This means that time seems to be dilated.