Time required for the formation of ice of some thickness in a lake

Question

The air above a large lake is at –2°C, while the water of the lake is at 0°C. Assuming that only thermal conduction is important and using relevant data selected from the given below find how many hours it would take for a layer of ice 10 cm thick to form on the lake’s surface.
Data(where $w$=water and $i$=ice):
$K_w=5.6Wm^{-1}k^{-1},$ $K_i=2.3Wm^{-1}k^{-1}$
$L_i=3.3*10^5J{kg}^{-1},$ $\rho_w=10^3kg m^{-3}$ $\rho_i=920kgm^{-3}$

This is how I solved.
Let the area of crossection of lake be A and same throught its depth.
Let the layer of ice formed at time $t$ be $x$ where $t$ is in seconds and $x$ is in meters.

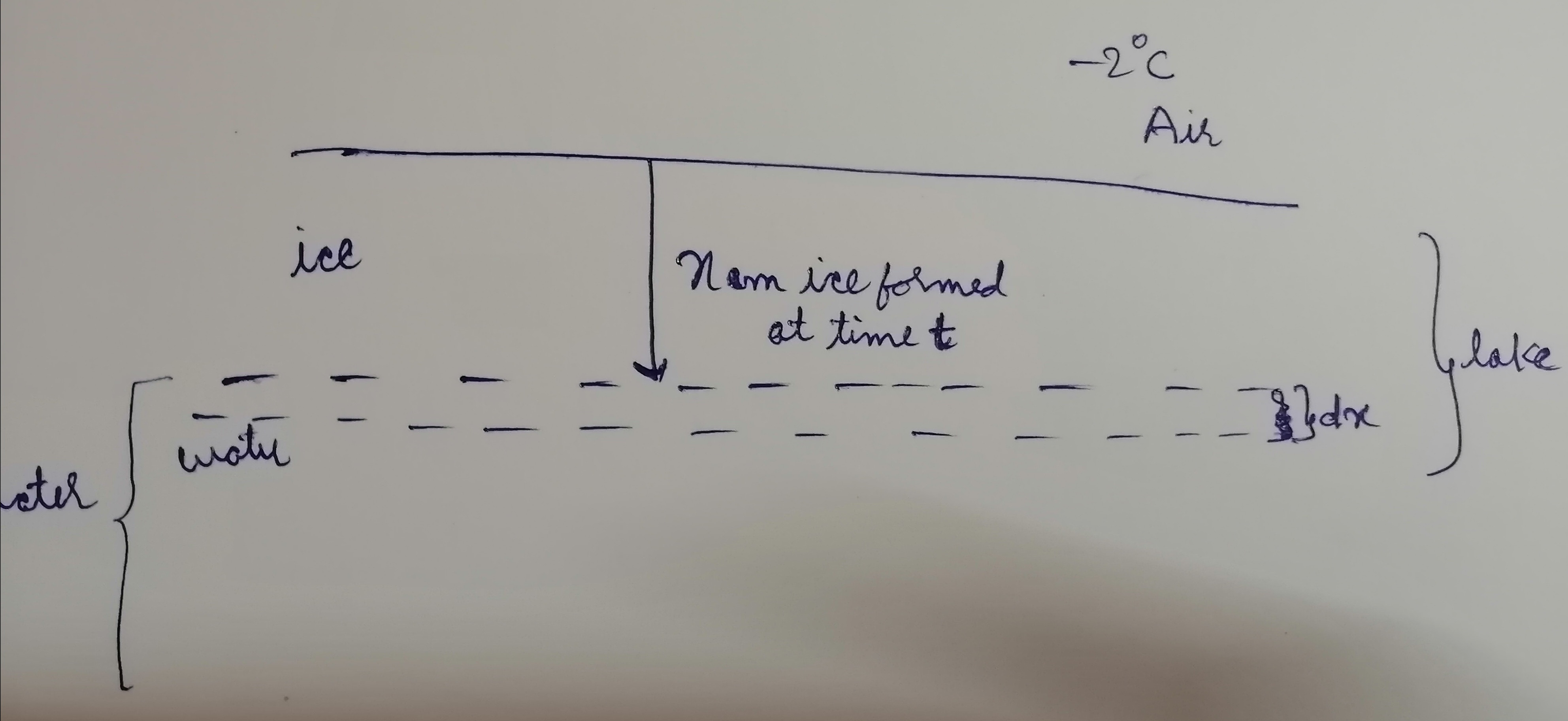
Now in time $dt$, $dm$ mass of water will be converted to ice but $dm=Adx\rho_w$.
So heat lost by water is $$dmL_i=A\rho_wL_idx$$
That amount of heat is transferred to the atmosphere through ice.
But heat transfer through ice is $$\frac{dq}{dt}=\frac{K_iA\Delta\theta}{x}$$
So heat lost by water is equal to heat transferred by ice. $$A\rho_wL_idx=\frac{K_iA\Delta\theta}{x}dt$$
Now on solving the differential equatio we get $$t=\frac{x^2\rho_wL_i}{2K_i\Delta\theta}$$
On substituing the values answer comes out to be 99.6 hours.
Where as the solution of the question has taken $\rho_i$ instead of $\rho_w$ which I took to solve the question.
This is here where I am stuck and unable to comprehend and understand why. After giving it an enough thought both seems to be correct.
Hence the final answer comes out to be 92 hours.

Answer 1

Assuming that only thermal conduction is important [...]

That's a very dicy assumption. There certainly is convection at the ice/air interface but assuming the water and ice are at the same temperature, convection at that boundary is $0$.

We can deal with that with a so-called overall heat transfer coefficient $U$:

$$\frac{\mathrm{d}q}{\mathrm{d}t}=UA\Delta\theta$$

$U$ is found using the principle of parallel heat resistors. $h_{ia}$ is the ice-to-air convection heat transfer coefficient:

$$\frac{1}{U}=\frac{1}{h_{ia}}+\frac{x}{K_i}$$

$$A\rho_iL_i\mathrm{d}x=UA\Delta\theta \mathrm{d}t$$

$$\frac1U=\frac{K_i+h_{ia}x}{h_{ia}K_i}$$ $$U=\frac{h_{ia}K_i}{{K_i+h_{ia}x}}$$ It then follows that: $$\rho_iL_i\mathrm{d}x=\frac{h_{ia}K_i}{{K_i+h_{ia}x}} \Delta\theta \mathrm{d}t$$ $$\rho_iL_i\left(K_i+h_{ia}x\right)\mathrm{d}x=h_{ia}K_i\Delta \theta \mathrm{d}t$$ Integrate as appropriate.

Answer 2

Note that the distance $x$ that you considered is the thickness of ice, not water. Hence $dx$ will be the elemental increase in thickness of ice, so we will use $\rho_i$ and not $\rho_w$. The rest of your calculation is correct.