3
$\begingroup$

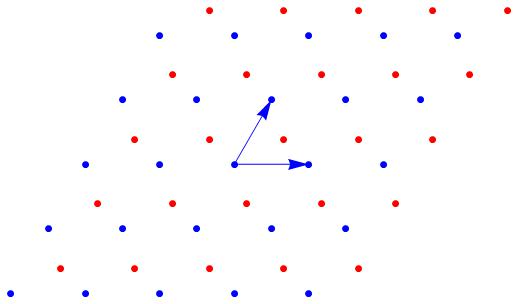
enter image description here

In the above image, I have a 2D crystal structure. The lattice vectors are described by:

a = {-1/2, -Sqrt[3]/2};
b = {1, 0};

and the location of atoms A and B are given by:

\[Tau][A] = {2/3, 1/3};
\[Tau][B] = {0, 0};

Now the problem I'm tasked with is to plot the locations of the atoms A and B, which I have done as seen above, where A is red and B is blue. I also have to plot the lattice vectors which I have done in blue arrows as seen above, and lastly I have to plot the basis vectors and this is where I don't know what to do.

I don't know what the basis vectors are. Googling has led me to discover that a lot of people use lattice vectors and basis vectors interchangeably, and overall, I have no clear definition to work with. Sometimes people say basis vectors are orthonormal, sometimes they need to be linear combinations with integers, sometimes with any real numbers.

I'm frustrated by the looseness with which all kinds of sources use these terms, and ultimately I don't have a clear reference to go with.

Improve this question
$\endgroup$
4
  • $\begingroup$ In the usual mathematical usage basis vectors must be mutually orthogonal. They are not, however unique. In a two-dimensional state in pair of perpendicular, unit vectors make a possible basis. In a case like this you would generally pick one of the lattice vectors to also be a basis vector and then pick the remaining basis vector as you please. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented May 6, 2013 at 19:32
  • $\begingroup$ @dmckee I'm not sure what you mean by "usual mathematical usage," but wouldn't you say that claiming basis vectors must be mutually orthogonal is misleading given that what's generally true is that they must be linearly independent? $\endgroup$
    – joshphysics
    Commented May 6, 2013 at 19:37
  • $\begingroup$ @dmckee Basis vector in crystallography has a very distinct meaning from basis vector in linear algebra. In crystallography, the basis vectors are what defines the positions of atoms within the unit cell, which in turn is defined by the lattice vectors. So in fact, the lattice vectors in crystallography are what in linear algebra would be called basis vectors... $\endgroup$
    – Lagerbaer
    Commented May 6, 2013 at 19:41
  • $\begingroup$ @joshphysics Damn. I believe you've caught be speaking out of turn. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented May 6, 2013 at 19:41

1 Answer 1

Reset to default
5
$\begingroup$

When talking about crystal lattices, the lattice vectors are what determines the translational symmetry of the crystal, and you have correctly identified those.

The basis vectors are the vectors that tell you where the different atoms in your unit cell are.

Thus, the basis vectors are those "locations of atoms A and B": The basis vector for atom B is just $(0,0)$ and the basis vector for atom A is $(2/3, 1/3)$. They tell you how to find your atoms within the unit cell once you have found the origin of that cell via the lattice vectors.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Yes I think this is the best-correct answer. $\endgroup$
    – Mark Rovetta
    Commented May 6, 2013 at 21:10
  • $\begingroup$ I had to go for a walk and think about this one, but now it makes sense! Much appreciated! $\endgroup$
    – user17338
    Commented May 6, 2013 at 21:21

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.