14
$\begingroup$

https://en.wikipedia.org/wiki/Blast_wave#Characteristics_and_properties says: "A blast wave travels faster than the speed of sound and the passage of the shock wave usually lasts only a few milliseconds."

I thought a blast wave was a sound wave, so I don't understand this.

Improve this question
$\endgroup$
2
  • 3
    $\begingroup$ There is absolutely no reason why an explosion couldn't push air molecules faster than 330 m/s. $\endgroup$
    – Criticizing Israel not allowed
    Commented May 8, 2021 at 12:15
  • $\begingroup$ @user253751 Would that necessarily be a blast wave? $\endgroup$
    – Matthew Christopher Bartsh
    Commented Dec 9, 2023 at 12:21

6 Answers 6

Reset to default
17
$\begingroup$

I think what it is referring to is the difference between an oscillating pressure wave propagating through air molecules (like sound) and the air molecules themselves moving in one direction, without oscillation, and experiencing significant displacement (like wind).

The expanding gasses in a gun barrel, for example. The bullet is supersonic so the expanding gasses pushing it must be supersonic too, but that's not the same as the sonic boom you hear which only travels at the speed of sound.

EDIT: There is some vagueness of where the line is crossed between oscillation and displacement in my above description since it is pretty qualitative, but I found this answer: How do we get supersonic bullets?

which states the speed of sound increases with pressure, so if you have a high pressure front it will be able to move faster than sound at ambient.

Improve this answer
$\endgroup$
2
  • $\begingroup$ "The bullet is supersonic so the expanding gasses pushing it must be supersonic too". True, but that isn't a blast wave, is it? $\endgroup$
    – Matthew Christopher Bartsh
    Commented Dec 9, 2023 at 12:24
  • $\begingroup$ @MatthewChristopherBartsh That is true. It's rather difficult to find distinct phenomena that clearly distinguish between air moving over large distances versus air that is oscillating since pretty much everything results in air oscillating. $\endgroup$
    – DKNguyen
    Commented Dec 9, 2023 at 18:42
9
$\begingroup$

I thought a blast wave was a sound wave

In the technical literature, a distinction is made between detonation, and deflagration. In both of these there is a rapid chemical reaction. The chemical reaction in one area stimulates the same reaction in a neighboring area. This creates a reaction "front", that moves rapidly from through the reactive material. The speed at which this front moves depends upon the type of "explosive". If the speed is supersonic, the "explosion" is called detonation. If the speed of the reaction front is below the speed of sound, the explosion is called deflagration. Both detonation and deflagration may cause loud "bangs" and destroy objects. So, to a casual observer, deflagration may appear similar to detonation. However the physics of detonations are different from those of deflagrations.

High explosives detonate, while black gun powder deflagrates. [Smokeless powder can detonate].

The Wikipedia article that describes as blast wave as supersonic is referring to the wave produced by detonation.

Improve this answer
$\endgroup$
8
  • $\begingroup$ Maybe this is a stupid question, but: what does it mean for a chemical reaction to occur at a velocity? I guess I've never really carefully thought about what units are on "fast" in phrases like "that reaction happens fast", but is it really meters/second? $\endgroup$
    – Daniel Wagner
    Commented May 8, 2021 at 17:11
  • $\begingroup$ @DanielWagner, I thought about this for a moment, and decided that "fast" is subjective when talking about, say, a quick colour change or phase change, but regarding explosion, one could precisely talk about the power of an exothermic reaction (energy released per time), and I suppose that this is what most people mean in that context. Perhaps "vigorous" is a better term for that, though... However, googling "definition of fast chemical reaction" turned up the notion of reaction half-life, which makes perfect sense. That is, how quickly do half of the reactants become the products? $\endgroup$
    – Jivan Pal
    Commented May 8, 2021 at 17:18
  • $\begingroup$ @DanielWagner — There is a formal definition. Regarding exothermic power, the notion of power half-life also applies, and I would guess is equal to the reaction half-life (since heat released is directly proportional to the amount of reactants consumed). The consensus seems to be that a reaction with a half-life on the order of milliseconds or less is deemed "fast". $\endgroup$
    – Jivan Pal
    Commented May 8, 2021 at 17:18
  • $\begingroup$ @JivanPal All of that makes perfect sense to me... but doesn't fit in at all with the usage from this answer, namely, "a chemical reaction that occurs at sub-sonic speeds". The term "sub-sonic" doesn't seem obviously malleable from m/s to half-life. $\endgroup$
    – Daniel Wagner
    Commented May 8, 2021 at 17:22
  • 2
    $\begingroup$ @DanielWagner. If you sprinkle black gunpowder on the ground in a long path, and light one end of the path, it will take some time for the reaction to reach the other end of the path. The distance between the ends of the path divided by the time between first ignition and the reaction reaching the far end is the speed of the reaction. If the black powder is packed very close together, instead of sprinkled, the speed will increase. But, as far as I know, the speed will never exceed the speed of sound for black gunpowder. On the other hand, for nitroglycerin, the speed will be supersonic. $\endgroup$
    – Math Keeps Me Busy
    Commented May 9, 2021 at 5:50
2
$\begingroup$

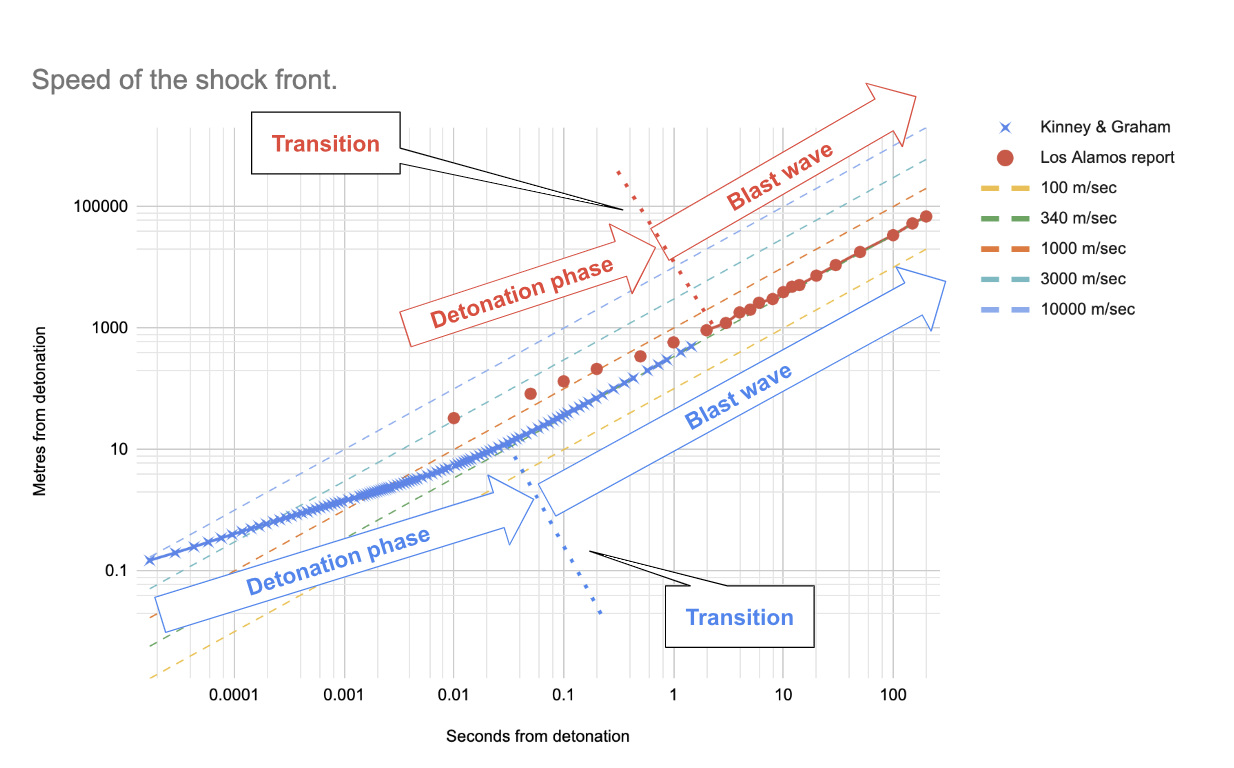
By consolidating shock propagation data from two distinct sources [1][2] into a single chart using a log scale for both axes, the contrasting speeds associated with two distinct phases of an explosion, which contribute to the formation of a shock front, become clearly evident. enter image description here

The detonation phase involves the expansion of hot gaseous products at an initial hypersonic speed. This speed eventually decays to the speed of sound, marking the transition to the blast wave. The blast wave detaches upon the completion of the detonation phase and then propagates as a pressure wave at the speed of sound in the surrounding air, or Mach 1.0.

The timing and location of this transition depend on the type and size of the explosion, with the blast wave originating within milliseconds of the detonation of a chemical explosion up to a few seconds of a nuclear explosion. In all cases, the blast wave propagates at Mach 1.0.

1 Kenney G. F and Graham K. J, (1985) Explosive Shocks in Air, Springer Science+Business Media, LLC, Table XI

[2] Bethe H. A, Fuchs K., Hirschfelder J, Magee J., Pearls R. and von Neumann J. (1958) Blast Wave U. S. Atomic Energy Commission, p. 185 ("The Los Alamos report")

Improve this answer
$\endgroup$
1
$\begingroup$

This is just an addition to the other answers already posted.

Note that the piston or driver of a shock wave is not limited by the speed of sound. Some common examples of pistons in Earth's atmosphere would be bullets, fighter jets, and space debris/rocks/meteors. Another example are caused by explosions, as your question eludes to.

A blast wave follows certainly relationships dependent upon initial energy, as I show in https://physics.stackexchange.com/a/242450/59023, https://physics.stackexchange.com/a/271329/59023, and https://physics.stackexchange.com/a/340487/59023.

I thought a blast wave was a sound wave, so I don't understand this.

You are correct, a shock wave is a nonlinearly steepened sound wave that has reached a balance between steepening and energy dissipation to form a stable discontinuity. Because it is being "pushed" by a piston/driver, the shock itself is not limited to the speed of sound, so long as the piston/driver has sufficient energy. As I show in the above linked answers, the Mach number, $M$, for a blast wave satisfies the following $M \propto t^{-3/5} \propto R^{-3/2}$. That is, with increasing time, $t$, or increasing radial distance from the explosion source, $R$, the Mach number will decrease. Eventually there will be no more shock wave.

Improve this answer
$\endgroup$
0
$\begingroup$

Yeah. The thing about gas is that any high-velocity particles are going to bump into other particles and quickly reach the average speed of the other particles in the gas (thermalizing). And the 'speed of sound' is actually a bit lower than the average particle speed, because the particles carrying a sound wave won't follow a completely straight line, they will get knocked about. (If you calculate it geometrically the speed of sound should be something like a factor of $\sqrt 2 $ lower than the average particle speed.)

But if you have enough high-velocity particles, they are just going to punch straight through and push a bunch of other gas particles with them. They won't thermalize for a while and you will see a blast wave.

Improve this answer
$\endgroup$
-1
$\begingroup$

Both existing answer touch the periphery of the question.

The underlying reason why the usual sound wave and the blast wave travel at different speeds is that the air (or any real-life acoustic media for that matter) is non-linear.

The acoustic waves travel by adiabatic compression and expansion if the medium. This is profoundly non-linear process.

enter image description here from http://hyperphysics.phy-astr.gsu.edu/hbase/thermo/adiab.html

The speed of sound squared is proportional to the elastic modulus of the media. https://en.wikipedia.org/wiki/Speed_of_sound

Elasticity of the air is the slope of the adiabatic curve.

It can be considered linear-ish for small pressure disturbances (like, less than 1% of the air pressure) and that's what the usual sound is.

A chemical explosion creates a change of pressure that is many times the atmospheric. A nuclear explosion changes the pressure by many orders of magnitude. In either case, the high pressure and temperature makes the elastic modulus of the media pretty much higher.

Roughly said, the blast wave carries its own high-pressure zone where it can travel faster.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.