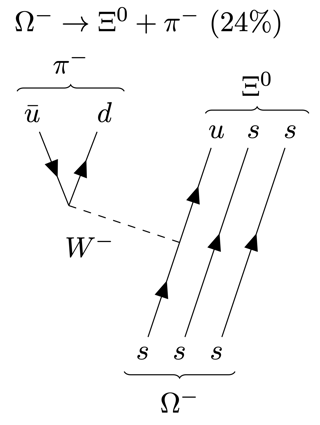
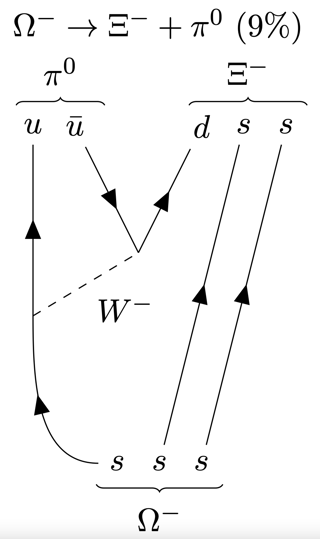
The $ \Omega^- $-decay can occur in a few different ways. According to this document (page 3, $\Omega^−$ DECAY MODES), the three most probable decays are \begin{align} \Omega^- &\to \Lambda^0 + K^- &(67.8\%);\\ \Omega^- &\to \Xi^0 + \pi^- &(23.6\%);\\ \Omega^- &\to \Xi^- + \pi^0 &(8.6\%). \end{align}
For these decays, I've made the feynman-diagrams below (are they correct?). The three decays all use the $W^-$-boson and thus the weak interaction.
Now my question is: if the three different decays use the same interaction (weak), why is there a difference in the probabilities for each decay. Has it something to do with the masses of the end products?
Masses:
- $\Omega^-\ (sss) = 1672 \,\text{MeV}/c^2$;
- $\Lambda^0\ (uds) = 1116 \,\text{MeV}/c^2$;
- $K^-\ (\bar{u}s) = 494 \,\text{MeV}/c^2$;
- $\Xi^0\ (ssu) = 1314 \,\text{MeV}/c^2$;
- $\Xi^-\ (ssd) = 1322 \,\text{MeV}/c^2$;
- $\pi^-\ (\bar{u}d) = 140 \,\text{MeV}/c^2$ and
- $\pi^0\ (u\bar{u}/d\bar{d}) = 135 \,\text{MeV}/c^2$.
Thanks in advance!