1
$\begingroup$

From grade 6th we have been taught that if we find the area of the velocity-time graph is distance covered or in other words if velocity is constant then distance= velocity* time as velocity = distance/ time and time cancels out.

So what would be distance* time?

Improve this question
$\endgroup$
2
  • 7
    $\begingroup$ Not everything has useful meaning. On the other hand, you might be more interested going in the opposite direction: Acceleration is change in velocity per time. Change in acceleration per time is called jerk. Change in the change in (that's not a typo) acceleration per time per time is called snap. I think it might even go beyond that. EDIT: It does. Crackle and pop. en.wikipedia.org/wiki/… $\endgroup$
    – DKNguyen
    Commented Apr 22, 2021 at 5:26
  • $\begingroup$ might want to check this: physics.stackexchange.com/q/456434/179405 $\endgroup$
    – Tomka
    Commented Apr 22, 2021 at 6:28

2 Answers 2

Reset to default
5
$\begingroup$

The integral of distance with respect to time is known as absement. It is one of the family of derivatives and integrals of position, and can be integrated further to get absity, abseleration and abserk.

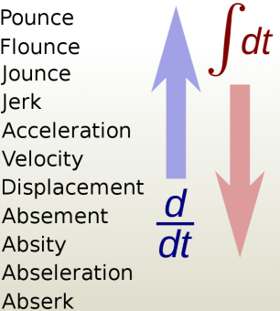
enter image description here

Absement appears when considering situations where a quantity depends on both how far something has moved or extended and how long the movement is maintained for. For example, it's been used in the study of memristors, artificial muscles and human posture.

Improve this answer
$\endgroup$
3
  • $\begingroup$ +1 for a good answer. May I ask for the link to the illustration you are showing? I have never heard the terms pounce and flounce before. $\endgroup$
    – Steeven
    Commented Apr 22, 2021 at 11:45
  • 1
    $\begingroup$ The wikipedia page on absement, which takes it from this paper: ieeexplore.ieee.org/document/7048123 $\endgroup$
    – Nick
    Commented Apr 22, 2021 at 11:49
  • $\begingroup$ Can displacement* time be space-time? I mean if we see space-time is nothing but 3 spacial dimensions and one-time dimension? $\endgroup$
    – Code breaker
    Commented Apr 23, 2021 at 2:30
0
$\begingroup$

As far as I know, distance $s$ multiplied by time $t$ or (as the differential analogue) the integral $$^{(*)}: \int_{t_0}^{t}s(\tilde{t})\text{d}\tilde{t}\ $$ are not used in the context of physics. Nonetheless, this does not mean that it cannot interpreted physically. If an object travels uniformly along a straight line, $^{(*)}$ will obviously be larger compared to an object accelerated uniformly along the same distance and within the same period of time. In general, $^{(*)}$ increases if an object is displaced farther away from its initial position for longer periods of time.
In a way, $^{(*)}$ measures for how long an object is displaced at which distance from its inital position. I can think only of a few "usecases" for $^{(*)}$. For example, if you were to stretch an elastic band and keep it stretched, you (at least ideally) do not do any work after you have reached the maximal displacement, but after some time you will obviously get tired and will have to relax the band. In this context, $^{(*)}$ might be used to measure ones physical ability to do such things.
However, I have never heard of anybody actually using $^{(*)}$ as a benchmark.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.