Say a regular string, with mass, is held by two persons in each end and they are in running in different acceleration in the same direction. Will there be any tension as different ends have different acceleration? Or not as they running in the same direction? If it does then how do you calculate that?
$\begingroup$
$\endgroup$
Add a comment
|
4 Answers
$\begingroup$
$\endgroup$
4
There are two possible scenarios:
- The fast accelerating person is in front.
- The slowly accelerating person in in front.
In case 2 there is no tension (the rope is not taut).*
In case 1, if the rope is rigid it will break. Otherwise the different accelerations are not possible.
Alternatively, if the rope is elastic, then elastic tension (spring force) will increase since the two people will separate more and more, corresponding to elongating the rope.
* If the rope is not massless, gravity might have an influence causing some tension within the rope depending on how it is carried and how it is hanging in its non-taut state. Finding the tension distribution depends highly on the exact shape with which it is hanging but can possibly be compared to a cable hanging between pillars of a suspension bridge.
-
$\begingroup$ Doesn't a non-taut massful rope still have tension to hold it up against gravity? $\endgroup$ Commented Apr 19, 2021 at 10:08
-
$\begingroup$ @user253751 Sure :) That is of course true. But let's assume an ideal massless rope in this scenario, since that is not the point we are focusing at. But for a heavy rope rather than a thin string, you are right that gravity should be considered as well. $\endgroup$– SteevenCommented Apr 19, 2021 at 15:28
-
$\begingroup$ The question explicitly specifies a massful rope $\endgroup$ Commented Apr 19, 2021 at 15:33
-
$\begingroup$ @user253751 That is a very good point... I have added a note to the answer. I believe the non-taut state is not the main focus of this question so I won't elaborate further. But good catch - thank you for the note. $\endgroup$– SteevenCommented Apr 19, 2021 at 15:41
$\begingroup$
$\endgroup$
If the string is not taut, then there is no tension. If the string is taut, and its ends are moving at different accelerations, then in Newtonian physics its length must be changing (and this would depend only on the difference between the accelerations, so one person acceleration at 10 m/s^2 and the other at 11 m/s^2 would be the same as one person being stationary and the other accelerating at 1 m/s^2).
If the distance is decreasing, then eventually the string would become slack. If the distance is increasing, then the string is being stretched, and stretching causes tension. The acceleration would not directly cause tension, but the acceleration would cause stretching and the stretching would cause tension. How much tension there is would depend on both the string's elasticity properties and how far it has been stretched. Eventually the increase in length would be enough to break it.
In relativity, it's more compliced, but under ordinary circumstances, we can ignore the relativistic effects.
$\begingroup$
$\endgroup$
Yes there is bound to be tension in the string . Consider an extreme case where one end is accelerated at 10000 m/ sec ^2 and the other at 1m/sec^2. Its obvious that the string would break . Why ? because of the tension of course now when the magnitude of acc is not that large there is bound to be some tension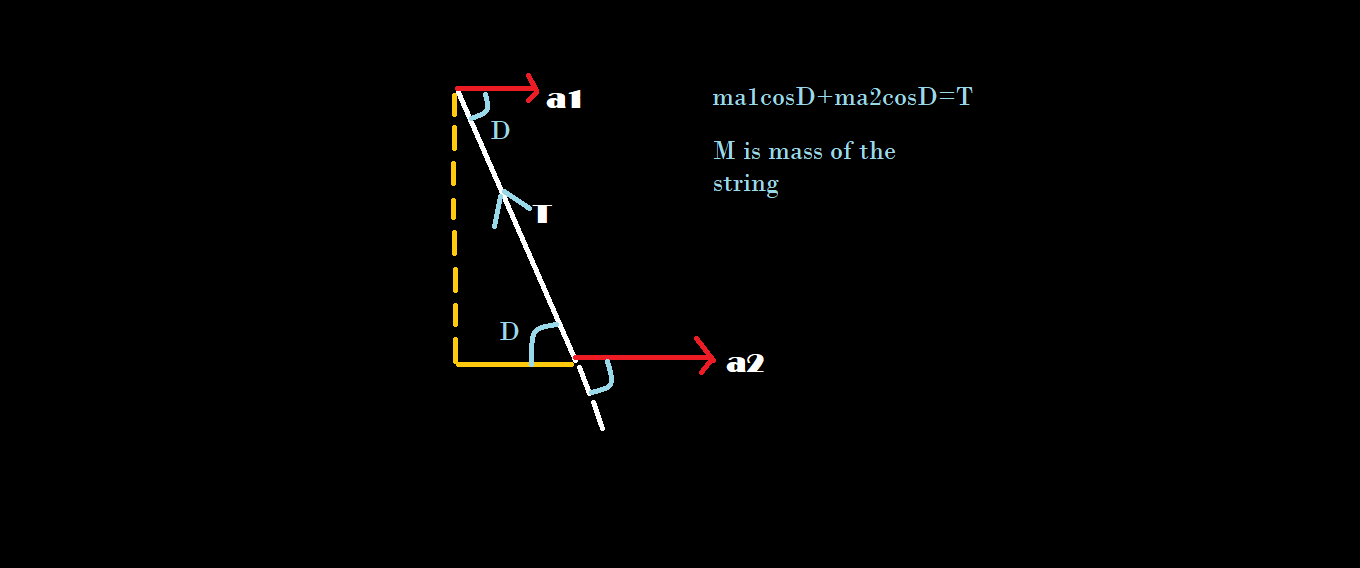 
$\begingroup$
$\endgroup$
0
To understand this question, you need to first specify which end is having greater acceleration.
Case 1 : Front end is having smaller acceleration
In this case, what actually happens is that the front end moves lesser distance than the back in a given time and thus the rope becomes slack and hence it is a complete "nuisance" to talk of tension force in this case..
Case 2 : Back end is having smaller acceleration
So I think this was the scenario you actually imagined. In this case, initially the man at the front end was having more acceleration than the back end and this will stretch the rope microscopically leading to cause some tension in the rope but the tension here will be adjusted in such a manner that the back end and the front end gets the same acceleration finally but if the initial difference gets too big the rope will eventually break apart in two pieces.
