Clearly, the Earth is accelerating (as it has centripetal acceleration), and thus it is a non-inertial frame of reference. Then, why don't we use pseudo forces all the time? My teachers told me that the effect produced by the centripetal acceleration of the Earth on our calculations is negligible and hence we can safely neglect pseudo force. But how? I want rigorous proof with examples that show exactly how much we are compensating in exchange for neglecting that the Earth is a non-inertial frame of reference.
-
1$\begingroup$ This is a good question. Hint: What would be the pseudo force on a rock on earth due to earth's revolution around the sun and what would be the force of gravity due to the sun on the same rock? $\endgroup$– user87745Commented Apr 15, 2021 at 14:01
-
$\begingroup$ Have you tried this first? en.wikipedia.org/wiki/Tide $\endgroup$– DanielCCommented Apr 15, 2021 at 14:02
-
2$\begingroup$ Related: physics.stackexchange.com/q/551843/195139 $\endgroup$– SandejoCommented Apr 16, 2021 at 5:32
-
$\begingroup$ Also related: physics.stackexchange.com/q/97743 $\endgroup$– David HammenCommented Apr 16, 2021 at 11:03
-
1$\begingroup$ @RIPANBARUAH - Just as an reminder, if an answer solved your problem or was the most helpful in finding your solution you could accept it by clicking on the checkmark. $\endgroup$– SG8Commented May 14, 2021 at 13:14
4 Answers
The way to properly answer your question is, for lack of a better word, by enumeration. Consider all the ways the Earth moves, compute the fictitious forces corresponding to them and check that they are negligible for the problem at hand. "All" is a strong word, but we can do quite well by looking at what goes into the expressions so that we can tell which ones are the most relevant.
The various motions can be all interpreted as compositions of rotations, so we can use the expression listed at the end of this Wikipedia section: the three kinds of fictitious force acting on a body of mass $m$, moving at velocity $v$, at a distance $r$ from the origin of the rotation (which happens at angular velocity $\Omega (t)$) are
- the Coriolis force, whose maximum magnitude is $2m \Omega v$,
- the centrifugal force, whose maximum magnitude is $m \Omega^2 r$,
- the Euler force, whose maximum magnitude is $m \dot{\Omega} r$ (the dot denotes a time derivative).
I'm saying "maximum" so we have an upper bound, which is attained when the vectors appearing in the vector products are perpendicular. Now, what is left is to take the system at hand, estimate the forces, and finally see how they compare to the precision of your experiment.
As an example, consider the rotation of the Earth around its axis. An upper bound for $r$ is the radius of the Earth, $r \approx 6400 \mathrm{km}$. The angular velocity is typically $\Omega \approx 2 \pi / (1 \mathrm{d}) \approx 7\times 10^{-5} \mathrm{Hz}$. The typical value of the variation of $\Omega$ is harder to estimate - it fluctuates a lot, from a quick look at the data here it seems like the variation of the length of a day to the next can be of the order of $100\mathrm{ms}$ at most. This relates to a variation of angular velocity of the order of $ 2 \pi (100 \mathrm{ms}) / (1 \mathrm{d})^2 \approx 10^{-10} \mathrm{Hz}$, therefore the quantity $\dot{\Omega}$ will be $\approx 10^{-10} \mathrm{Hz / d}$.
The velocity is dependent on your specific experiment: for concreteness' sake, let us assume we are considering things moving at $10 \mathrm{m/s}$ at most (as much as a fast cyclist, for instance). With these numbers, let us compute accelerations instead of forces (removing the $m$, so that our considerations generalize for any mass):
- the Coriolis acceleration will be at most $\approx 10^{-3} \mathrm{m/s^2}$,
- the centrifugal acceleration will be at most $\approx 3 \times 10^{-2} \mathrm{m/s^2}$,
- the Euler acceleration will be at most $\approx 6 \times 10^{-9} \mathrm{m/s^2}$.
This procedure can be applied for any other kind of rotation you are worried about --- for example, the rotation of the Earth around the Sun will have $\Omega \approx 2 \pi / (1 \mathrm{yr})$ and $r \approx 1 \mathrm{AU}$; this leads to a Coriolis force of the order of $\approx 4 \times 10^{-6} \mathrm{m/s^2}$ and a centrifugal force of the order of $\approx 5 \times 10^{-3} \mathrm{m/s^2}$.
From this analysis we can already tell that the most significant force to consider is the centrifugal one from the Earth's rotation, followed by the centrifugal one from the Earth's revolution, et cetera. If you had large velocities (for example, if you were doing ballistics) then the Coriolis contribution might be the largest.
So, take the experiment you want to perform, make some Fermi estimates like these, and see whether you can experimentally detect these effects! If it turns out you can, you should look at how exactly they affect your object since they are quite different: the full aforementioned expression shows how to determine their direction as well, and sometimes you might not be able to disentangle their contribution from something else. For example, if you are on the Equator the centrifugal force points radially outward, so it is equivalent to a decrease in the effective gravity at that point.
On the other hand, the Coriolis force deflects the motion of a particle, so it is easier to detect - it is the driving principle behind the Foucault pendulum. This experiment also illustrates the point that, while the accelerations are generally small, if you let them act for a long time their cumulative effect can be rather large.
This procedure (estimating the typical size of the corrections) is quite general: it can also be applied to check whether it makes sense to use Newtonian mechanics in your case, or if you should account for general relativity, quantum mechanics or some other more complicated theory.
-
2$\begingroup$ A thorough answer! Is there some case to make that the centrifugal force from the Earth's rotation is already taken into account by reducing the effective force of gravity? Eg: if I calibrate a set of scales using a calibrated mass, that mass was subject to the centrifugal acceleration during calibration, and so will be everything it is used to weigh. Does using an adjusted value of g much further reduce errors, and is this done in practice? $\endgroup$– Tom VCommented Apr 16, 2021 at 15:08
-
$\begingroup$ @TomV I must admit I'm not an expert in gravimetry, but it looks like the answer is yes: it is more convenient to define the gravitational field of the earth by including the centrifugal contribution. $\endgroup$ Commented Apr 16, 2021 at 20:52
-
1$\begingroup$ I agree with @Jacopo Tissino. The force of gravity is typically modified by subtracting the centrifugal force. The centrifugal force depends on latitude; maximum at the equator, zero at the poles. See the text Simon, Mechanics, for details. $\endgroup$ Commented Aug 21, 2021 at 20:09
There's two things you might mean by "centripetal acceleration of the Earth", but it turns out that you don't really need to worry about either one under most circumstances.
Orbital motion
If you're referring to the fact that the Earth is accelerating towards the Sun due to its orbit, then this would give rise (in a frame accelerating with the Earth) to a fictitious force pointing away from the Sun. In this reference frame, we might expect that objects would be pushed away from the Sun by this fictitious force. But any object would also be attracted to the Sun; and the magnitude of this gravitational attraction towards the Sun is (more or less) the same as the fictitious force away from the Sun. So the two effects cancel each other out (more or less) and we can safely ignore them both.
(Aside: the two only "more or less" cancel out because at most points on the Earth, you are actually slightly closer or slightly farther from the Sun than the center of the Earth is, and so you accelerate at a slightly smaller or larger rate than the Earth does. These small differences are the basis for understanding tidal forces.)
Rotational motion
If you're referring to the rotation of the Earth, in a frame rotating with the Earth there would be a centrifugal force of magnitude $- m \vec{a}_\text{rot}$ pointing away from the rotation axis (where $\vec{a}_\text{rot}$ is the centripetal acceleration of a point on the surface), and a gravitational force $m \vec{g}_0$ due to the gravitational attraction of the Earth (what the gravitational force would be if the Earth wasn't rotating.) The net force on an object due to these effects would be $$ \vec{F} = m \left( \vec{g}_0 - \vec{a}_\text{rot}\right). $$ But importantly, there is no way to disentangle these forces. Every object will get an acceleration of $\vec{g}_0 - \vec{a}_\text{rot}$ due to these effects. Since they are not distinguishable from each other, we can define the effective gravitational acceleration of an object near some point on Earth's surface to be $\vec{g} \equiv \vec{g}_0 - \vec{a}_\text{rot}$; and then the force on such an object is $\vec{F} = m \vec{g}$.
(Aside: this implies that the gravitational acceleration $|\vec{g}|$ actually varies from point to point on Earth's surface, since the magnitude of $\vec{a}_\text{rot}$ is larger near the equator and smaller near the poles. This effect is amplified by the oblateness of the Earth—which is itself due to the rotation of the Earth—with the result that $|\vec{g}|$ varies by about 0.03 m/s2 over various points on the Earth's surface.)
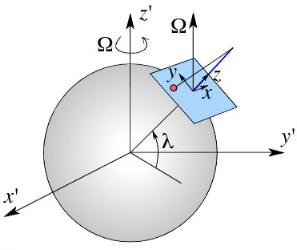
the pseudo forces are:
$$\vec F_p=\underbrace{m\,\left(\vec \Omega\times (\vec \Omega\times \vec R)\right)}_{\text{Centrifugal force}}+ \underbrace{2\,m\,(\vec \Omega\times {\dot{\vec{R}}})}_{\text{Coriolis force}}$$
with:
$$\vec R=\begin{bmatrix} x \\ y \\ z \\ \end{bmatrix}\qquad,{\dot{\vec{R}}}=\begin{bmatrix} v_x \\ v_y \\ v_z \\ \end{bmatrix}\\ \vec \Omega=\Omega\,\begin{bmatrix} 0 \\ \cos(\lambda) \\ \sin(\lambda) \\ \end{bmatrix}$$
$\Rightarrow$
$$\vec F_p=\left[ \begin {array}{c} m{\Omega}^{2}x-2\,m \left( \cos \left( \lambda \right) v_{{z}}-\sin \left( \lambda \right) v_{{y}} \right) \Omega\\ -m\sin \left( \lambda \right) \left( \cos \left( \lambda \right) z-\sin \left( \lambda \right) y \right) { \Omega}^{2}-2\,m\sin \left( \lambda \right) v_{{x}}\Omega \\ m\cos \left( \lambda \right) \left( \cos \left( \lambda \right) z-\sin \left( \lambda \right) y \right) {\Omega}^{2}+2 \,m\cos \left( \lambda \right) v_{{x}}\Omega\end {array} \right] $$
with $~\Omega=7.27\,10^{-5}~[\frac 1s]$ and $~\lambda=\frac{\pi}{2},~\vec\Omega=[0~,0~,\Omega]^T$
$$F_p=\left[ \begin {array}{c} 5.2 \,10^{-9}\,mx+ 1.4 \,10^{-4}\, mv_{{y}}\\ 5.2 \,10^{-9}\,my- 1.4 \,10^{-4}\,mv_{{x}}\\ 0.0\end {array} \right] $$
hence the pseudo force components (unit [N]) are very small and can neglected
I don't think there is rigorous proof as these things depend more or less on the experiment, you are performing and the accuracy you needed. If you need more accurate results or it might possible that your experiment is a long time range, So you want to include these effects.
But Less talks few examples, Maybe we wanna look at gravity that is effective gravity due to centrifugal force.
Quoting Wikipedia: The surface of the Earth is rotating, so it is not an inertial frame of reference. At latitudes nearer the Equator, the outward centrifugal force produced by Earth's rotation is larger than at polar latitudes. This counteracts the Earth's gravity to a small degree – up to a maximum of $0.3$ % at the Equator – and reduces the apparent downward acceleration of falling objects.
The effects of the surface centrifugal force due to rotation mean that sea-level gravity increases from about $9.780$ m/s$^2$ at the Equator to about $9.832$ m/s$^2$ at the poles, so an object will weigh approximately $0.5$ % more at the poles than at the Equator.
Recall that:
$$g_\text{eff}=g-R\Omega^2\cos\phi$$ $$\frac{\Delta g}{g}=\frac{R\Omega^2\cos\phi}{g}$$ For $\phi =0$ $$\frac{\Delta g}{g}=3.44\times 10^{-3}$$
One more example can be the Coriolis force. You can look for my answer which does the calculation (it's too approximate but convey the thought).
For more accurate calculation look for Exercise 9.9 Train on Track in Introduction to Mechanics by Klepner.
A 400-ton train runs south at a speed of 60 mi/h at a latitude of 60◦ north. What's the magnitude of horizontal force?
Which turn out to be $277 \ lbs$.