Rotating a stone with a string

Question

Suppose we are given a stick and a stone tied to the stick by a string. Now if we rotate the stone around the stick the stone rises in height (see picture below). My question is which force accounts for this rise in height?

According to me (please correct me if I am wrong) this happens due to centrifugal force. The centrifugal force is directed along the string outwards, so we can resolve it into two components, one is the horizontal component and the other is the vertical component. If the mass of the stone is m, its velocity is v, the length of the string is r, and the angle the string makes with the horizontal is θ, then the total centrifugal force is mv^2/r, the horizontal component of the centrifugal force is mv^2/r * cos(θ), and its vertical component is mv^2/r * sin(θ) (see picture below). Can anyone tell me if I am correct? The directions of the forces are given in the picture, but I am not sure whether the directions are right or not. Also note that in the second picture for sake of clarity I have not drawn the direction of rotation of the stone.

Answer 1

First of all $mv^2/r $ is a pseudo force that acts in frame of rotating body itself. So, let's work it like that.

The centrifugal force acts outwards radially from the circle of rotation (not along the thread).

First let's see the the first image.

$$T\cos\theta=mg$$ $$T\sin\theta=\dfrac{mv^2}r$$

As we move the body faster. $T\sin\theta$ must increase keeping the $T\cos\theta$ component constant(equals $mg$.)

So, both $T,\theta$ must increase to balance the centrifugal force and keep vertical component constant.

Hence , body moves upwards while speed of rotation increases.

Also, see that the motion with $\theta\ge90^0$ are not possible due to the vertical component are not balanced. The max. tension ($T\to\infty$) comes when $\lim \theta\to 90$

Answer 2

Firstly, the centrifugal force is an pseudo force and only appears to exist if you are sitting on the stone and flying along with it. This is because you are in an accelerating (non-inertial) frame of reference. Another common pseudoforce you experience every day is the "force" that throws you into the seat of your car when it accelerates. For further explanations of the centrifugal "force" see this wiki article.

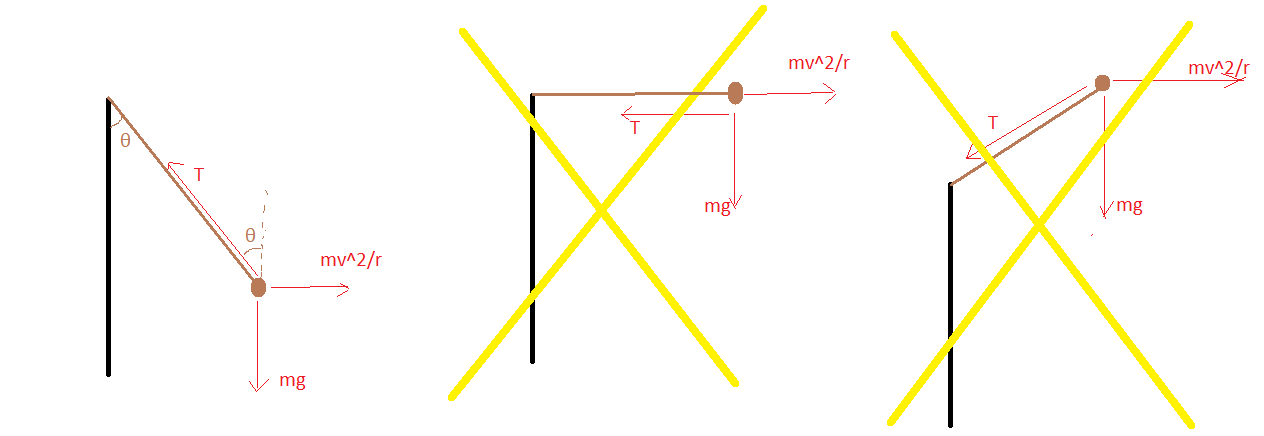
To treat your problem properly the observer (you) needs to be stood still watching the pendulum. The only forces acting on the stone at the instant of your diagram are 1) gravity and 2) the string pulling on the stone. These two forces exist whether or not the stone is rotating and are the only two forces acting on the stone.

Force 1) gravity, always points down towards the earth.

Force 2) the tension points along the string, away from the stone.

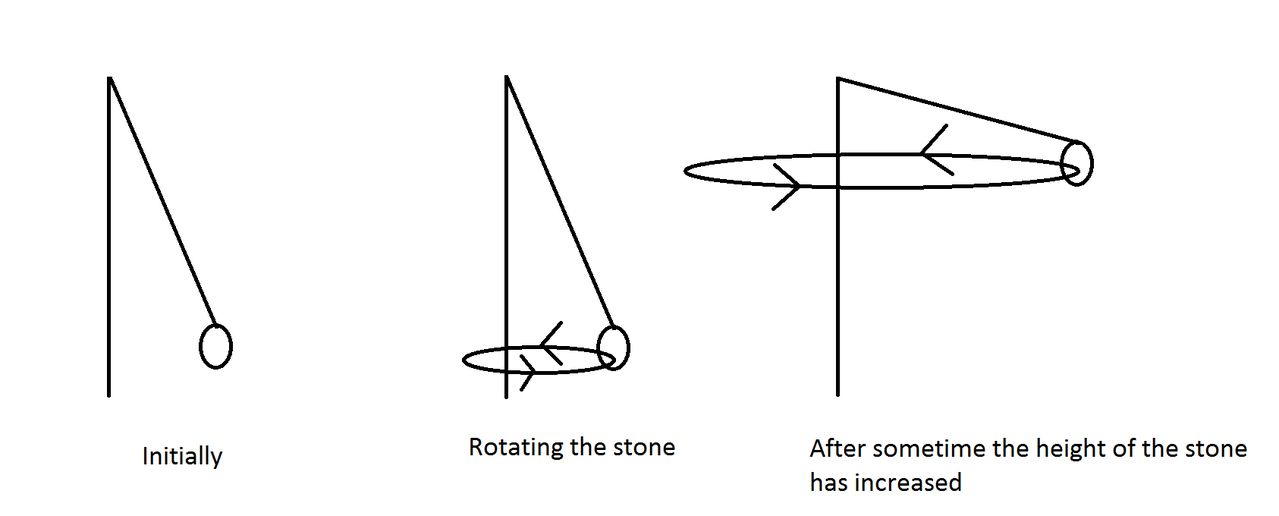
When the stone is just hanging at rest (no rotation) it is obvious that these two forces cancel out and the stone does not move. So what is different when the stone is rotating?

If the stone is made to rotate, it now has momentum in some direction. At any instant, the stone is trying to fly off in a straight line (think what happens if the string were to snap) but it can't because the string is holding it back. Instead, the force of the string pulling on the stone changes the stone's direction by accelerating it towards the stick. This happens constantly - the stone is trying to fly off in a straight line while the string pulls it towards the stick.

Now the faster the stone is going, the harder the string must pull to make it travel in a circle. So when the stone is rotating, the tension in the string must apply this force called the centripetal force . The centripetal force acts radially, from edge of the rotation circle to the centre. Here's a picture:

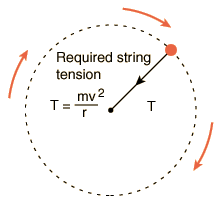
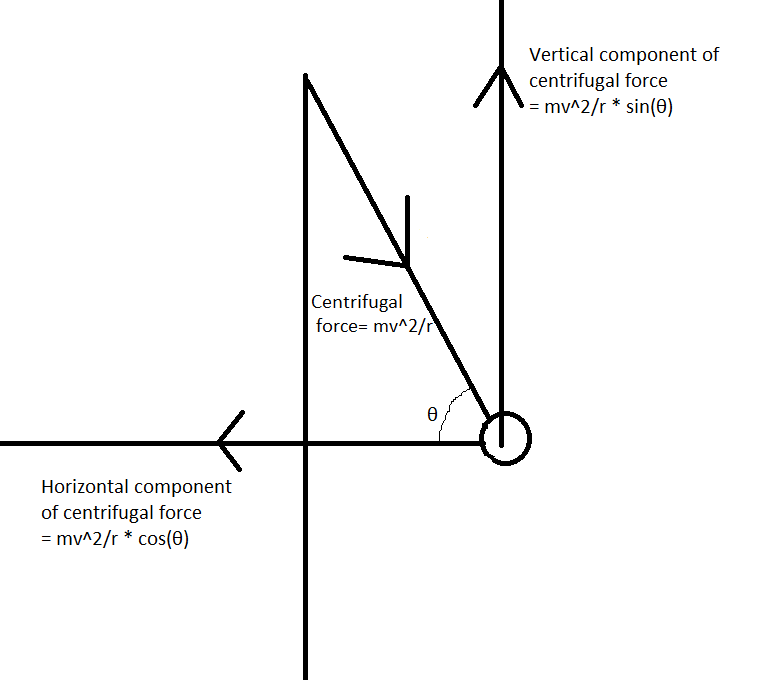
Finally, the tension in the string needs two components. 1) It must counteract the force of gravity on the stone, 2) it must apply the centripetal force to stop the stone from flying away. 1) is the vertical component, 2) is the horizontal component so the combined force 1)+2) will be at an angle (and hence the string will be at an angle relative to the stick).

Answer 3

Basically no force except tension acts , 1 thing is centrifugal force is a pseudo force .

Secondly length of string is constant , so when you rotate too fast .

Faster than what $\frac{mv^2}{r}$ = $Tcos\theta$(horizontal force) permits for a radii $r$ , then horizontal force has to increase , and T in the string can only attain a max value(after which it will break),suppose it has it now, .

And it wants to compensate still for as $cos\theta$ has to increase $\theta$ will have to decrease towards $0$, and it will do it when height of the ball will increase w.r.t. ground and radius of the ball's circle(base) will increase , since hypotenuse(length of string) remains constant .

So height rises be causes length of string is constant and you need more centripetal force and for that $cos \theta$ has to increase and for that base has to increase for the right triangle in your image . and tension is directed away from ball along string .