0
$\begingroup$

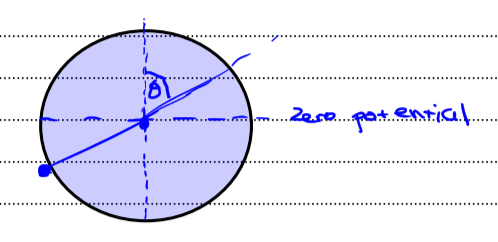
I have been trying to find the value of theta at which a string goes slack in a vertical circle, by letting the diameter of the circle be the zero potential

diagram

My question is would the gravitational potential above and below the zero potential have opposite signs?

I am well aware that potential energy is a scalar quantity however when I apply the conservation of energy $$K.E_{initial} + P.E_{initial}= K.E_{final} + P.E_{final}$$

The only way I get a satisfactory answer (one that matches the answer on the book) is when the potential energies have opposite signs.

Improve this question
$\endgroup$
3
  • $\begingroup$ Is it perhaps that the change in potential energy overall would be a net gain and not a net loss? $\endgroup$
    – Filthyscrub
    Commented Apr 8, 2021 at 18:17
  • $\begingroup$ And if so would alternating signs above and below the potential line account for this? $\endgroup$
    – Filthyscrub
    Commented Apr 8, 2021 at 18:18
  • 1
    $\begingroup$ What string? Is it acting on a object? What would cause it to go slack? $\endgroup$
    – R.W. Bird
    Commented Apr 10, 2021 at 15:13

1 Answer 1

Reset to default
1
$\begingroup$

The zero potential plane also called Datum plane is the plane where gravitational potential is considered to be 0. Now if you where 5 m above this plane initially and came down to 4 m your potential energy would reduce from mg $\times$ 5 to mg $\times$ 4. In short potential energy decreases with decreasing height.Now when you go below datum plane why should the rule be any different ? But we know that potential energy is 0 at this plane .So if you go below this plane your potential energy should decrease i.e become negative .

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.