However, once the textbooks define the work in this manner, they often
jump straight into PV indicator diagrams that represent the system's
internal pressure! For example, an isobaric process is a horizontal
line which does work, but many times this is actually the system
pressure.
Without an explanation of the process(es) involved, a PV diagram only tells you what the external pressure is. The system pressure only equals the external pressure if you are told the process(es) is (are) reversible.
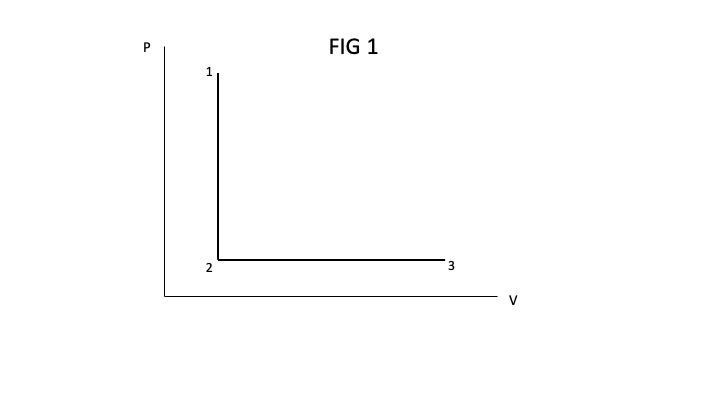
To illustrate, consider the PV diagram in FIG 1 below. If we are told that process 1-2 is a reversible isochoric (constant volume) heat extraction process and process 2-3 is a reversible isobaric expansion process, then we know that P in the diagram is both the external pressure and the equilibrium pressure of the gas (system).
On the other hand, suppose we are told at equilibrium state 1 the external pressure P1 abruptly drops to P2. An example is a vertically oriented cylinder with piston where weight is suddenly removed from the piston so that the external pressure suddenly drops to P2. Then the gas allowed to expand irreversibly at constant external pressure until it re-equilibriates with the surroundings.
In the example path 1-2-3 the processes are irreversible and the gas pressure is only equal to the external pressure at the equilibrium states 1 and 3.
- Is the work done by the system equal and opposite to the work done by the surroundings? If so, how can this be proven generally?
Is this just effectively a restatement of Newton's 3rd law?
It is and it can be proven by conservation of energy. Work is energy transfer. When body 1 does positive work on body 2, body 1 transfers energy to body 2. At the same time body 2 does negative work on body 1 (the force being opposite to the displacement) absorbing the energy transferred by body 1, for conservation of energy.
In this example, or any example where work is done, it is a restatement of Newton's third law, since the force body 1 exerts on body 2 is equal and opposite to the force body 2 exerts on body 1. But keep in mind that although equal and opposite work always follows from Newton's third law, Newton's third law does not necessarily lead to equal and opposite work being done. For that, we need to apply Newton's second law to each body individually.
For example, if I exert a force F on you, you exert an equal and opposite force F on me per Newton's third law. But if maximum static friction force between my feet and the ground is not exceeded, and the maximum static friction force between your feet and the ground is not exceeded, per Newton's second law the net force on each of us is zero and no work is done.
- For practical problems, do we decide to choose which ever is more convenient for the calculation? For example, if I had a piston in an
external pressure reservoir, then it is obviously most easiest to
calculate the work with the known external pressure instead of the
more complex evolving internal pressure?
If the process(es) are reversible, then either the external or system pressure can be used because they are the same. But if the process(es) are irreversible, we have no choice but to use the external pressure. More importantly, if the process(es) are reversible we can use the applicable equation of state to describe the relationship between the system properties at each point in the process, because the system is in equilibrium. That is not the case if the process(es) are irreversible, as in the above example.
- How can I show that isothermal expansion/compression under a constant external surrounding pressure is equal to the work done by
the internal pressure if they are equal and opposite? If I consider
the above formula for work, then the work done by surroundings on
system is $W = -P_{ext}\Delta V$
First of all, an isothermal expansion/compression cannot be carried out under constant external pressure if the meaning of "isothermal" is in the ordinary sense, i.e., that the gas temperature is always in equilibrium with the temperature of the surroundings, which is only the case for a reversible isothermal expansion/compression.
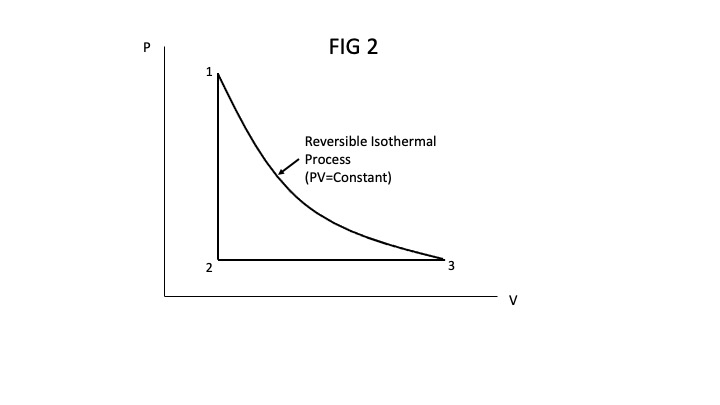
For a reversible isothermal process the product of pressure and volume is constant throughout the process, or $PV$=constant. See Fig 2 below. In this case the work is not $P_{ext}\Delta V$ but instead is reversible work given by
$$W_{rev}=\int PdV=nRT\ln \frac{V_2}{V_1}$$
Where $P$ is both the external pressure and the equilibrium pressure of the gas. Nevertheless, for the reversible isothermal process the work done by the gas on the surroundings is equal and opposite to the work done by the surroundings on the case.
On the other hand, in the case of an irreversible "isothermal" process, path 1-2-3 in FIG 2, where the external pressure and temperature is constant and the system is in constant contact with a constant temperature surroundings (thermal reservoir or bath), we have irreversible work given by
$$W_{irr}=P_{ext}\Delta V=P_{2}(V_{3}-V_{2})$$
Here again, the work done by the gas on the surroundings is equal and opposite to the work done by the surroundings on the gas, because at the boundary between the system and surroundings both the pressure and temperature is constant. It is just a different amount of work than for a reversible isothermal process.
Hope this helps.