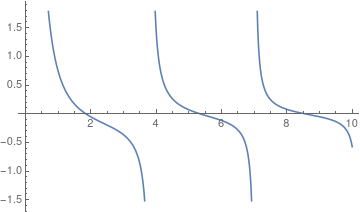
In a step-index optical fiber, the eigenvalue equation for the $\mathrm{HE}_{11}$ fundamental mode, under the weak guidance approximation, can be written as
$$\frac{J'_n (u)}{u J_n (u)} = - \frac{K'_n (w)}{w K_n (w)} - n \left( \displaystyle \frac{1}{u^2} + \frac{1}{w^2} \right)$$
It can be plotted with the same procedure shown in this answer. Refer to the link also for the notation used here.
This is the Left Hand Side with $n = 1$:
The $\mathrm{HE}_{11}$ arises when the first (leftmost) branch is intersected. This mode is well-known because it has no cutoff frequency, so even with a $v = a \omega \sqrt{\mu_0 \epsilon_0} \sqrt{n_1^2 - n_2^2} \to 0$, there should be an intersection. However, once $v$ has been fixed, the Right Hand Side plot is only available for the values of $u$ such that $w = \sqrt{v^2 - u^2} \geq 0$, that is $u < v$.
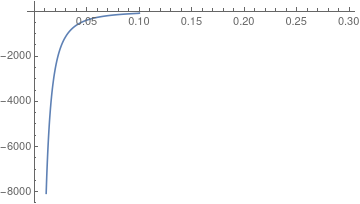
For example, with $v = 0.1$, this is the Right Hand Side:
It stops well before being able to intersect the first branch of the Left Hand Side. So, how can the $\mathrm{HE}_{11}$ mode exist?