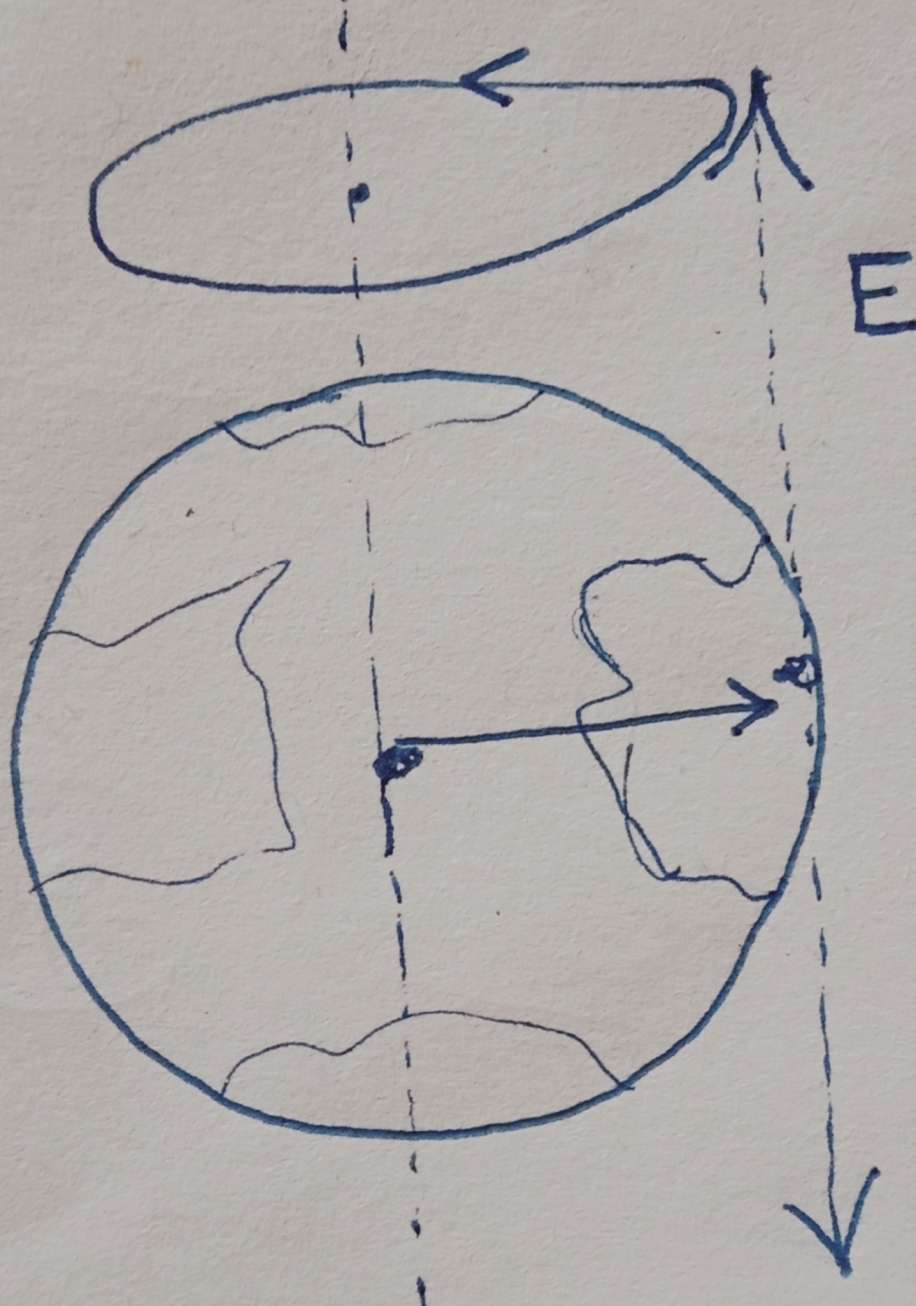
your position on the sphere surface from the sphere center is:
$$\mathbf R=\left[ \begin {array}{c} r\cos \left( \theta \right) \sin \left( \phi
\right) \\ r\sin \left( \theta \right) \sin \left(
\phi \right) \\ r\cos \left( \phi \right)
\end {array} \right]
$$
where $~r~$ is the sphere radius $~\phi~$ the polar coordinate and $~\theta~$ the azimuth coordinate.
the sphere coordinate system if rotating about the z-axes with constant angular velocity $~\omega$, thus the position vector in the rotating system is:
$$\mathbf R_r=\left[ \begin {array}{ccc} \cos \left( \omega\,t \right) &-\sin
\left( \omega\,t \right) &0\\ \sin \left( \omega\,t
\right) &\cos \left( \omega\,t \right) &0\\ 0&0&1
\end {array} \right]
\,\mathbf R$$
from here you can obtain the velocity:
$$\mathbf v=\frac{\partial \mathbf R_r}{\partial \phi}\,\dot{\phi}+
\frac{\partial \mathbf R_r}{\partial \theta}\,\dot{\theta}+\frac{\partial \mathbf R_r}{\partial t}$$
with the kinetic energy $~T=\frac m2 \mathbf v\cdot \mathbf v~$ the potential energy
$~U=m\,g\,\mathbf R_r\cdot \mathbf e_z~$ you obtain the equations of motions
$$\left[ \begin {array}{c} \ddot\theta \\
\ddot\phi \end {array} \right]
=\left[ \begin {array}{c} 2\,{\frac { \left( \dot\theta +\omega \right)
\cos \left( \phi \right) \dot\phi }{\sin \left( \phi \right) }}
\\\\ -{\frac {\sin \left( \phi \right) \left( 2\,r\,\dot
\theta \,\omega\,\cos \left( \phi \right) +r\,{\dot\theta }^{2}\cos \left(
\phi \right) +r{\omega}^{2}\cos \left( \phi \right) +g \right) }{r}}
\end {array} \right]
$$
The pseudo forces is:
$$\mathbf F_s= m\,\left[ \begin {array}{c} {\omega}^{2}x+2\,\omega\,{\it \dot y}
\\ {\omega}^{2}y-2\,\omega\,{\dot x}
\\ 0\end {array} \right]
$$
where
$$x=\mathbf R\cdot \mathbf e_x~,\dot x=\frac{\partial x}{\partial \phi}\,\dot \phi+
\frac{\partial x}{\partial \theta}\,\dot \theta$$
$$y=\mathbf R\cdot \mathbf e_y~,\dot y=\frac{\partial y}{\partial \phi}\,\dot \phi+
\frac{\partial y}{\partial \theta}\,\dot \theta$$
you can obtain now the equations of motion with Newton-Euler method
$$m\,\ddot{\mathbf R}=-m\,g\,\mathbf e_z+\mathbf F_s$$