Faraday's law for a 3-dimensional conductor plate moving in a uniform magnetic field

Question

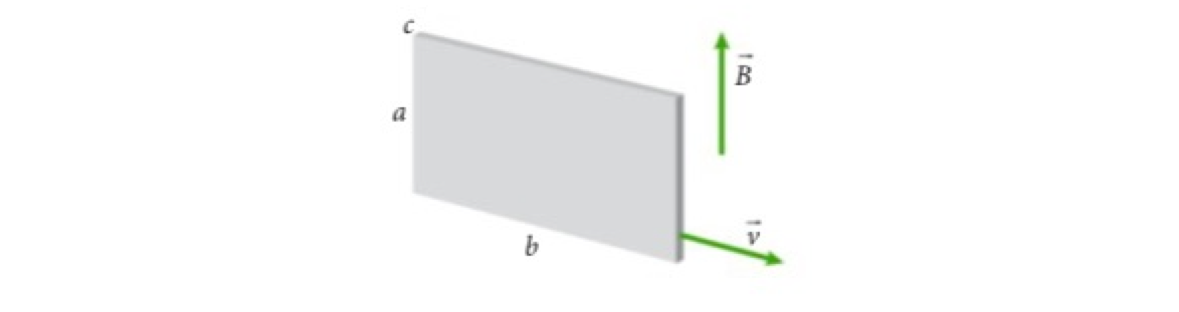
I am struggling to understand this supposedly simple problem I found in a highschool textbook.

A metallic plate is moving with constant velocity v in a region in which there is a uniform magnetic field (oriented as in figure).

• Explain why the two surfaces with sides $a$ and $b$ are electrically charging. Is it correct to assume, in first approximation, that the charges are uniformly distributed?

• Compute the surface charge density of the two surfaces and the potential difference between them.

• Compute the current that is flowing through a conductor with resistance $R$ connecting the two surfaces.

  

Now, it seems the object is moving inside the region with the uniform magnetic field, it is not entering the region nor exiting.

In the reference frame assumed by the problem, in which we see the object moving, we consider uniform $\textbf{B}$ and no external electric field.

Thus, on each charge inside the conductor, a force $\textbf{F}=q \textbf{v}\times\textbf{B}$ is exerted. This force is perpendicular to both $\textbf{v}$ and $\textbf{B}$ and makes the charges inside the conductor separate.

This separation of charges let an electric field arise, which should stop the flow of charges, so no current is present.

Usually Faraday's law holds and the electromotive force is

$$\mathcal{E}=-\frac{\text{d}}{\text{d}t}\int_\Sigma \textbf{B}\cdot \text{d}\textbf{A}.$$

Since there is not varying flux (for any surface I consider) then $\mathcal{E}=0$.

But I expect an internal electrostatic field to be present.

Question 1: How can I formally prove this conservative electric field to be $\textbf{E}=-\textbf{v}\times\textbf{B}$ other than with dynamics arguments? How to deal with $\oint_{\partial\Sigma} (\textbf{E}+\textbf{v}\times \textbf{B})\cdot \text{d}\textbf{l}$? Is this (non-conservative) $\textbf{E}$ present (still with $\nabla\times \textbf{E}=-\frac{\partial \textbf{B}}{\partial t}=0$)?

When the two plates are connected with a resistor I should expect a current. But the emf seems to be still zero.

Question 2: Is this one of those Faraday's paradox cases?

Now, from the results, the book wants me to find $\sigma$ using the same formula of a plain capacitor with vacuum between the plates.

But only the conduction electrons would be shifted, every other charge would be stuck. This is not the case in which charges are (macroscopically) separated by vacuum.

Question 3: How could I use Gauss's law to get $E=\frac{\sigma}{\epsilon_0}$? Are the charges really uniformly distributed? Since the finite width $c$ of the plate, wouldn't the electrons be shifted towards the negative verse of the velocity (due to the arising component of the Lorentz force during their motion)?

Extra question: what would happen in the reference frame of the plate?

---

Edit: Just to emphasize the answers of what's bugging me. (@honeste_vivere pardon my insistence; if you suggest to move the edit as a separate question I will).

Is it correct to state the following?

• The net electric field inside the conductor is zero (in its rest frame). This is due to the field induced by the charges, which has opposite verse than the external one. Gauss' law is to be applied to the distribution of charges using the field induced by the charges. Moreover we can ignore the fact that this is not the case in which two metallic plates are separated by vacuum, but, instead, we have fixed metal ions and moving electrons (there is not a macroscopic separation between negative and positive charges).

• When I connect the two sides of the plate with a wire (of resistance R), as long as the wire is moving with the plate (not some fixed rails along which the plate is moving), nothing should happen after the capacitor has charged (i.e. the electrons have moved to one surface). Emf is still zero (integral form of Faraday's law) and no constant current is flowing. The exercise in the book is wrong assuming there is such current.

Answer 1

Update
I rephrased a few things and added some additional details after chatting with the OP.

Question 1: How can I formally prove this conservative electric field to be $\mathbf{E} = -\mathbf{v} \times \mathbf{B}$ other than with dynamics arguments?

The rest of this question isn't really necessary, so I only put in the first part. The electric field arises due to a Lorentz transformation, nothing more. The electric and magnetic fields transform according to: $$ \begin{align} \mathbf{E} & = \gamma \left( \mathbf{E}' - \frac{ \mathbf{V}_{o} }{ c } \times \mathbf{B}' \right) - \frac{ \gamma^{2} }{ \gamma + 1 } \frac{ \mathbf{V}_{o} }{ c } \left( \frac{ \mathbf{V}_{o} }{ c } \cdot \mathbf{E}' \right) \tag{0a} \\ \mathbf{B} & = \gamma \left( \mathbf{B}' + \frac{ \mathbf{V}_{o} }{ c } \times \mathbf{E}' \right) - \frac{ \gamma^{2} }{ \gamma + 1 } \frac{ \mathbf{V}_{o} }{ c } \left( \frac{ \mathbf{V}_{o} }{ c } \cdot \mathbf{B}' \right) \tag{0b} \end{align} $$ where $\mathbf{V}_{o}$ is the speed of the moving (primed) frame relative to a stationary one (unprimed) and $\gamma$ is the Lorentz factor.

If you look at Equation 0a for the case with $\mathbf{E}' = 0$, i.e., zero electric field in the sheet rest frame and you assume that $\mathbf{V}_{o}/c \ll 1$, then it reduces to $\mathbf{E} \approx -\tfrac{ \mathbf{V}_{o} }{ c } \times \mathbf{B}$. Therefore, in the lab frame where the sheet is moving, there will be an electric field orthogonal to outward normal of the sheet. The net result is very closely related to something called the Hall effect. Some of the electrons in the conductor will be able to move and will migrate one one side (electric field is into the page in your example, thus electrons will try to move toward the out-of-the-page side).

As you can see, you need not bring in Stokes theorem or anything else. It's just the consequence of a conductor moving in a stationary, uniform magnetic field resulting in an electric field due to a Lorentz transformation. That is, in the limit that $\mathbf{V}_{o}/c \rightarrow 0$ the electric field will disappear.

Question 2: Is this one of those Faraday's paradox cases?

No, nothing to do with that.

Now, from the results, the book wants me to find $\sigma$ using the same formula of a plain capacitor with vacuum between the plates. But only the conduction electrons would be shifted, every other charge would be stuck. This is not the case in which charges are (macroscopically) separated by vacuum.

I think you are over complicating this. You know what the electric field is that is experienced by each conducting sheet. Therefore, you can solve Gauss's law using the usual approach of drawing a pill box that goes part way into either of two conductors but not all the way. Therefore, there will be a net charge enclosed when this is done on either of two conductors. Note that the electric field must be the same as above, i.e., $\mathbf{E} \approx -\tfrac{ \mathbf{V}_{o} }{ c } \times \mathbf{B}$ (or $\mathbf{E} \approx -\mathbf{V}_{o} \times \mathbf{B}$ if you prefer SI units). That is, the induced charge is a result of the external electric field and they cannot generate more field outside the conductor, since that would violate energy conservation. Perhaps seen another way, the external field is inducing the charge on the conductor such that the conductor's electric field matches that of the external electric field. This happens because electric fields do work to get rid of themselves. You will notice that inside the conductor, the net electric field will be zero (e.g., think of Ohm's law in the limit of infinite conductivity). If we "turn on" the external electric field from a zero state, there would be a short period of time where electrons moved within the conductor in reaction to the external field but once static, the net field within the conductor would go to zero.

Question 3: How could I use Gauss's law to get $E = \tfrac{ \sigma }{ \varepsilon_{o} }$?

As I said above, treat each plate of the capacitor as an isolated thing and then do the usual pill box drawing etc. It is literally the same as a real capacitor hooked up to circuit (for the sake of this step in the solution/thought experiment).

Are the charges really uniformly distributed?

Yes, they must be and must be on the outer boundary of a conductor. As I said, electric fields do work to get rid of themselves. The charge distribution will appear as though one side of the plate is negative while the other is positive but the net electric field within the conductor will still be zero (in the limit of infinite conductivity).

Since the finite width $c$ of the plate, wouldn't the electrons be shifted towards the negative verse of the velocity (due to the arising component of the Lorentz force during their motion)?

Nope, the electrons move in the opposite direction to an electric field. Based on your geometry here, the electric field is directed into the page. Therefore, the electrons will move in the direction out of the page, i.e., the side facing the you viewing the plate here. The motion of the plate is irrelevant for the electrons in the absence of external electromagnetic fields. Acceleration could, temporarily, alter the electron distribution within the plate if the acceleration was incredibly strong but this is a whole other issue entirely.

Extra question: what would happen in the reference frame of the plate?

As you see from my above comments, the external electric field in the rest frame of the plate is zero, i.e., $\mathbf{E}' = 0$. However, the plate now has a polarization to it so it will have it's own electric field which will match the sign and magnitude of the original electric field, $\mathbf{E}$, for the reasons I stated above.

Now, from the results, the book wants me to find $\sigma$ using the same formula of a plain capacitor with vacuum between the plates.

If we suppose the two parallel plates are connected by a wire and resistor, then this would constitute a simple RC-circuit, where the external electric field would act analogous to there being a DC power supply as part of the circuit. Technically, I think this would act like a low-pass RC filter voltage divider, but similar principle. Note this is in the limit as the input power supply oscillation frequency goes to zero, i.e., static/stationary state. In this limit, the charge distribution is static and there's no discharge so long as the external field continues to exist and do work on the circuit.

To clarify, if we start the parallel plates (with attached circuit elements) at rest and start moving at $\mathbf{v}$ (ignore acceleration), the parallel plates will behave like a capacitor and charge up for some period of time related to the magnitude of the resistance in the circuit and the capacitance of the capacitor, i.e., the RC time. Once the plates are charged, the circuit will be static. Note that in this scenario, the motion through the field results in the experience of an external electric field, which is static. This can do work on the system just like a DC power supply (e.g., battery). The result is that the circuit is like an RC-circuit with a DC power supply in the static limit, i.e., long after the circuit started moving at $\mathbf{v}$. Then the resistor and capacitor will have potential drops across them, just like the equivalent circuit. So according to Ohm's law, there still must be a voltage drop, just like the equivalent circuit. In the limit of a perfect capacitor, the current can stop flowing (e.g., see answers to https://electronics.stackexchange.com/q/135632).

Answer 2

Lorentz's force is applied to electrical charges in movement, so the charges contained by the plane are moving with a velocity $v$. Due to the fact that $v$ and $B$ are perpendicular, the force $\vec{F} = q \vec{v} \times \vec{B}$ is pointing "to the left" for positive charges and "to the right" for negative ones.

For the second point think about the symmetry of the plane.

For the third point think that the electric field generated by Lorentz's force is $\vec{E} = \vec{F} /q = \vec{v} \times \vec{B}$ and the difference of potential can be seen as $V = Ec$ (the electric force between the "separated" charges equals the Lorentz's force when the system is in equilibrium).

Using your formula for the electromotive force doesn't make sense because the magnetic field $B$ and the geometry of the plane are constant, so no changes in the flux of $B$ (your assumption is correct but not useful in this case).

Now you should have all the knowledge to get the result given by your book.