What is the mathematical justification for the quadratic approximation to the energy of a spring in a one-dimensional lattice?

Question

It follows easily from this draw, the length $l$ of this spring as a function of the vertical distance $x$, as $l(x)=\sqrt{1+x^{2}}$

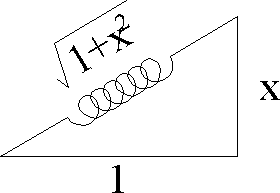
Now, $l$ can be expressed as a MacLaurin expansion:

$$l(x) = 1+\frac{1}{2}x^{2}+\frac{-1}{8}x^{4}+\frac{1}{16}x^6+...$$

Now, under the assumption that the natural length of the spring is equal to 1 (which happens for $x=0$) we can write the variation in the length of the spring as a function of $x$, provided that $x$ does small oscillations around $x=0$, as:

$$\Delta l = l - 1 \approx \frac{1}{2}x^{2}$$

There is no possible lower order approximation to $\Delta l$ (except saying that $\Delta l \approx 0$ which is of no use) because there is no first order term in the expansion. And so, I don't understand the justification that in the minute 46:30 of this lecture Prof. Susskind states that the longitude of a similarly arranged spring (belonging to a linear disposition of mass points connected by springs) is proportional to $x$ (he equivalently uses $q_{i+1} - q_{i}$ instead)

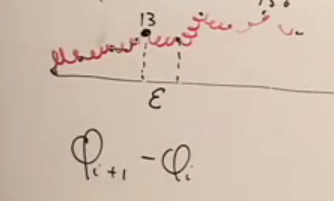
By the same reasons, I think that the potential energy of the spring $U = \frac{1}{2}k(\Delta l)^{2}$ for small oscillations of $x$ should be:

$$U = \frac{1}{2}k(\frac{1}{2}x^2)^{2}$$ $$=\frac{1}{8}kx^4$$

You may or may not like a four order term in the potential energy but as mentioned, because of the absence of a lower order term in the expansion above, it is either that or nothing at all.

But then, consequently with the statement of minute 46, the potential energy he derives (only vertical displacements are allowed) is quadratic and not of order 4

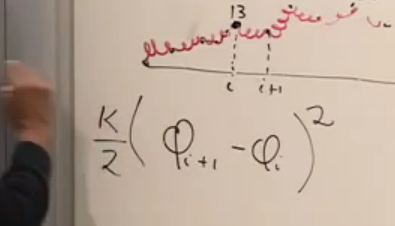
This is of course not a mistake of Prof. Susskind but a well-known approximation and quite elementary, bread and butter for condensed matter physicists. But, can anybody justify the validity of that quadratic approximation? (needless to say, trying an expansion of the polynomial $x^4$ gives as a result... $x^4$ itself)

I understand that such approximations always are (or at least can be justified as) introduced by neglecting terms in an expansion. But this one seems to me arbitrary.

Answer 1

Your answer is indeed what's going on in the lecture, but it doesn't explain what was wrong with your initial argument: you'd expect a model with $l_0>0$ to be a closer representation of reality than one with $l_0=0$, wouldn't you?

Actually, your initial reasoning was correct: transverse displacements of springs under zero tension do indeed result in fourth-order potentials. The harmonic potential is a trick used in the lecture to make the math work in a very simple model of a guitar string.

Normally, to get the equations of motion for a guitar string, one solves a second order differential equation for the transverse displacements. Such a derivation is given here, for example. In order to get the correct behaviour (without enforcing the harmonic potential) you need the $y(0)=y(l)=0$ boundary condition, and tension $T$ (i.e. $\Delta l \neq 0$).

A guitar string needs to be tensioned, with both ends fixed, in order to work.

Answer 2

Consider the first three terms of the Taylor expansion of the potential. The first term is a constant, which will not affect the motion. The next term is the linear term, which will only change the equilibrium point. So the first interesting term is the quadratic term.

Answer 3

Interestingly, the quadratic potential can be justified by considering that the springs have natural length zero, i.e. $l_{0}=0$ and not $1$ (this means $l=1$ for $x=0$ just the same, but the spring is not relaxed and the potential energy will have a different dependency). I understand that this is silently assumed behind the explanation in the 1h15min mark. Translating to my draw and notation, $\Delta l = l $ and not $l-1$ as before. Thus

$$\Delta l = l = \sqrt{1+x^2}$$

and then the potential energy is

$$U=\frac{1}{2}k(\Delta l)^2=\frac{1}{2}k(\sqrt{1+x^{2}})^2 = \frac{1}{2}k+\frac{1}{2}kx^{2}$$

The term $\frac{1}{2}k$ is a constant and therefore it is ignored because it is going to disappear anyway when we plug the Lagrangian into the Euler-Lagrange equations with its derivatives. That's all.

Another way to see it is that, when $l_0=0$ and not $1$, a MacLaurin expansion can be used for $\Delta l(x)$ (although in this case it is not needed) and the zero order term (the $1$) does not disappear, giving rise to the desired quadratic term when plugged into the potential energy expression:

$$l(x) = 1+\frac{1}{2}x^{2}+\frac{-1}{8}x^{4}+\frac{1}{16}x^6+...$$

$$\Delta l = l - 0 \approx 1+\frac{1}{2}x^{2}$$ (and NOT $\Delta l \approx \frac{1}{2}x^{2}$ as before; that additional $1$ makes all the difference!)

$$U=\frac{1}{2}k(\Delta l)^2=\frac{1}{2}k(1+\frac{1}{2}x^{2})^{2}$$ $$=\frac{1}{2}k(1+x^{2}+\frac{1}{4}x^{4})\approx \frac{1}{2}k(1+x^{2})$$ $$=\frac{1}{2}k + \frac{1}{2}kx^{2}$$ There it is.

Summary

The quadratic potential arises naturally as a consequence of using springs with zero natural length in the model. This is not possible in the case of natural length equal to the horizontal distance between the point masses.

Final comment: a guitar string has a strong tension, even when completely horizontal. This suggest that the model with $l_{0}=1$ (that would yield zero tension when the string is horizontal) is unrealistic. The model with $l_0=0$ is a better approximation to a tensed string.

Answer 4

I see this post is old but I hope I can help those who read it in the future:

In the string model that (I assume) is being explained, one is only interested in the vertical component of the spring's force (it is easy to see that the horizontal one must be, at least on average, always zero), which is obtained by multiplying the net force times the sine of the angle formed between the spring and the horizontal. Thus, we are bot searching for $F(x)=k\sqrt{1+x^2}$, but for $F_{vertical}(x)=k\sqrt{1+x^2}\frac{x}{\sqrt{1+x^2}}=kx$. Because the potential of a spring is quadratic it can be neatly decomposed into various identical springs, one in each cartesian direction, with no troublesime crossed terms, so each direction behaves independently, and therefore, linearly.