Are fundamental forces net forces being effective in straight lines?

Question

Considering electrostatic force,a part of electromagnetic force,

When a positive point charge (fixed) and a free negative point charge are in surroundings,we observe an attraction between them. Also,the force of attraction is along the line joining the two charges.

Just for an imagination,

I thought that there can be forces ,not along the line joining the two charges, which can also contribute to attraction force. I also considered those forces to be symmetric. Seeing the net effect, it also created an attractive force in the direction of line joining both the charges.

This arose me a question, Is electrostatic force (similar for gravitational force too) between charges just a single force or a net force? How can we say a fundamental force is a net force or not?

This can also be rephrased as, Is electrostatic force between two charges is along the line joining the two charges or just we are perceiving so as a net force?

EDIT

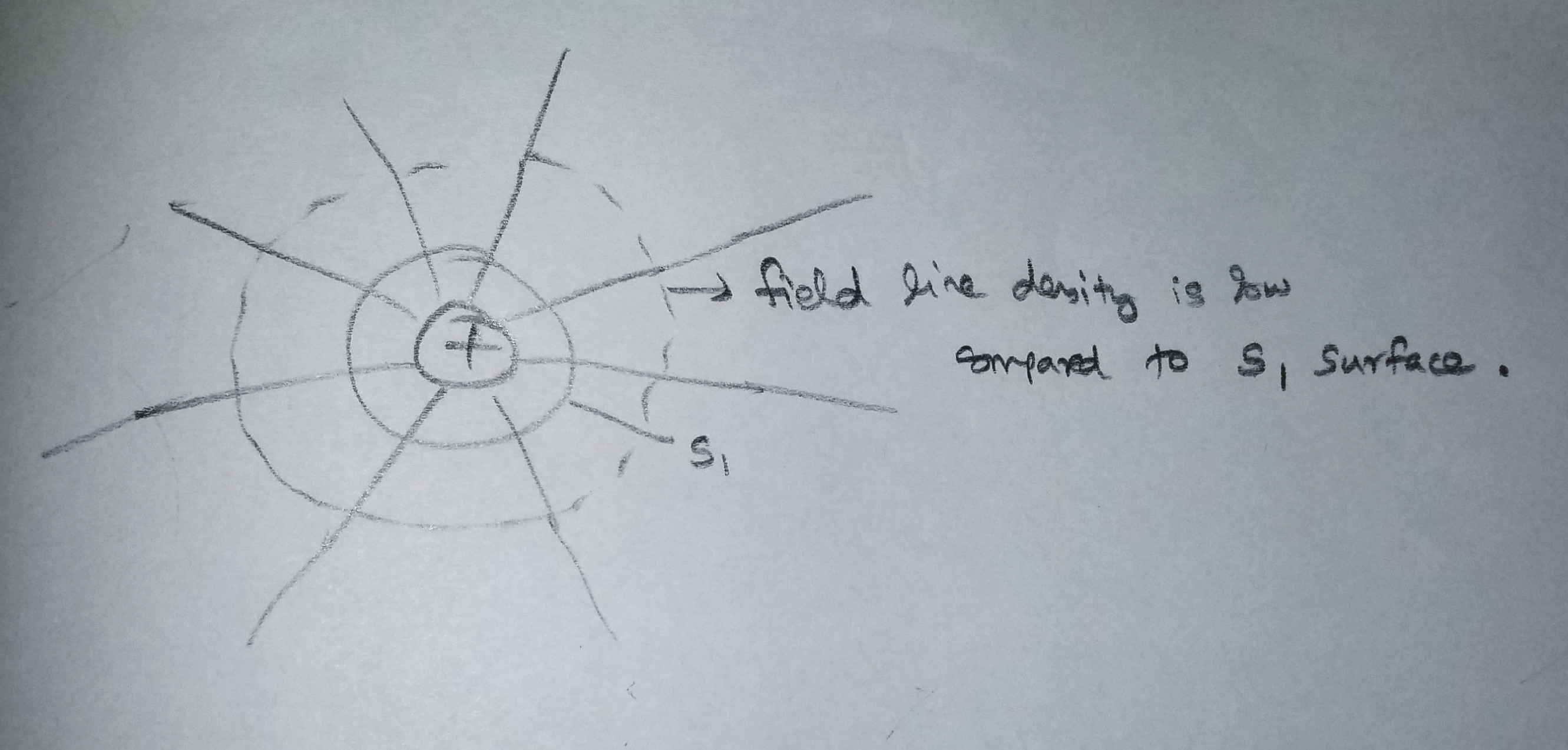
To explain electrostatic force we developed the idea of electric field and field lines. So, F=Eq. When we draw field lines around a positive charge, the density of lines gives us an idea of electric field intensity around the charge.

Electrostatic force is related to field intensity. Also, field intensity at a point is represented by density of field lines. Density depends on surroundings. This gives me an idea that, force at any point depends on surrounding (supporting my question , "is force just along the line joining the two charges?" ) , as field intensity depends on density, which needs the idea of surrounding region. I know the idea of density of lines related to field intensity is just from pictorically representing field lines. But I feel that this idea can support my question, but not sure.

Answer 1

The electrostatic force or the electric field (force per unit of charge) is the force the charges feel. It's not the net force of many forces, since it's the only force.

A force field is a function from the space variables (points in space) which returns the force (in the form of a vector) at that point. The other points in space, where the charge isn't, aren't feeling any "force", nor those "forces" aren't having any effect in the charge. The field is just a construction for representing the (net, if there is more than 1 charge) electric force at each point.

Answer 2

Modern QM and GR clearly showed us the material world is ontologically nothing but metrical field bundled by its affine connection field, thus all other physical quantities can be reduced to some illusory phenomena like force, even spacetime can be better viewed as an external quotient relation between the above said bundled fields which are the only really existed substance. From this top-down view the classical electrostatic force you were concerned with can be always viewed as a net phenomenon like moving in a potential well, so strictly speaking it's not a "net force" but a "net effect" perceived as a "single force", and neither "net force" nor "single force" is real ontology.

Answer 3

The force between two point charges IS defined as a single force acting along the line joining them , but that between two distributions of point charges or one distribution and one point charge is a Net force according to the superposition principle. Because we can not break the smallest force between two point charges to a sum of other elementary forces , since we already assumed that it is a point charge and that its dimensions are very small, at least in theory. I hope I answered your question.

Answer 4

Thinking about it again, your interpretation is actually a little interesting as an intuitive explanatory picture. It is a (not often discussed, as far as I know) corollary of the shell theorem that results when you combine it with Newton's laws, particularly the third law.

Newton's third law says that every force has an equal reaction force. Thus, when we integrate over a spherically symmetric shell as in the shell theorem, we can similarly be integrating over this reaction force (i.e. the force on that point of the shell due to the external charge). We're able to translate these reaction forces to the center of the sphere, because:

1. Each force has partner on the other side of the axis that connects the sphere's center to the external particle, so we can translate these forces to that axis without changing the torque on the rigid spherical body.
2. Once the forces are on the central axis, and cancel with their partner, it is clear they do not torque the axis of symmetry, so we can translate them across the axis to the center of the sphere.

These forces will add in superposition, and the result, by Newton's third law, is that the force on the shell equals the Coulombic force imparted on the external charge, but in the opposite direction, exactly as if the shell were a point charge located at the center.

The important thing is that the shell isn't made to spin, or anything. (Although that is a simple consequence of $\nabla\times\mathbf{E}=0$.)

Once you add a bunch of external charges outside, this simple view still holds in superposition.

So this shows that you can think about the classical electrostatic force as a net force, as long as that force acts on a spherically symmetric charge distribution with the same total charge as the point charge, and all external charges are outside the radius of that ball.

Intuitive Explanation

Let's look back at your picture, and the above connects with an intuitive picture of the field lines.

First, you blow up the point charge to a finite size (as discussed above), so you can see the difference in electric field line densities across its surface. (Although it's not too important, note that inside, the field will appear as how things would be if the point charge was not there in the first place.)

Because each field line starts at a positive charge, and ends at a negative charge, we can imagine each field line as a "tug" on the charged particle. (For negative charges, the tug on it is in the opposite direction as the arrow on field line.)

For a charge in free space those field lines go out to infinity, and they are tugged equally in all directions. (Hence, not at all.)

When a positive and a negative charge approach each other, the field lines deflect toward one another, and the net tug on each particle is attractive.

When charges of equal sign approach each other, the field lines deflect away from each other, and the net tug on each particle is repulsive.

As long as you still draw the electric field lines such that they follow Maxwell's equations (i.e. get the divergence right, have no curl), I am fairly confident this intuitive picture should hold in the electrostatic case for point charges.

I still think it's easier just to stick to the Coulombic force, but your idea can lead to a nice picture, as I've tried to (non-rigorously) show.

(Note: I cleaned up this answer in an edit, adding the "proof sketch" at the beginning.)

Answer 5

I think, in a classical context, the confusion occurs in the first drawing of your second photograph. The field depicted is due to both charges and that field represents the direction of the electrostatic forces which a third charge experiences. For each of both individual charges, the field lines emanating from the individual charges (so the field lines which are there in the presence of only one electric charge) determine how the other charge will move. Each separate charge, when considered a point particle, will not experience the infinity of symmetric forces you depict if the density of field lines goes wild. This would yield an infinite force. Of course, if the charges have a spatial extension, as in your drawing, the charges are influenced by more than one field line. In fact by an infinite number of them. In the case of the two charged blobs you depicted one has to integrate over the charge densities to find the force between the blobs.
The density of field lines is in fact ill-defined. In every volume of space, there is an infinity of field lines. So its quite useless to state that the nearer a charge is approached, the "more infinite" the field line density grows. Electric flux, on the contrary, is well defined and its density at various points is related to the electric field.
The pattern of field lines in your first drawing on the second photograph is the pattern a third charge encounters when moving relative to these two. The two charged particles themselves don't follow these field lines (without a third charge in play), just as a single charge doesn't move in the field lines produced by itself (even though the sum of all forces ending "on" the charge is zero in this case).
The infinity of different field lines at the point where each charge finds itself doesn't act on the charge producing these field lines. Moreover, this infinity doesn't actually exist at the point where the charge finds itself. There is a shell (with a radius that approaches zero) around the charge on which the flux density is infinite (infinite electrostatic force). The field lines don't intersect, as would be the case if the lines end up at the charge (only one field line can exist at every point in space).
So the field produced by two charges tells you how a third charge will move. And just so, the field of one point-charge tells you how a second charge will move in it (and vice-versa). So don't confuse the field produced by two charges with the fields in which the two charges move (which are one-charge-fields).
So the point charges don't experience symmetric forces in the way you depicted as this would yield an infinite force towards the other charge after summing them all. A finite result is only obtained if all forces (or the charge itself) approach zero, which isn't the case.

Of course, I can misunderstand what you mean. It could be that you mean to say that there is a finite amount of symmetric forces, leading to a finite net force, but then obvious new problems arise. It might also be that you mean that there is an infinite amount of forces that have all a magnitude that approaches zero, leading to a finite net force (see the answer involving tiny threads of elastic "material").

Answer 6

To explain this question, I will use classical mechanics and deflection of a beam of positively charged particles from a nucleus by aiming the positively charged particles a distance above the nucleus.

In a simple experiment, as the velocity of a positively charged particle increases, the particles deflected will cover a smaller range as they are deflected.

The range is complete when the horizontal velocity is equal to zero. The force that slows the particles down in the horizontal direction is the repulsive force along the horizontal direction. As the objects move a unit distance x in the horizontal direction, their velocity decreases from the work-energy theorem. The time it takes for the objects to take the distance x, is therefore important because the Range is modeled as the time it takes for the horizontal velocity to reach zero and the average speed in the horizontal direction.

On the other hand, as the velocity decreases in the horizontal direction, the vertical component increases from zero. In the end, the object only has vertical velocity.

if we consider both of them point charges, the net force acting on the particles is modeled by $kQq/((rx)^2+(ry)^2)^{1/2}$, and the x and y components are constantly changing.

Answer 7

I think a lot of the answers above mine are very insightful when viewing the problem from the perspective of classical field theory. However, I think when we apply the principles of quantum field theory (QFT), as we should to fundamentally understand the electromagnetic interaction, it becomes more accurate to describe the force caused by a field as a net force as you proposed.$$$$"Forces" do not per se exist in QFT, but we do consider particles acting under the influence of fields. These particles will take a trajectory given by Feynman's sum-over-histories integral: $$G=\int{D[q(t)]e^{\frac{iS}{\hbar}}}$$ Where G is the "propagator" which essentially moves the particle from one point to another.$$$$This is a nasty looking integral, but the conceptional impact of it is that we imagine a particle taking every possible path from spacetime point $(x_0,y_0,z_0,t_0)$ to point $(x,y,z,t)$ and we sum up all of these different paths weighted by their action $S$ such that: $$S=\int{Ld^4x^\mu}$$ The long and short of this is that a particle moving in a field characterized by the potential energy component of $L$ will take every single path possible to create a propagator which will result in the most likely path of propagation being the one with the least action, e.g. the straight line between two point-like charges. So while I cannot, in good conscience, speak to force, I can say that the sum of all possible paths a particle might take, characterized by their Lagrangians including potential energy, is required to propagate between two points, so I think by analog it is more correct to say that a fundamental force is a net force.

Answer 8

All the other answers seem to focus on the force aspect of this problem. Let me try to address your question in a slightly different way.

Electrodynamics is distinct from classical mechanics in many ways, one of which being that it is a field theory: the (electric) field here is the fundamental object. Indeed, the forces on charged objects should be thought of as resulting from those objects' interactions with the field, given by Coulomb's law, $$\mathbf{F} = q\mathbf{E} + q\mathbf{v}\times \mathbf{B}.$$

A nice way to think about your setup with two point particles is this. Every charged particle interacts with the electromagnetic field in its immediate vicinity, whether that field is generated by parallel plates, another particle, or other external currents. When you fix a positive charge in space, it generates an electric field $$\mathbf{E} = \frac{q\hat{\mathbf{r}}}{4\pi\epsilon_0 r^2},$$ which permeates all space (except for the location of the charge, a subtlety we currently ignore). We know that charged particles interact with any electromagnetic field, so a negatively-charged object, sensing this field in its vicinity, will be pulled along the field lines towards the positive charge. Notice that so far, the electric field seenby the negative charge is radially outwards and spherically-symmetric.

Do not confound this with the dipole electric field, which has those curved field lines you drew. That field is what a third charged particle will see as it moves in the vicinity of the two particles. Indeed, the dipole field you drew is precisely the superposition of two spherically-symmetric electric fields, and so the force exerted on the third particle can be thought of as a vector sum of the forces exerted on the third particle by the other charges.