Each thermodynamic potential (Enthalpy, Helmholtz free energy, Gibbs free energy) is the same as the 1st law of thermodynamics. Then, why do we need them? Why did people introduce them in the first place?

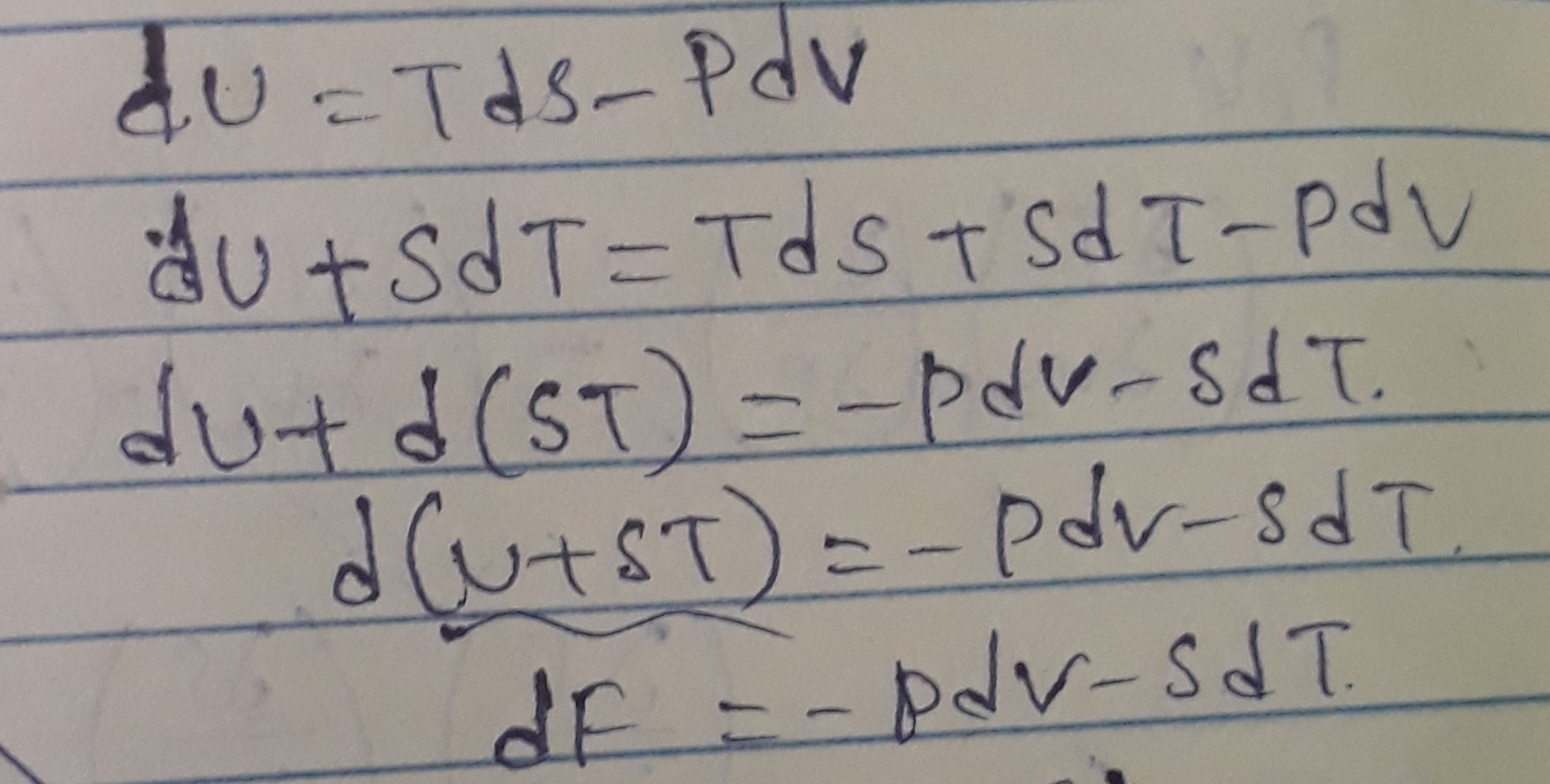
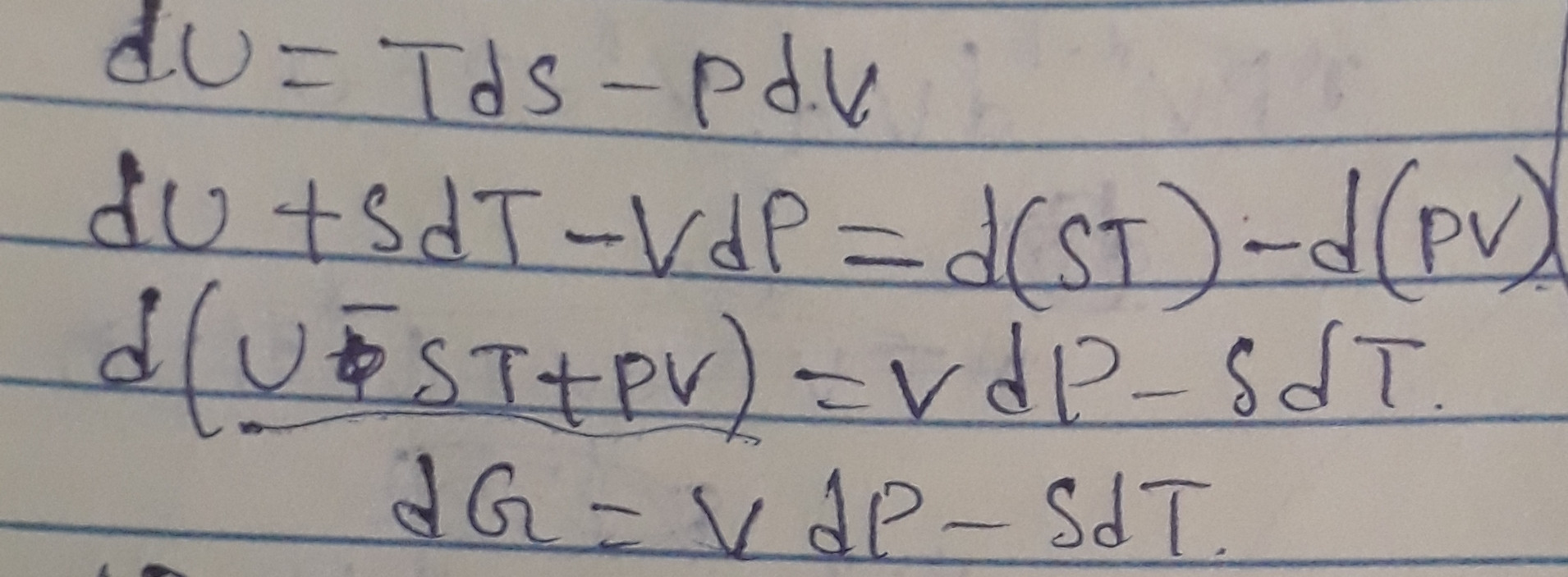
-
$\begingroup$ $dU=TdS-pdV$ is not the first law of thermodynamics. $\endgroup$– jacob1729Commented Jan 26, 2021 at 13:43
4 Answers
These potentials are introduced primarily for their usefulness and the corresponding insight which they bring. Of course one can do the whole of thermodynamics without ever giving a name or a symbol to things like $U-TS$ and $U+pV$ and $U+pV-TS$, but in practice it is useful to draw attention to them.
The potentials $F$, $H$ and $G$ behave somewhat like potential energy and somewhat like negative entropy.
$F$ is useful when considering things like mechanical work for a system maintained at constant temperature. This is because $$ dF = - S dT - p dV $$ so if $T$ is constant then the change in $F$ is equal to the work done if the work is done in a reversible manner.
$H$ is useful for things like flow processes and chemical reactions where the conditions often involve fixed pressure. $G$ is also useful for chemical reactions, and for the consideration of phase changes.
For an isolated system, the equilibrium state is the one which maximises the entropy. For a system in contact with a thermal reservoir at some given temperature, the equilibrium state is NOT the one which maximises the entropy of the system alone; it is rather the one which maximises the entropy of system and reservoir together, and, as it turns out, this is the one which minimizes the free energy $F$ of the system alone! This is a remarkable observation, and it opens up a road for understanding a system whose temperature is fixed. For such a system, $F$ is offering us a way to go straight to the heart of the problem, because it is the function which takes an extreme value (here a minimum value) in thermal equilibrium, and it is a minimum with respect to all possible physical changes in the system as long as the latter remains in contact with the fixed-$T$ reservoir. This is the sense in which $F$ acts as an entropy-like as well as an energy-like function: the equilibrium state, in a certain commonly-realised situation, is the one where $F$ takes a stationary value with respect to internal changes in the system, and those internal changes could be any sort of change at all, including chemical reactions, electrical currents, mechanical effects, living cells, etc..
The other thermodynamic potentials behave in a corresponding way under various other conditions (thermal reservoir, pressure reservoir, or both).
Each potential describes a different physical situation, i.e. they differ by the controlled variable of the experiment being described by them. Take for example the Helmholtz free energy. Note that $$ dF=-PdV-SdT $$ so F is a function of volume and temperature: $F(T,V)$, since it follows from the equation that small variations in V or T cause a variation in F. It therefore describes a situation in which these variables are controlled, while their conjugate variables depend on them. An experiment connected to a heat bath and confined to a constant volume can be described by this potential. A different experiment, e.g. when pressure is constant (and volume may vary), will be described by the Gibbs free energy, and so on.
-
$\begingroup$ Why is volume constant, when the system is described by helmholtz free energy. $\endgroup$ Commented Jan 26, 2021 at 8:06
-
$\begingroup$ From the differential form of F (dF), we identify the thermodynamic variables that it depends on (see Differentials in several variables) are V and T. This means that one can plug in a value of temperature and volume and get a value of F, so it is defined when V, T are defined. However, if for example P is kept constant, the volume may vary. In this case, which value of V will you plug into F(T,V)? $\endgroup$– occd2000Commented Jan 26, 2021 at 8:15
Two central questions in thermodynamics are
- What are the conditions for spontaneous reaction?
- What are the conditions for equilibrium?
Constrained by First law of thermodynamics (Conservation of Energy), and hinted by second law of thermodynamics (Increasing entropy), under different conditions, these questions are answered by different types of thermodynamic potentials.
Consider any dynamic reaction, for generalised constant externally applied force and displacement, the work input to the system is $\text{đ}$W $\leq$J$\cdot$ dx, also from second law $\text{đ}$Q $\leq$ TdS; now use first law dU=$\text{đ}$Q+$\text{đ}$W $\leq$ TdS +J$\cdot$dx
Rearragement gives: dU-TdS-JdX $\leq$0. This inequality indicate when system is not in equilibrium, the quantity U-TS-JX tends to minimise, that's the answer for spontaneous reaction, ie: when the quantity is not minimal, spontaneous reaction take place to minimise this quantity. Similarly, for equilibrium, once that quantity is minimise, equilibrium is achieved. This quantity is called availability in thermal physics.
Return to the question why people introduce various thermodynamic potentials, actually these thermodynamic potentials are special case of availability.
For instance Helmholtz free energy, TdS $\geq$ dQ Substitution gives: dU=TdS-pdV= $\text{đ}$ Q+ $\text{đ}$W Rearragement gives dU+pdV=TdS $\geq$ dQ For $dT=0$ and $dV=0$, this gives: d(U-TS)=dF $\leq$ 0
Which means for a system with fixed volume at constant temperature, the condition for equilibrium is to minimise Helmholtz free energy.
The potential F and G depend not only on the first law of thermodynamics, but also the second law of thermodynamics (since they contain the entropy S). You are correct that all the results of interest in thermodynamics can be expressed without making use of these potentials. But these potentials are very convenient to use in a huge number of situations, and the results of interest are expressed much more concisely in terms of these potentials.
