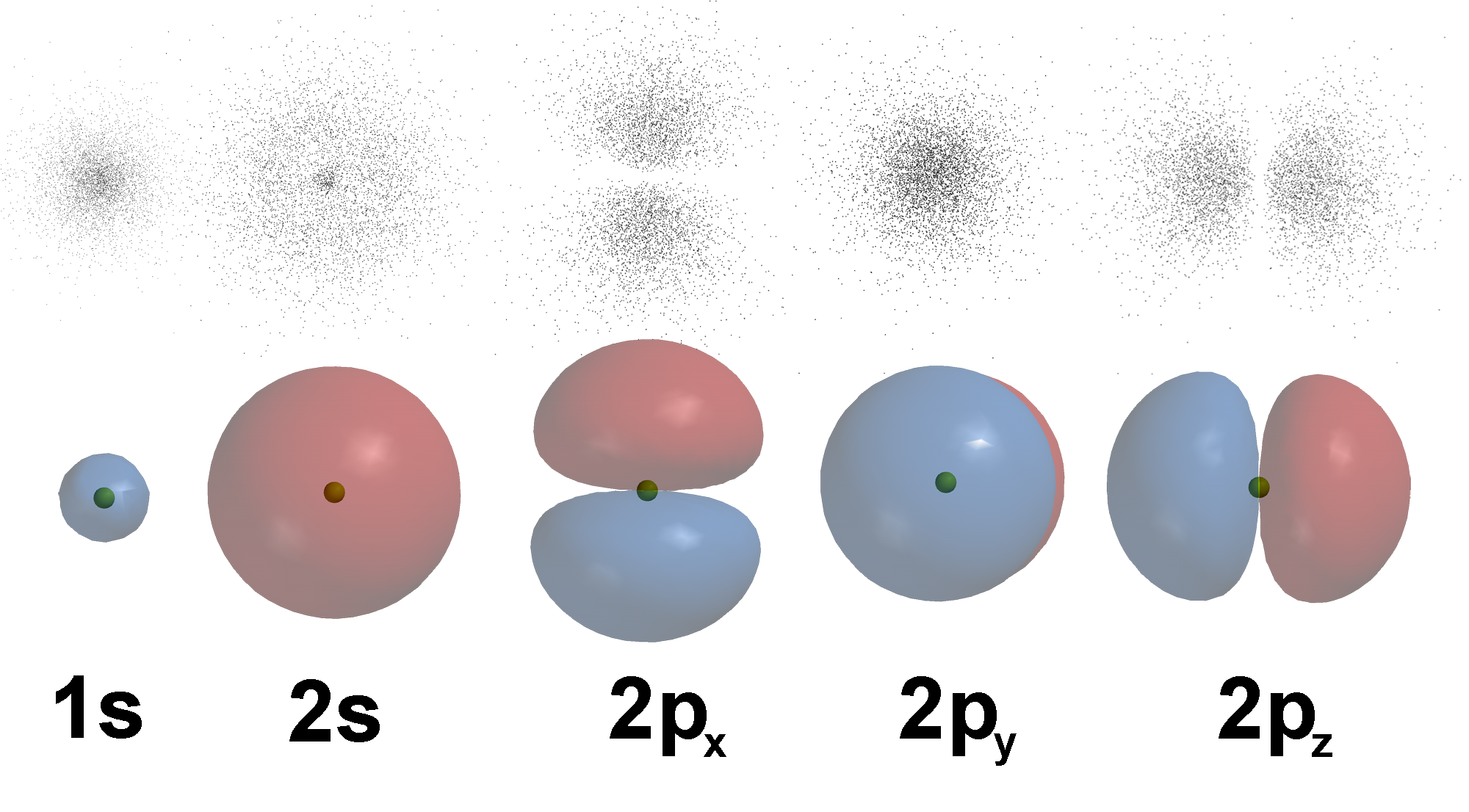
It's important to note the atomic orbitals are approximations. In the context of the basic hydrogen atom Schrödinger Equation, they are exact eigenstates of energy, total angular momentum squared, and $L_z$, where $z$ points in any direction you want it to.
As energy eigenstates, they are stationary states, and their time evolution involves a global phase rotating with frequency $E/\hbar$. As such, they can never change, which obviously contradicts experiment. Call this "problem 1".
Also: in quantum mechanics, the electron is a point particle. This leads to problematic interpretations that have their uses, but are not fundamental. One of these interpretations is that electron moves randomly in a fashion that has it inside an orbital boundary 90% of the time. Call this "problem 2".
Both these problems are addressed in quantum field theory, in which the electron is no longer a point particle, but the minimum excitation of the electron field, a spinor field that fills all space. With that, an orbital describes how the electron field excitation of a single electron is spread out over space in an approximate energy eigenstate, and how it propagates in time.
The wave function then represents the complex quantum amplitude, whose modulus squared is the probability density of the electron's location. There really is no intuitive (or classical) way to understand coherent complex amplitudes of fermion fields, other than it's kind of like how we treat light...but with conserved quantum numbers, antiparticles, and Fermi-Dirac statistics.
The quantum field treatment also applies to the electromagnetic field, which then adds an interaction term to the hamiltonian, and allows transitions between states. It also adds virtual electron positron pairs to the binding, and that's only at the 1st order. The actual complexity of the state is beyond calculation.
With that, I would say the wave function is a mathematical approximation to something physical. I do believe this conundrum is the origins of Feynman's two famous quotes on quantum mechanics:
The disheartening,
" I think I can safely say that nobody understands quantum mechanics."
and the practical,
"Shut-up and calculate"